If f(x) = (x - a)(x - b)(x - c), show that f'(x)/f(x) = 1/(x - a) + 1/(x - b) + 1/(x - c)

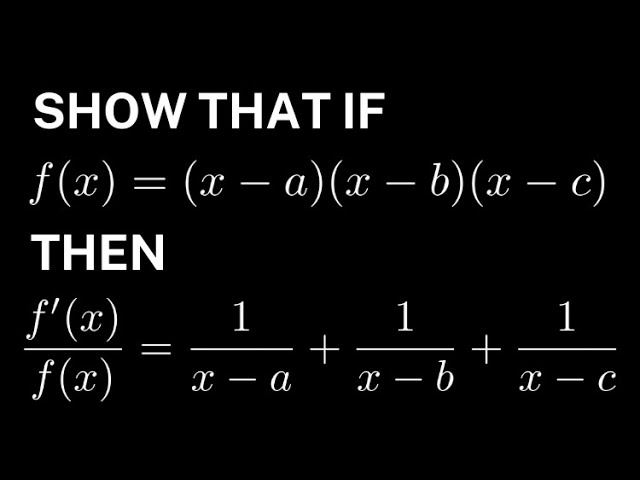
Показать описание

This is a Calculus problem where we do a simple proof. To do this problem we start by taking the natural logarithm of the function f(x), and then we differentiate.

If you enjoyed this video please consider liking, sharing, and subscribing.

There are several ways that you can help support my channel:)

************Udemy Courses(Please Use These Links If You Sign Up!)*************
Abstract Algebra Course

Advanced Calculus Course

Calculus 1 Course

Calculus 2 Course

Calculus 3 Course

Calculus 1 Lectures with Assignments and a Final Exam

Calculus Integration Insanity

Differential Equations Course

Differential Equations Lectures Course (Includes Assignments + Final Exam)

College Algebra Course

How to Write Proofs with Sets Course

How to Write Proofs with Functions Course

Trigonometry 1 Course

Trigonometry 2 Course

Statistics with StatCrunch Course

Math Graduate Programs, Applying, Advice, Motivation

Daily Devotionals for Motivation with The Math Sorcerer

Thank you:)

Рекомендации по теме

Комментарии

There is something beautiful about thinking of the derivative of a polynomial expressed via it's roots as being equal to that function distributed over the sum of the reciprocals of its roots. Also, this problem made me consider the generalization of the product and quotient rule to more than two functions, which surprisingly I hadn't encountered before

Penrose

Nice, I just differentiated the function with the product rule and divided, then the terms all cancel out XD

johnchristian

sir that's smart omg I just brute force it it was pretty easy you should just know what is the derivative of the multiplication of 3 functions are

medaliboulaamail

Ok I want to do this the stupid way, and see what happens; so here goes:

Given:
f(x) = (x – a)·(x – b)·(x – c)
To show:
f'/f = 1/(x – a) + 1/(x – b) + 1/(x – c)

Differentiating both sides:
f' = (x – b)·(x – c)·1 + (x – a)·((x – b)·1 + (x – c)·1)
= (x – b)·(x – c) + (x – a)·(x – b) + (x – a)·(x – c)

Dividing by f:
f'/f =
((x – b)·(x – c) + (x – a)·(x – b) + (x – a)·(x – c))/(x – a)·(x – b)·(x – c)

Separating terms and dividing through:
f'/f = 1/(x – a) + 1/(x – b) + 1/(x – c) proved.

Ok this was much easier than I thought it would be.

GirishManjunathMusic

I've followed your advice and I read the Ian stewart "calculus". Right now I saw this video and I've understood every passage. Great satisfaction. Thanks!

emanuelamorandini

There’s a very quick jaunt from here to the definition of the Digamma function from the Weirstrass form of Gamma

yugiohsc

Sir this question is similar as class 12 Ncert MATHS part 1
Chapter continuity and differentiability

wpmrszy

I didnt even think about that solution, I tried to execute the whole polynomial product but it became so exhausting 😂

bilalhadrous

If f(x) = (x - a)(x - b)(x - c), show that f'(x)/f(x) = 1/(x - a) + 1/(x - b) + 1/(x - c)

If f(x +1/x) = x - 1/x , find f(x)

If f(x-3)=4x+5, then find f(5) and f(x).

Determine if f(x) = x + 1/x is an Even or Odd Function

Find a and b if f(x) is continuous everywhere

If f(x-1)=2x+3, what is f(x)

If f(2/x) = 3x + 5 find f(x) and f(2)

If f(x + 1) = 3x + 11, find f(x) and f(-1)

Finding f(0) When f(f(x))=x^2-x+1

If f(x-1)= 2x-5, find f(-3) and find f(x+1)

If f(x) = ax + b, f(-1) = -1, f(3) = 1 find the values of a and b and then find f(x)

Find f(g(x)) and g(f(x)) and Determine if the Functions f and g are Inverses

If f(2x-1/3x+2) = 3x - 2 find f(x)

If f and g are the functions whose graphs are shown

If f(x)=2x+3 & f-¹(x)=1,find x. algebra | inverse functions

If f is a function satisfying f(x + y) = f(x) f(y) ∀ x, y ∈ N such that f (1) 3 and ∑f(x) for…...

Find f(x) if f^-1(x)=1/x-2

Check if f(x)=4x+3 is invertible then find inverse of f

Learn how to evaluate for a function

Prove that if f(x) = log(x/(x - 1)) then f(y + 1) + f(y) = log((y + 1)/(y - 1))

Given f(x)=x^3-ax^2+bx+1 If f(2)=-3 and f(-1)=0 Find values of| Functions | Values | Pythagoras Math

Find f(1), f’(1) for f(x) = x^5 + 2x^3 + x -1, find f^(-1) (3), f^(-1)’(3). Derivatives inverse...

(fog)(x) and (gof)(x): How to find them, given f(x) and g(x).

f(x+y) = f(x)f(y)

f(x) bigger than 0 | Grade 11 Functions