filmov
tv
Mathematics Colloquium: Algebraic Graph Theory and Quantum Physics | Chris Godsil

Показать описание
Online Mathematics colloquium by Professor Chris Godsil (University of Waterloo), held on 1 July 2021.
Abstract: Somewhat surprisingly, work in quantum physics leads to interesting questions in algebraic graph theory. This connection goes a long way back, but has become much stronger because of recent developments in quantum information theory. I will discuss some particular cases: Quantum walks, Geometry of lines, and Colouring problems.
Bio: Chris Godsil is Distinguished Professor Emeritus at the University of Waterloo. Chris is a research leader in discrete mathematics and has been (and continues to be) hugely influential in many different fields of mathematics around the world. Recently, Chris has been a pioneer in using techniques from algebraic combinatorics to tackle some fundamental questions in quantum information theory.
He has served as a member of the Research Committee of the Canadian Mathematics Society, on the Speaker Selection Committee for the Combinatorics Session of the 1998 International Mathematics Congress and on the organizing committee for the SIAM Conference on Discrete Mathematics (San Diego, August 2002) and for the second Canadam Conference (Montreal, May 2009). He is a cofounder of the Journal of Algebraic Combinatorics and serves on the editorial board of the Australasian Journal of Combinatorics, the Journal of Combinatorial Theory Series B, and Combinatorica. His extensive contributions to mathematical research include three very successful books, Algebraic Combinatorics, Algebraic Graph Theory (co-authored with Gordon Royle), The Erdős-Ko-Rado Theorem: Algebraic Approaches (co-authored with Karen Meagher), and over 120 papers in research journals. He has supervised over 30 graduate students.
Abstract: Somewhat surprisingly, work in quantum physics leads to interesting questions in algebraic graph theory. This connection goes a long way back, but has become much stronger because of recent developments in quantum information theory. I will discuss some particular cases: Quantum walks, Geometry of lines, and Colouring problems.
Bio: Chris Godsil is Distinguished Professor Emeritus at the University of Waterloo. Chris is a research leader in discrete mathematics and has been (and continues to be) hugely influential in many different fields of mathematics around the world. Recently, Chris has been a pioneer in using techniques from algebraic combinatorics to tackle some fundamental questions in quantum information theory.
He has served as a member of the Research Committee of the Canadian Mathematics Society, on the Speaker Selection Committee for the Combinatorics Session of the 1998 International Mathematics Congress and on the organizing committee for the SIAM Conference on Discrete Mathematics (San Diego, August 2002) and for the second Canadam Conference (Montreal, May 2009). He is a cofounder of the Journal of Algebraic Combinatorics and serves on the editorial board of the Australasian Journal of Combinatorics, the Journal of Combinatorial Theory Series B, and Combinatorica. His extensive contributions to mathematical research include three very successful books, Algebraic Combinatorics, Algebraic Graph Theory (co-authored with Gordon Royle), The Erdős-Ko-Rado Theorem: Algebraic Approaches (co-authored with Karen Meagher), and over 120 papers in research journals. He has supervised over 30 graduate students.
 0:59:23
0:59:23
 0:52:41
0:52:41
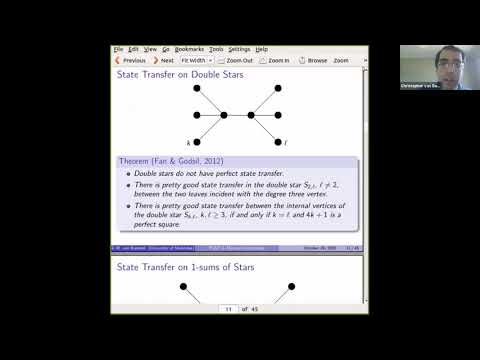 0:47:24
0:47:24
 0:59:39
0:59:39
 0:53:00
0:53:00
 0:42:25
0:42:25
 0:56:16
0:56:16
 0:49:27
0:49:27
 1:15:31
1:15:31
 0:45:30
0:45:30
 0:49:34
0:49:34
 0:55:03
0:55:03
 0:59:45
0:59:45
 1:08:56
1:08:56
 1:01:35
1:01:35
 0:53:28
0:53:28
 0:56:06
0:56:06
 0:55:28
0:55:28
 0:48:07
0:48:07
 0:51:23
0:51:23
 0:37:34
0:37:34
 0:00:15
0:00:15
 0:16:26
0:16:26
 0:54:39
0:54:39