Algebraic Graph Theory: Finite edge-transitive Cayley graphs, quotient graphs and Frattini groups

Talk by Cheryl E Praeger.

Joint work with Behnam Khosravi, Institute of Advanced Studies in Basic Sciences, Zanjan, Iran

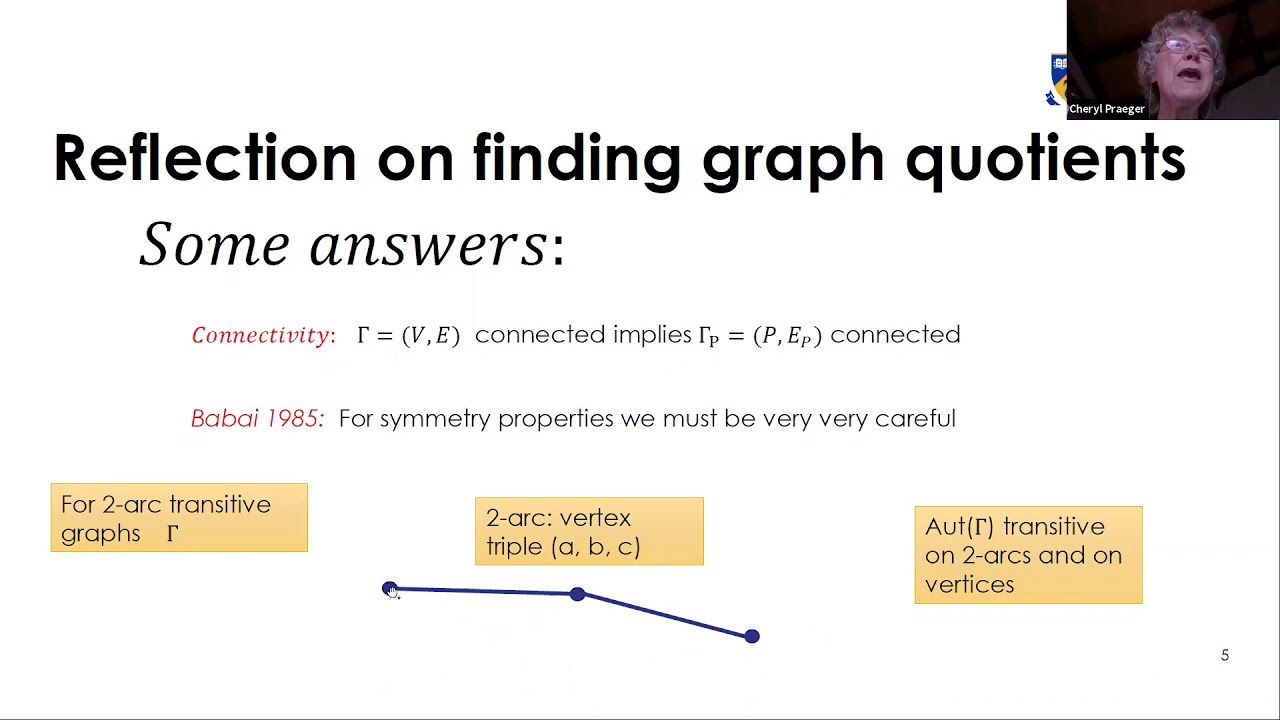
The edge-transitivity of a Cayley graph is most easily recognisable if the subgroup of “affine maps” preserving the graph structure is itself edge-transitive. These are the so-called normal edge-transitive Cayley graphs. Each of them determines a set of quotients which are themselves normal edge-transitive Cayley graphs and are built from a very restricted family of groups (direct products of simple groups). We address the questions: how much information about the original Cayley graph can we retrieve from this set of quotients? And can we ever reconstruct the original Cayley graph from them: if so, then how?
Our answers to these questions involve a type of “relative Frattini subgroup” determined by the Cayley graph, which has similar properties to the Frattini subgroup of a finite group – I’ll discuss this and give some examples. It raises many new questions about Cayley graphs.