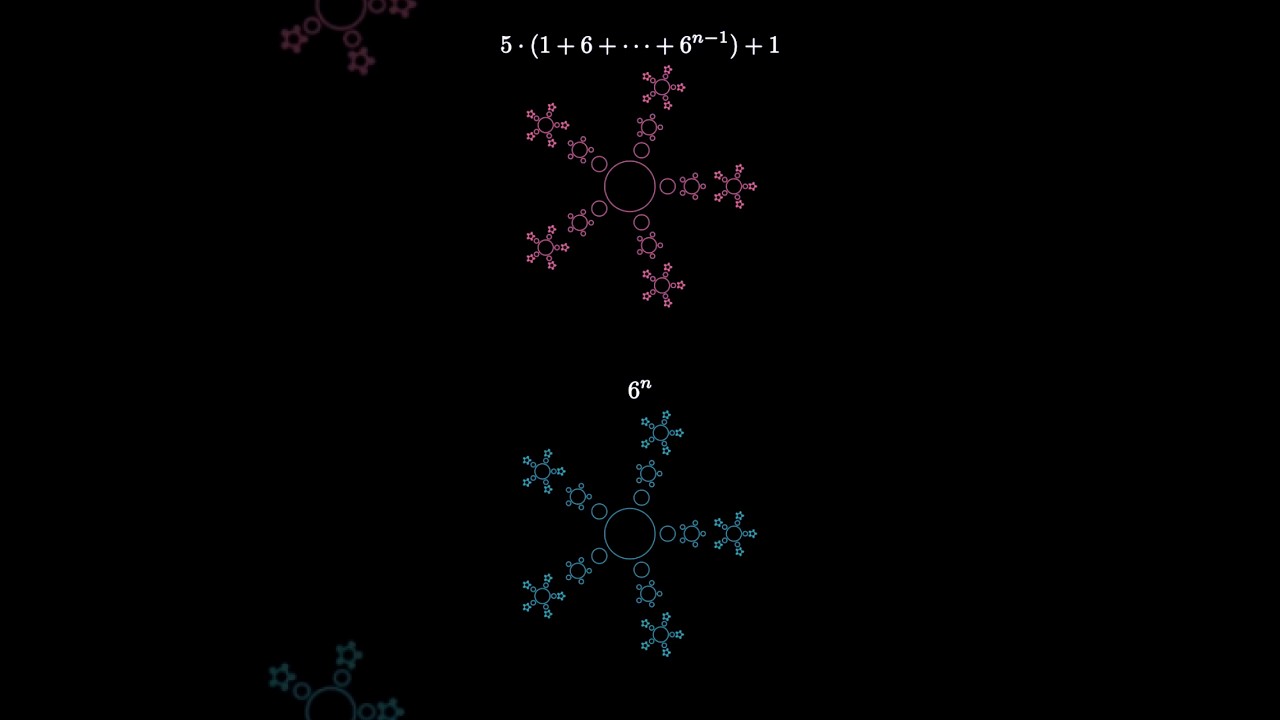
Summing Powers of 6 (geometric sum visualization)

This is a short, animated visual proof demonstrating the finite geometric sum formula for any integer n with n greater than 3 (explicitly showing the case n=6 with k=3). This series (and its infinite analog when x less than 1) is important for many results in calculus, discrete mathematics, and combinatorics.

Thanks!

#manim​ #math #geometricsums #series​ #calculus #mtbos​ #animation​ #theorem​ #pww​ #proofwithoutwords​ #visualproof​ #proof​ #iteachmath #finitesums #finiteseries #geometric #mathshorts​ #mathvideo​

To learn more about animating with manim, check out:

[mrbrown]

I am stunned by the advances in Audio Engineering
that allows us to distort the krap out of computer
generated audio files.

[حسينالقطري-بص]

I really like your videos on visualising things. Often I watch a single one of your videos 20-25 consecutive times.

It would be really appreciated if you visualise how you make these nice visualisations 😂😂😂🙂🙂🙂

But please, do some easy, summarised, step-by-step explanation, please 🙏

[valentinleguizamon]

you now are using edits music for math visual proofs, you're amazing. I love this so much 😔💓

[captainbucket]

your proofs are pure art, the moment it clicks in my mind feels amazing lol

[Noises]

ok i understand. n=3 in the graphic. kinda confusing if you dont know what your proving. which i dont what is the equation for?

[GutArthur]

That is literraly Bernouilli's equality, just transform 5 into 6-1 and transfert the 1 to the left and Gg

[GenericYigaFootsoldier]

Is that the red guy from Just Shapes & Beats

[pushkardiwan]

Don’t know when sum of powers of 10 will be useful but it is cool

[jonathanpopham]

Makes me think about primes and what they must be. There must be something to the primes fundamentally that we don't understand. Like numbers are just observations of some counting property like this graphic function. I wonder what's hidden about primes.

[mayuri]

(x-1)[Σx^(i-1)]=x^y, where i = 1 with an upper limit of y.

[DAMIENDMILLS]

So basically the sum of 6^i, where i=0-n equals 6^(n+1)/5

[ДанилаВласов-мя]

это всего лишь геометрические фракталы….

[Price-kllb]

Just wait until I unf 🥵 harness those numbers and create agh the power of math to oooo 🥵 no one will stop me 🤭