Area of polygons, including in a grid

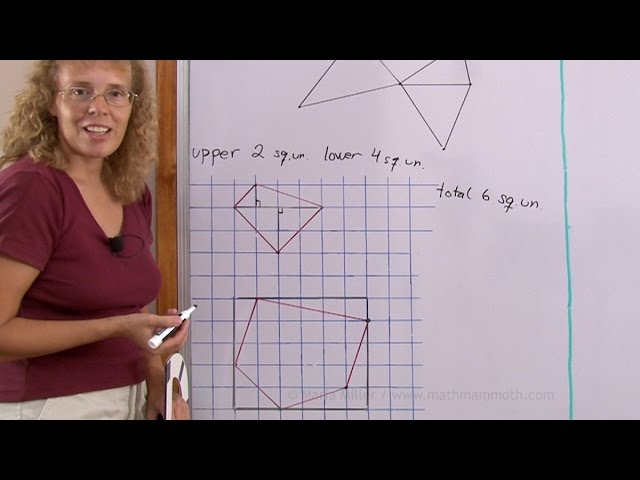
The basic principle for calculating the area of polygons, such as pentagons, hexagons, heptagons, and so on, is to divide the polygon into triangles (or other simple shapes), figure out the areas of those, and add them.

We do that for one quadrilateral. Then I show another method which works well for polygons in the coordinate grid. You draw a rectangle AROUND the polygon. Then you figure out the areas of the right triangles that are between the actual polygon and the surrounding rectangle, and subtract the areas of those from the area of the rectangle.

Lastly in this lesson we calculate the area of a trapezoid... not by using the formula, but by dividing it into two triangles and a rectangle. Nothing difficult there, but the main point we learn that it is SO very IMPORTANT to keep track of and record your intermediate calculations. :)

This geometry lesson is intended for 6th grade math.

Check out also my geometry worktext -- available both as a digital download and a printed copy:

[nicolestokes]

You have no clue how much I needed this ;)

[travelgangplanes]

i now understand the indian guy on youtube memes

[DesOwen]

If you call each intersection point on the perimeter, P and the internal intersections, I then, the area of the unit grid polygon is A = ((P-2)/2) + I. 😁