filmov
tv
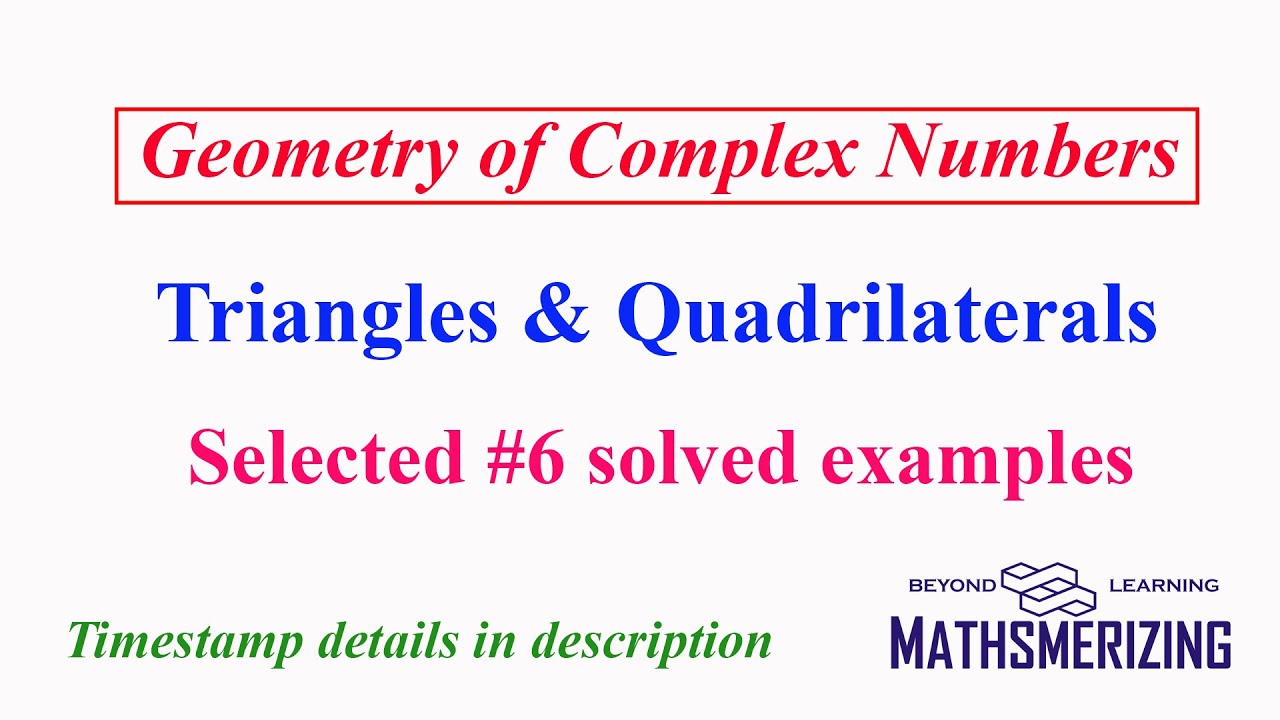
JEE Delight | Geometry of Complex numbers | Triangles & Quadrilaterals | Selected #6 solved examples
Показать описание
JEE Delight | Geometry of Complex numbers | Triangles & Quadrilaterals | Selected #6 solved examples
00:00 #SE1: If the area of the triangle on the complex plane formed by z, iz and z+iz is 50, then |z| is
01:24 #SE2: The points A, B and C depict the complex numbers z1, z2 and z3 respectively on a complex plane and the angle B and C is triangle are 1/2(pi-a) each. Show that (z2-z3)^2=4sin^2(a/2)(z3-z1)(z1-z2)
06:10 #SE3: If z1, z2, z3 and z4 are the vertices of a square in order then which of the following are true (z1-z3)/(z2-z4) is imaginary?
08:29 #SE4: Suppose z1+z2+z3+z4=0 and |z1|=|z2|=|z3|=|z4|=1. If z1, z2, z3 and z4 are vertices of a quadrilateral, then the quadrilateral must be?
12:18 #SE5: Let z1, z2, z3 and z4 be the vertices A, B, C and D respectively for a square on the argand diagram, then prove that: 2z2=(1+i)z1+(1-i)z3
14:31 #SE6: Prove that the roots of the equation 1/z-z1+1/z-z2+1/z-z3=0 where z1, z2 and z3 are pairwise distinct complex numbers, corresponds to points on a complex plane which lie inside a triangle with vertices z1, z2 and z3 or on its sides. Support the channel:
00:00 #SE1: If the area of the triangle on the complex plane formed by z, iz and z+iz is 50, then |z| is
01:24 #SE2: The points A, B and C depict the complex numbers z1, z2 and z3 respectively on a complex plane and the angle B and C is triangle are 1/2(pi-a) each. Show that (z2-z3)^2=4sin^2(a/2)(z3-z1)(z1-z2)
06:10 #SE3: If z1, z2, z3 and z4 are the vertices of a square in order then which of the following are true (z1-z3)/(z2-z4) is imaginary?
08:29 #SE4: Suppose z1+z2+z3+z4=0 and |z1|=|z2|=|z3|=|z4|=1. If z1, z2, z3 and z4 are vertices of a quadrilateral, then the quadrilateral must be?
12:18 #SE5: Let z1, z2, z3 and z4 be the vertices A, B, C and D respectively for a square on the argand diagram, then prove that: 2z2=(1+i)z1+(1-i)z3
14:31 #SE6: Prove that the roots of the equation 1/z-z1+1/z-z2+1/z-z3=0 where z1, z2 and z3 are pairwise distinct complex numbers, corresponds to points on a complex plane which lie inside a triangle with vertices z1, z2 and z3 or on its sides. Support the channel:
JEE Delight | Geometry of complex number | Circles | SE#10 | JEE Advanced 2013
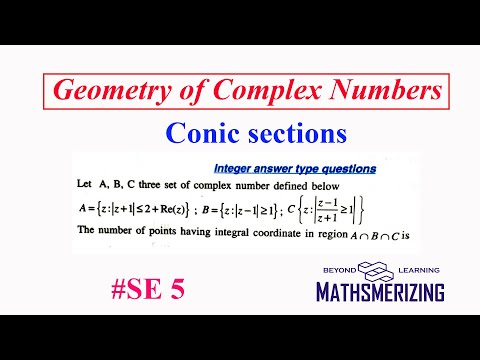
JEE Delight | Geometry of complex number | Conic sections | SE#5 |
JEE Delight | Geometry of complex number | Circles | SE#6 | JEE Advanced 2013
JEE Delight | Geometry of complex number | Circles | #3 Selected solved examples
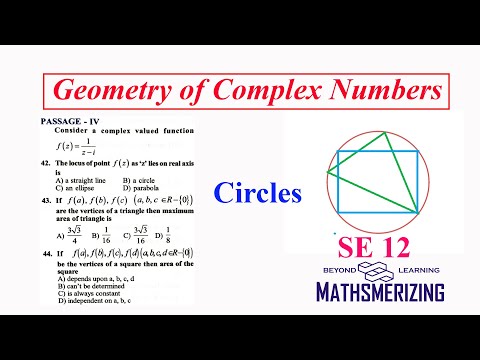
JEE Delight | Geometry of complex number | Circles | SE#12 | Triangle & square | Complex functio...
JEE Delight | Geometry of complex number | Selected 8 solved example | Some Important locus | Part 1
JEE Delight | Geometry of complex number | Circles | SE#11 | MCQ type
Circles | JEE Delight | Two statements based on geometry of circles
JEE Delight | Simple yet elegant | max [r1+r2] for two touching circles inscribed in a semi-circle
JEE Delight | Geometry of complex number | Circles | SE#9 | JEE Advanced 2008
JEE Delight | Geometry of complex number | Conic sections | Some Important locus | Part 2 | #4 SE
JEE Delight | 3 D geometry | Equation of a plane | Practice questions
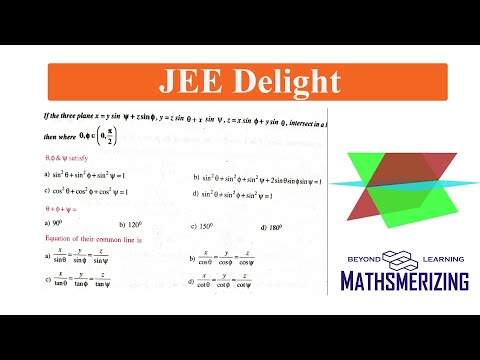
JEE Delight | 3 D geometry & Determinants | Passage | Three planes intersect in a line
JEE Delight | Geometry of complex number | Circles | #5 Selected solved examples | Timestamp details
JEE Delight | Geometry of complex number | Interaction of Circles | SE#7-8
JEE Delight | Circles | Lines 5x+12y-10=0 & 5x-12y-40=0 touch a circle C1 of diameter 6
JEE Delight | JEE Advanced 2023 | Paper1 | Q12 | 3 D geometry | Parallelopiped
JEE Delight | Geometry of complex number | Some Important locus | Part 1
JEE Delight | Circles | A circle trapped in a trapezoid | Matrix matching
JEE Delight | Geometry of Complex numbers | Lecture 7 | Triangle in a Circle | Selected #7 SE
JEE Delight | 3 D geometry | Vector equation of a line | Practice questions
JEE Delight | 3 D geometry | Intersection of vectors | Practice questions
JEE Delight | Two circles passing through two fixed points & touching a line intersect orthogona...
JEE Delight | Circles | All Common tangents to three given circles
Комментарии
 0:04:42
0:04:42
 0:03:45
0:03:45
 0:04:08
0:04:08
 0:06:53
0:06:53
 0:03:41
0:03:41
 0:12:07
0:12:07
 0:07:09
0:07:09
 0:09:30
0:09:30
 0:12:03
0:12:03
 0:05:37
0:05:37
 0:10:15
0:10:15
 0:05:18
0:05:18
 0:08:28
0:08:28
 0:11:40
0:11:40
 0:08:11
0:08:11
 0:04:46
0:04:46
 0:07:59
0:07:59
 0:15:12
0:15:12
 0:07:39
0:07:39
 0:17:36
0:17:36
 0:02:25
0:02:25
 0:05:49
0:05:49
 0:04:55
0:04:55
 0:08:46
0:08:46