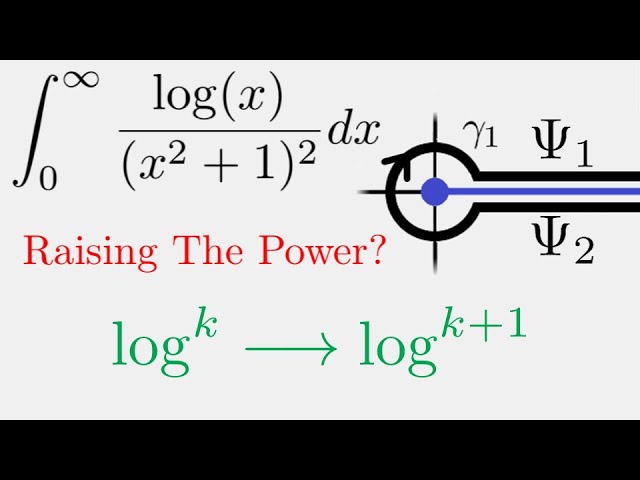
Complex Analysis: Integration Trick For Logarithms

Today, we discuss a trick used when integrating functions with logarithms on keyhole contours.
This is a rerecorded video, hopefully I've corrected all the mistakes from the original :)

[GingerMath]

After two years I found this video and it makes sense!! Thank you <3

[Uncpped]

Was a lot of work but quite elegant. Keep up the great work!

[parthasarathikondapure]

if you have a cut on the left side in wiki, then I think the argument runs from -pi to pi instead of 0 to 2pi

[haoqinggenius]

Why didn't u use the upper half semicircular contour for this integral?

[raymondhusser]

How are you assuming small gamma goes to zero when there is clearly a singularity there?

[navierstokes]

Can you generalized it with (x^n+1)^n in the denominator?

[polychromaa]

Do the integral of ln^2(x)/(x^2+1) and ln^3(x)/(x^2+1), both from 0 to infinity. They are much easier with complex methods, but i have evaluated the first integral with real methods.

[trannam]

At 2:02 why the line could not lie on the Real axis? I didn't see any poles on the Real axis

[fonzi]

I have one x^(i-1)/(x+1) from 1 to infinity

[darkseid]

But is it okay to take branch having argument 0 to 2pi ? because generally logarithms and exponentials in complex space is typically defined over -pi to pi. And if we take 0 to 2pi then we are going over a "domain cut". Or is there a flaw in my logic ?

[jameyatesmauriat]

Can i know why are you deepening your voice?