Math Olympiad Problem | Find X | x+y+z=0 |challenging Algebra Problem | Olympiad Mathematics

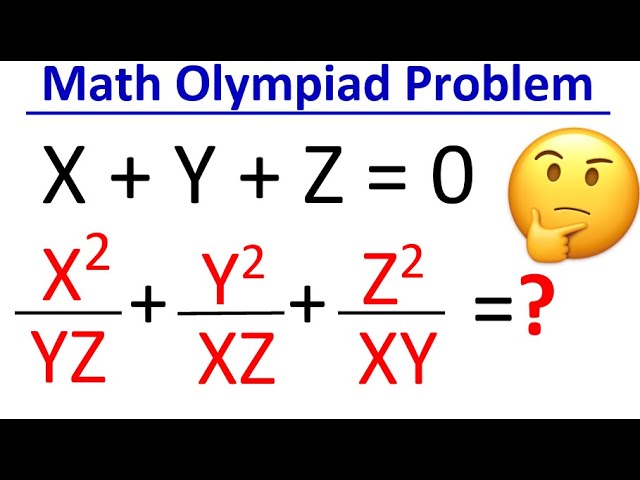
In this video, we have math Olympiad problems with solutions. we will solve this algebraic equation problem trending 2022. The problem is find the value of x^x/yz + y^2/xz + z^2/xy from x +y + z=0. Math Olympiad problems. System of equations
we will solve for all possible value of x^x/yz + y^2/xz + z^2/xy . This is actually one of the challenging math problems so do not ignore this video.

If you are new to my channel, please subscribe, like, comment and share.

[Icewallocumm]

Nice. - This just show me how beautiful math is. Ty, it really helped me!

[SuperYoonHo]

wow! thanks a lot for these videos mr. ____! i really appreciate these

[koenth]

It looks looks more natural and easier to first make the denominators equal. From the expression we have to evaluate, we infer that none of (x, y, z) is 0, so we may write

(x^3+y^3+z^3) / (xyz)  and substitute -(x+y) for z into that, to get

(x^3+y^3-(x+y)^3) / (-xy(x+y)) = (-3xy(x+y)) / (-xy(x+y)) = 3

[jorgepinonesjauch]

Debiera explicar porque se decide a despejar ( x+y) =- z y cuál es el motivo para después elevarlo al cubo? En cambio es preferible sumar la expresión final, donde aparece en el numerador: x^ 3+ y ^3+ z^

[sparky]

I took
x²/yz + y²/xz + z²/xy and I multiplied the fractions by x, y & z respectively.
So, it is :
x³/xyz + y³/xyz + z³/xyz
= (x³ + y³+ z³)/xyz

Now if x + y + z = 0
Then, x³ + y³+ z³ = 3xyz
So, (x³ + y³+ z³)/xyz becomes 3xyz/xyz
which is 3.