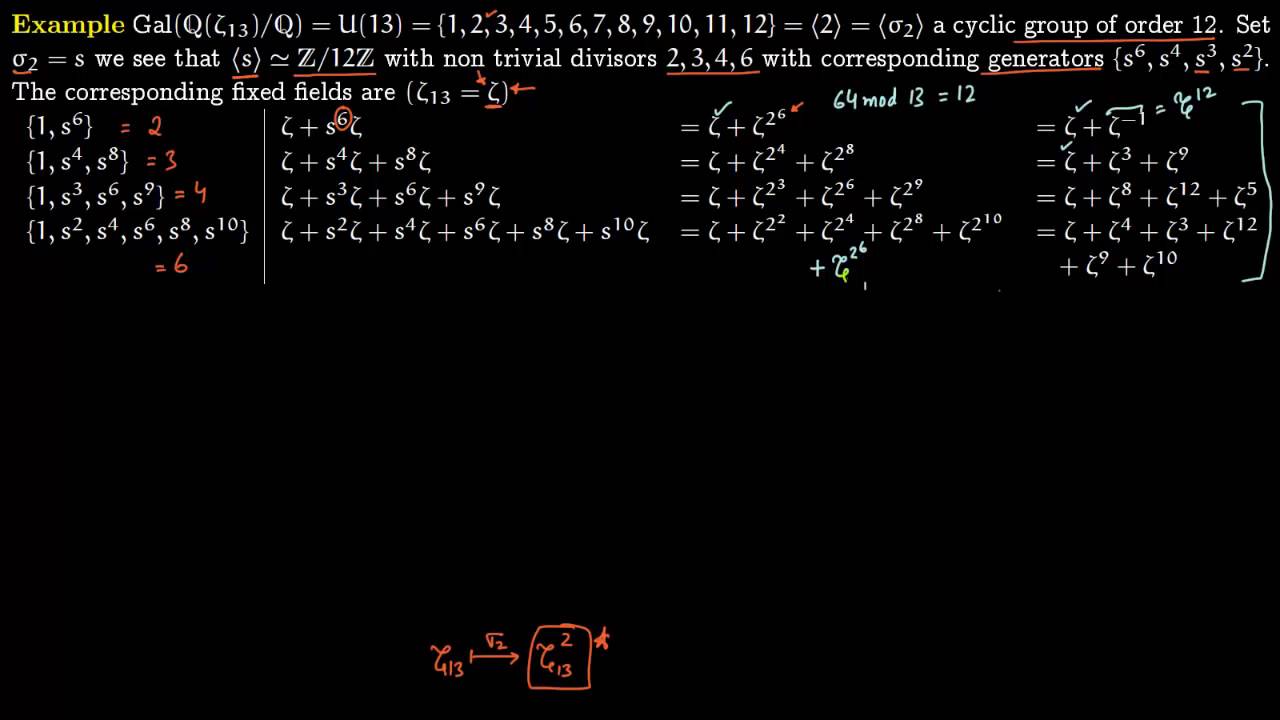
Example Galois Group Primitive Root

preview_player
Показать описание

  1. Harpreet Bedi
  2. Cyclotomic Polynomial
  3. Galois Group
  4. Mathematics (Field Of Study)
  5. Évariste Galois (Academic)
Рекомендации по теме
Example Galois Group 0:08:52

Example Galois Group Primitive Root

FIT4.3.2. Example of 0:06:10

FIT4.3.2. Example of Galois Group over Finite Field

Primitive Roots of 0:14:42

Primitive Roots of Finite Fields

Abstract Algebra II: 0:53:45

Abstract Algebra II: Theorem 55, 56, elementary Galois group examples, 1-23-17

Galois Group of 0:08:52

Galois Group of Cyclotomic Polynomial

Galois Groups Revisited 0:14:01

Galois Groups Revisited - Chapter 25

Galois theory: Primitive 0:15:20

Galois theory: Primitive elements

Visual Group Theory, 0:34:13

Visual Group Theory, Lecture 6.4: Galois groups

Galois Fields Lecture-1 0:17:47

Galois Fields Lecture-1

302.S9A: Galois Groups 0:13:49

302.S9A: Galois Groups and 'Stubborn' Polynomials

Galois Groups of 0:06:50

Galois Groups of Finite Fields

FIT4.3. Galois Correspondence 0:19:35

FIT4.3. Galois Correspondence 1 - Examples

That's Why Mohit 0:00:19

That's Why Mohit Sir Called 'God Of Mathematics'| Puzzle Brain teaser | #competishun ...

Galois Theory Lecture 0:19:03

Galois Theory Lecture 18: Galois Group of Irreducible Cubic Polynomials

Primitive nth root 0:20:09

Primitive nth root of unity, Examples Lec-22

Abstract Algebra, Lec 1:05:24

Abstract Algebra, Lec 38: Galois Group Example, Grad School Topics, Review for Final Exam

Galois Groups of 0:18:12

Galois Groups of Cyclotomic Fields (Algebra 3: Lecture 25 Video 1)

Visual Group Theory, 0:26:28

Visual Group Theory, Lecture 6.5: Galois group actions and normal field extensions

Information Coding Theory 0:10:27

Information Coding Theory Part 26 - Primitive Root and Polynomial, Primitive Test of Polynomial

Abstract Algebra II: 0:52:56

Abstract Algebra II: Galois group of polynomial, 3-2-18

An Example of 0:09:34

An Example of a Galois Group (Algebra 3: Lecture 17 Video 3)

Galois group example. 0:24:20

Galois group example.

Primitive Root 0:13:49

Primitive Root

The Galois group 0:35:25

The Galois group

Комментарии
Автор

Very helpful among all videos available on YouTube

kajalmittal
Автор

You took <2> as a generator and I see that you could have taken any of the numbers as a generator as 13 is prime and so every number in Z/13Z is relatively prime to 13. At about 5 minutes though you take (zeta^2)^k where k is a multiple of a particular divisor for the associated subgroup. I am guessing that you could have taken any <3>, <4> .. <12> as generators (sure <12> = <-1> etc.) and somehow gotten the same answers as you did as when <2> was chosen as a generator. So for example, how would one show that combining the sum of (zeta^5)^k = sum of (zeta^2)^k for k a multiple of a particular divisor? Thanks, and a huge thanks for posting these Harpreet! I am a huge fan!

Relative