Galois theory: Primitive elements

This lecture is part of an online graduate course on Galois theory.

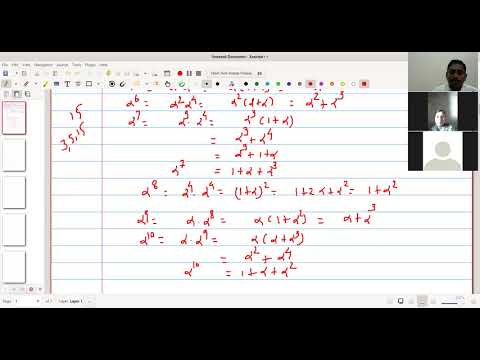
We show that any finite separable extension of fields has a primitive element (or generator) and given n example of a finite non-separable extension with no primitive elements.

[andreben]

Here's an alternative proof for the lemma about a finite number of vector subspaces. We first reduce to M=K^n and define the "twisted curve"
f: t in K |---> (1, t, t², ..., t^(n-1)).

Then since each subspace V_i is given by a non-zero linear form
(x_0, ..., x_{n-1}) |---> a_0*x_0+...+a_{n-1}*x_{n-1}


the t's for which f(t) is in V_i are the roots of a_0+...+a_{n-1}*t^{n-1}, which are a finite number of.
So if we rule out the finite union of these finite number of roots, for each V_i, then we can have a t0 in K, such that f(t0) is not in any V_i, since we assumed K to be finite.

[olivierbegassat]

I really appreciate these lectures, the exposition is very straightforward and clear, thank you for your work !

[m_yt]

This is a joy to follow. Thank you for uploading.

[gunhasirac]

Detail for infinitely many subextensions:

Let k = F_p, p prime. K = k(X, Y) ⊆ k(x, y), X = x^p, Y = y^p. Let f(X), g(X) ∈ K[X], f ≠ g. Write r = f(X)x + y, s = g(X)x + y. Then if K(r) = K(s), we can deduce x, y ∈ K(r), i.e. r is a primitive element, which contradicts the extension is not simple. Hence K(r) ≠ K(s) if f, g are distinct.

[hausdorffm]

5:38
Does any finite extension have the finite number of intermediate fields? Or is there any counter example?

The finiteness of the number of intermediate fields L, K < L < M cannot be deduced from finite algebraic extension? Is there any example of non-separable finite extension M/K so that there are infinitely many intermediate fields L, K < L < M.

Let k be a filed of characteristic 2 and suppose that t is not in k. Consider an extension L/K := k(t)/k(t^2). This is not a separable extension, because minimal polynomial of t over K is given by f(x) = x^2 – t^2 which is decomposed to (x-t)^2 on L. Because (x-t)^2 does not have distinct root, the extension L/K is not a separable extension. The extension L/K has infinitely many intermediate fields, for example, In this example, degree of extension is 2, so finite. What is intermediate fields?