filmov
tv
Germany Math Olympiad | A Very Nice Geometry Problem
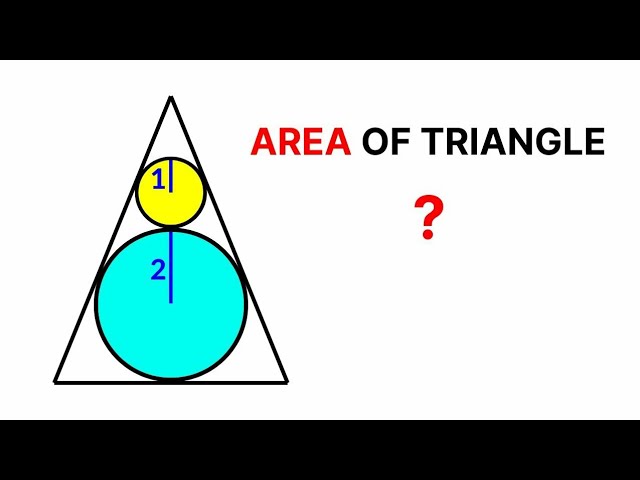
Показать описание
GET MY EBOOKS
•••••••••••••••••••••••
OTHER CHAPTERS : COMING SOON.....
--------------------------------------------------------------------------------
Join the channel to become a member
•••••••••••••••••••••••
OTHER CHAPTERS : COMING SOON.....
--------------------------------------------------------------------------------
Join the channel to become a member
South Korean l can you solve this exponential problem?? l Olympiad Math
Germany l can you solve?? l Olympiad Math exponential problem
Germany l can you solve?? l Hardest Olympiad Math problem
Germany - Math Olympiad Question
Germany Math Olympiad, a system of cubic equations
Germany Math Olympiad || A Nice Radical Math Simplification✍️🖋️💯
Germany | CAn You Solve This? | Math Olympiad
Germany | Can You Solve this? | A Nice Math Olympiad problem (x,y) =? 👇
Germany Math Olympiad || A Nice Radical Math Simplification✍️🖋️💯
Germany l can you solve this?? l Olympiad Math exponential problem
Germany | Can you solve this? | Math Olympiad
Germany ~ Can you solve this ?? | Math Olympiad
Germany - Math Olympiad Exponential Problem.
Germany || Can you solve this?|| Math Olympiad Problem🖊️📘✍️
Germany | Can You Solve? A Nice Maths olympiad Question |
Germany - Math Olympiad Challenge | Solve for integers a,b
Germany - Math Olympiad Question | The BEST Trick
Germany l Very Nice Olympiad Math Radical Problem
Germany - Math Olympiad Problem | Be Careful!
Germany Math Olympiad Problem | A Very Nice Geometry Challenge
Germany | A Nice Math Olympiad Exponential Equation | Solve for X ❓
Germany Math Olympiad: Solve for a!
Germany Math Olympiad | A Very Nice Geometry Problem
A math olympiad exponential math problem|Germany|solve for a
Комментарии
 0:12:58
0:12:58
 0:09:59
0:09:59
 0:18:54
0:18:54
 0:11:00
0:11:00
 0:11:36
0:11:36
 0:09:23
0:09:23
 0:12:47
0:12:47
 0:08:22
0:08:22
 0:10:12
0:10:12
 0:17:05
0:17:05
 0:08:51
0:08:51
 0:10:10
0:10:10
 0:04:45
0:04:45
 0:08:05
0:08:05
 0:08:13
0:08:13
 0:04:25
0:04:25
 0:10:37
0:10:37
 0:08:49
0:08:49
 0:10:06
0:10:06
 0:11:25
0:11:25
 0:07:20
0:07:20
 0:08:28
0:08:28
 0:13:53
0:13:53
 0:13:15
0:13:15