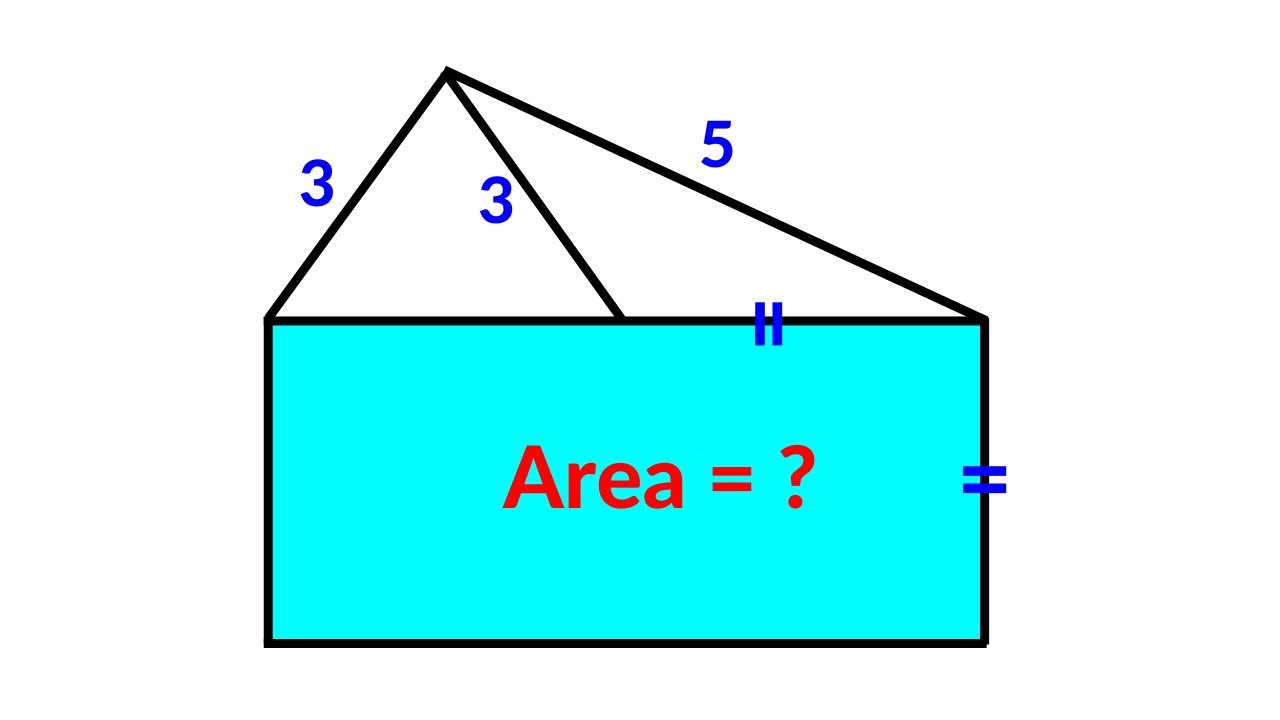
Math Olympiad | A Very Nice Geometry Problem

Math Olympiad | A Very Nice Geometry Problem

MY OTHER CHANNELS
••••••••••••••••••••••••••••••••

--------------------------------------------------------------------------------

Join the channel to become a member

[alexbayan]

If this is just a short answer question, collapse AB and AF then AFC is a right triangle with sides 3-4-5 and CD=FC=BC=4 so 4*4=16.
To prove it for the general case is simple too if you put angle ABF=AFB=theta;angle ACF=phi.
BC = 3*cos theta + 5+ cos phi ; FC = 5*cos phi - 3* cos theta
Also 3 * sin theta = 5 * sin phi; square both sides and 16=25 - 9=25 * (cos phi)^2 - 9 * (cos theta)^2 = BC * FC = BC * CD
This is essentially the same as your solution.

[jimlocke]

I think many of us will be able to get to the diagram at 2:35. When we then use the Pythagorean theorem to write equations, we have 2 equations, b² + h² = 3² and (a+b)² + h² = 5², but 3 unknowns, a, b, and h. We can't find a third equation. However, we note that we are solving for the area of the rectangle, a(a+2b), and do not need to solve for a, b and h separately. In fact, there may be many sets a. b and h which produce the same value for area = a(a + 2b). I like to try special cases. Let h = 3, collapsing ΔABM and ΔAFM into line segments of length 3, as alexbayan8302 did. Then, ΔACM is a right triangle with side 3 and hypotenuse 5, so the other side must be 4 (3 - 4- 5 Pythagorean triple). So, a = 4, b = 0, and BCDE is a square, which is a special case of a rectangle, and its area is 4² = 16. If this were a multiple choice test, we would assume that the area of the rectangle does not change as h varies over a valid range, and mark 16 as our answer. To support our suspicion of other solutions for a, b and h, let's let h = 2. Then, b = √5 and (a + b) = √(21), so a = √(21) - √5 and (a + 2b) = √(21) - √5 + 2√5 = √(21) + √5. Area of square = a(a + 2b) = (√(21) - √5)(√(21 + √5) = (√21)² - (√5)² = 21 - 5 = 16. We get the same result, 16, supporting our suspicions. So, we see if our set of 2 equations will produce an integer value for a(a + 2b):. b² + h² = 3² = 9, so h² = 9 - b². (a + b)² + h² = 5² expands to a² +2ab +b² + h² = 25, h² = 25 - (a² +2ab +b²) and h² = 25 - a² -2ab -b². Equating h², 9 - b² = 25 - a² -2ab -b² and a² + 2ab =16. We factor a² + 2ab into a(a + 2b) and find that the area of the rectangle is 16 for all valid (a, b) pairs. (Pairs are valid if a and b are both positive and produce a positive h which satisfies the 2 equations provided by the Pythagorean theorem.)

[hongningsuen]

This is a problem of Stewart's theorem in disguise.
The mnemonic for Stewart's theorem is "DAD is the MAN who puts the BoMB into the s(C)iNk(C)."
The equation is dad + man = bmb + cnc with the usual notations for the sides such that a = m + n, c is over m and b is over n in the triangle.
The area of rectangle in this problem is just (an) in the equation.
Hence transform the equation into an = (b^2 - d^2) + (c^2 - d^2)(n/m)
In this problem a = m + n, b = 5, c = 3, d =3.
Then an = (5^2 - 3^2) = 16.
Note that n/m has no fixed value in this problem.

[michaeldoerr]

This is probably the first time I have intuitively understood how HL congruency can be used to solve the area of a rectangle. All that is required is to draw the midpoint in the first triangle so that HL is justified and then the h is indented. After doing some algebra and Pythagoras Theorem is it simply substituting in what b is.

[quigonkenny]

Let DC = CF = x. Drop a perpendicular from A to BF at G. Let AG = h. As AB = FA, ∆FAB is an isosceles triangle, so AG bisects ∆FAB and creates two congruent right triangles, ∆BGA and ∆AGF. Therefore, BG = GF = y.

Triangle ∆BGA:
BG² + AG² = AB²
y² + h² = 3²
h² = 9 - y²

Triangle ∆AGC:
AG² + GC² = CA²
h² + (x+y)² = 5²
h² = 25 - x² - 2xy - y²

9 - y² = 25 - x² - 2xy - y²
x² + 2xy = 16
x(x+2y) = 16

Note that the length of ED, which is the width of rectangle BEDC, is equal to x+2y. Also, the length of DC, which is the height of rectangle BEDC, is equal to x. Therefore, the area of rectangle BEDC is equal to x(x+2y), which is equal to 16.

[IOSALive]

Math Booster, I subscribed because your videos are super cool!

[holyshit]

Let |BF|=y
Triangle ABF is isosceles so
cos(alpha) = y/6
From cosine rule in ABC
25= 3^2+(x+y)^2-2*3*(x+y)*y/6
16 = x^2+2xy+y^2-xy-y^2
16 = x^2+xy
16 = x(x+y)
Area = 16

[phungcanhngo]

Thank you professor for very nice solution.

[Irtsak]

Let FC=x, BF=y
Apply Stewart’s theorem in triangle ABC:
AB²⋅FC+AC²⋅BF=BC⋅(AF²+BF⋅FC)
9x+25y=(x+y)(9+xy)
9x+25y=9x+x²y+9y+xy² =>
x² y+xy²=16y => x²+xy=16 (y>0)
x(x+y)=16
=> area (BCDE)=16 square units

[RobG]

The disparity between the assertions made and the picture distracts from following the solution.
An accurate figure would be so useful.

[giuseppemalaguti]

Col teorema dei seni e coseno risulta FC=√10, BF=6/√10, CD=√10...Arett=(√10+6/√10)√10=16...ma non sono sicuro