filmov
tv
Proof: Recursive Identity for Binomial Coefficients | Combinatorics

Показать описание
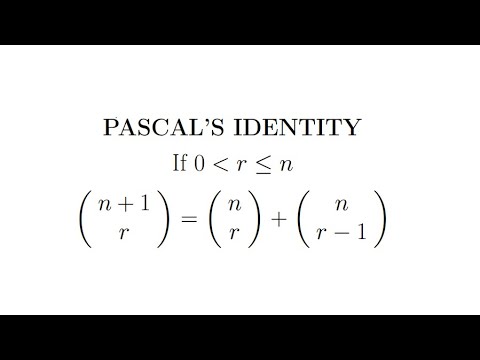
The binomial coefficient n choose k is equal to n-1 choose k + n-1 choose k-1, and we'll be proving this recursive formula for a binomial coefficient in today's combinatorics lesson!
This is the identity implicitly being used when we typically construct Pascal's triangle, and we can easily see why it's true by cleverly splitting the number of ways we can select k objects from n objects into two separate counts! Full details in the lesson!
I hope you find this video helpful, and be sure to ask any questions down in the comments!
+WRATH OF MATH+
Follow Wrath of Math on...
This is the identity implicitly being used when we typically construct Pascal's triangle, and we can easily see why it's true by cleverly splitting the number of ways we can select k objects from n objects into two separate counts! Full details in the lesson!
I hope you find this video helpful, and be sure to ask any questions down in the comments!
+WRATH OF MATH+
Follow Wrath of Math on...
Proof: Recursive Identity for Binomial Coefficients | Combinatorics
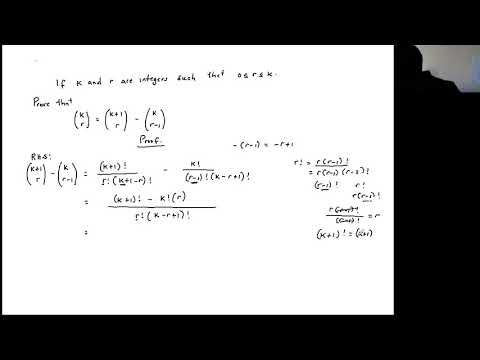
Pascal's Identity proof
Pascal's identity (algebraic proof)
Proving Binomial Identities
Induction with Sigma Notation (4 of 4: Binomial Theorem - Pascal's Identity)
My favorite proof of the n choose k formula!
Proof of Binomial Identity
Binomial Coefficient
Proving Binomial Identities (4 of 6: Finding pairings for constant term & proving identity)
How to Develop Recursion Formula for Binomial Distribution
Proof of Binomial Coefficients to follow Pascals Triangle
Story Proofs Explained: Binomial Coefficients and Vandermonde's Identity
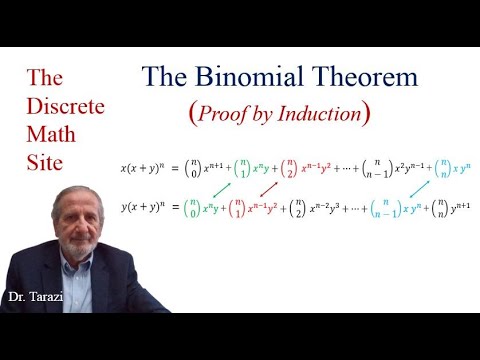
The Binomial Theorem Proof by Induction
How To Evaluate Binomial Coefficients
The Binomial Recurrence from Lattice Paths (visual proof)
Binomial Identity with Even Coefficients Only?
What Is The Recurrence Relation For The Binomial Coefficient?
Proving Binomial Identities (5 of 6: Techniques to filter and find greatest absolute coefficient)
Vandermonde's Identity in Combinatorics
CO19 Combinatorial Proofs of Binomial Identities
Combinatorial Proof (full lecture)
Pascal's Identity| Algebraic and Combinatorial Proof
Proving Harder Binomial Identities (3 of 3: Finding a pattern in the expression and prove identity)
Proof of the Binomial Theorem (Proof of the Formula)
Комментарии
 0:08:12
0:08:12
 0:05:43
0:05:43
 0:05:58
0:05:58
 0:13:12
0:13:12
 0:08:47
0:08:47
 0:13:36
0:13:36
 0:06:55
0:06:55
 0:03:37
0:03:37
 0:05:05
0:05:05
 0:11:35
0:11:35
 0:05:56
0:05:56
 0:05:07
0:05:07
 0:06:36
0:06:36
 0:08:37
0:08:37
 0:03:27
0:03:27
 0:14:28
0:14:28
 0:01:36
0:01:36
 0:04:56
0:04:56
 0:05:14
0:05:14
 0:24:00
0:24:00
 0:26:04
0:26:04
 0:07:14
0:07:14
 0:12:31
0:12:31
 0:16:08
0:16:08