filmov
tv
Fundamental Theorem of line integrals
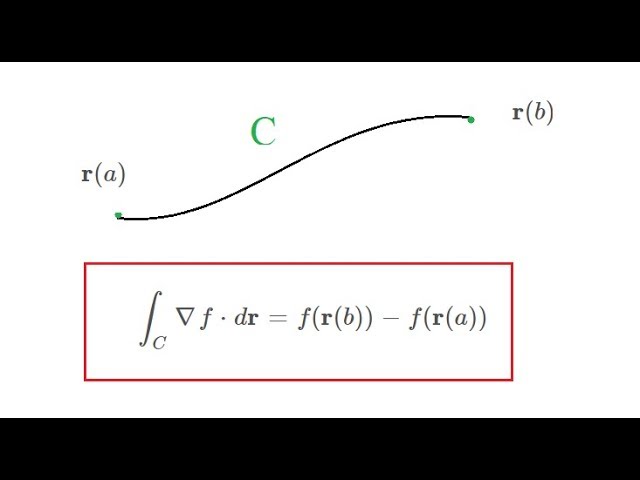
Показать описание
In this video, I present the fundamental theorem for line integrals, which basically says that if a vector field ha antiderivative, then the line integral is very easy to calculate. This illustrates why conservative vector fields are so important! I also provide a proof of the FTC, which uses... the chen lu!!! Finally, I show why we need the condition P_y = Q_x to check if a vector field is conservative. Enjoy!
The Fundamental Theorem of Line Integrals // Big Idea & Proof // Vector Calculus
The Fundamental Theorem for Line Integrals
Fundamental Theorem of line integrals
The Fundamental Theorem of Line Integrals - Part 1
Solve the Line Integral with the Fundamental Theorem of Line Integrals - 16.3.14 Cengage
16.3: The FundamentalTheorem for Line Integrals
Example of the Fundamental Theorem of Line Integrals
Fundamental Theorem for Line Integrals :: Conservative Vector Field Line Integral
The Secret Key to Riemann Hypothesis
Evaluating Line Integrals
The Fundamental Theorem for Line Integrals
Fundamental Theorem for Line Integrals
Calculus 3: Line Integrals (44 of 44) What is the Fundamental Theorem for Line Integrals? Ex. 2
Fundamental Theorem for Line Integrals
Fundamental Theorem of Line Integrals
Multivariable calculus 4.2.2: Proof of the fundamental theorem of line integrals
Line Integrals on CONSERVATIVE Vector Fields (Independence of Path): Calculus 3 Lecture 15.4
The Fundamental Theorem of Line Integrals - Part 2
Calculus 16.3 Fundamental Theorem for Line Integrals
Line integrals: Fundamental theorem
Calculus 3: The Fundamental Theorem for Line Integrals (Video #29) | Math with Professor V
The Fundamental Theorem of Gradients | Multivariable Calculus
Calc 3 16.3 Notes: The Fundamental Theorem for Line Integrals
Solve the Line Integral Directly & with the Fundamental Theorem of Line Integrals - 16.3.13 Ceng...
Комментарии
 0:06:38
0:06:38
 0:04:16
0:04:16
 0:15:36
0:15:36
 0:09:15
0:09:15
 0:07:23
0:07:23
 0:43:34
0:43:34
 0:14:21
0:14:21
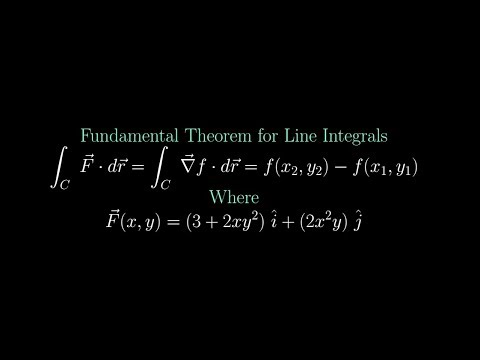 0:08:09
0:08:09
 0:43:26
0:43:26
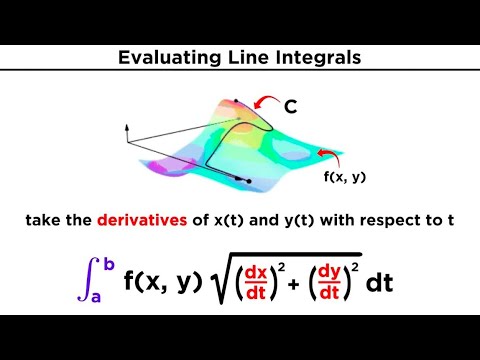 0:12:54
0:12:54
 0:09:41
0:09:41
 0:14:03
0:14:03
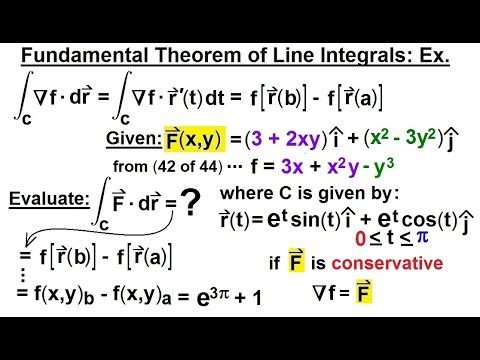 0:07:03
0:07:03
 0:08:18
0:08:18
 0:16:25
0:16:25
 0:05:30
0:05:30
 1:53:58
1:53:58
 0:07:10
0:07:10
 0:35:10
0:35:10
 0:19:37
0:19:37
 1:02:18
1:02:18
 0:19:35
0:19:35
 0:28:08
0:28:08
 0:13:49
0:13:49