filmov
tv
Find the derivative implicitly. #calculus

Показать описание
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
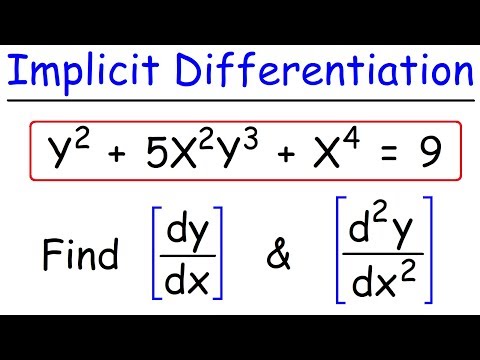
Implicit Differentiation - Find The First & Second Derivatives
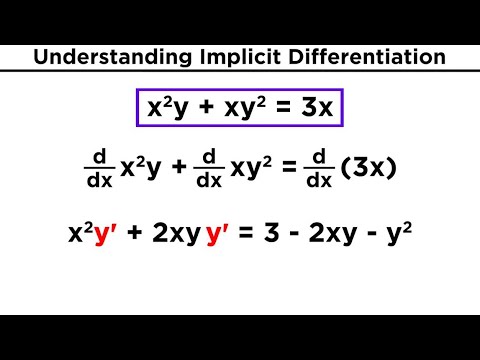
Implicit Differentiation
Find the derivative implicitly. #calculus
Implicit Differentiation
Second derivatives (implicit equations): find expression | AP Calculus AB | Khan Academy
How to Do Implicit Differentiation (NancyPi)
Calculus 1 Lecture 2.7: Implicit Differentiation
Second Derivative Implicitly | Calculus 1 Exercises
Partial Derivatives of Second Order | Higher Order | Part-I | SYBSc KBCNMU JALGAON
Implicit Differentiation for Calculus - More Examples, #1
Implicit Differentiation With Partial Derivatives Using The Implicit Function Theorem | Calculus 3
Calculus - Derivatives - Implicit Differentiation (1 of 3)
Implicit differentiation, what's going on here? | Chapter 6, Essence of calculus
Calculus 1: Implicit Differentiation (Video #13) | Math with Professor V
Calculus Implicit Differentiation Find dy/dx Example 3
Implicit differentiation | Advanced derivatives | AP Calculus AB | Khan Academy
Tangent Line with Implicit Differentiation | Calculus 1
Implicit Differentiation | 10 Practice Problems | Calculus 1
Implicit Differentiation 3x^2 + 2xy + 4y^2 Calculus MCV4U
Calculus 1 CH 5.2 Second and Higher Order Derivatives (7 of 11) Implicit Differentiation (Ex. 1)
MultiVariable Calculus - Implicit Differentiation
Finding the 2nd Derivative using Implicit Differentiation ❖ Calculus 1
Calculus 1: Lecture 2.5 Implicit Differentiation
Implicit Differentiation (for AP Calculus!)
Комментарии
 0:12:16
0:12:16
 0:11:45
0:11:45
 0:00:44
0:00:44
 0:14:34
0:14:34
 0:04:47
0:04:47
 0:14:17
0:14:17
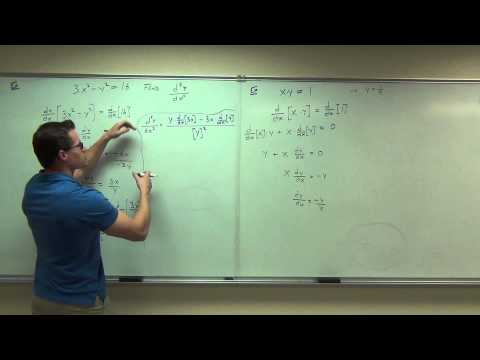 1:08:11
1:08:11
 0:07:02
0:07:02
 0:36:52
0:36:52
 0:03:51
0:03:51
 0:11:11
0:11:11
 0:04:37
0:04:37
 0:15:34
0:15:34
 0:33:00
0:33:00
 0:03:26
0:03:26
 0:08:02
0:08:02
 0:08:23
0:08:23
 0:28:59
0:28:59
 0:03:52
0:03:52
 0:06:22
0:06:22
 0:05:51
0:05:51
 0:08:54
0:08:54
 0:57:07
0:57:07
 0:11:19
0:11:19