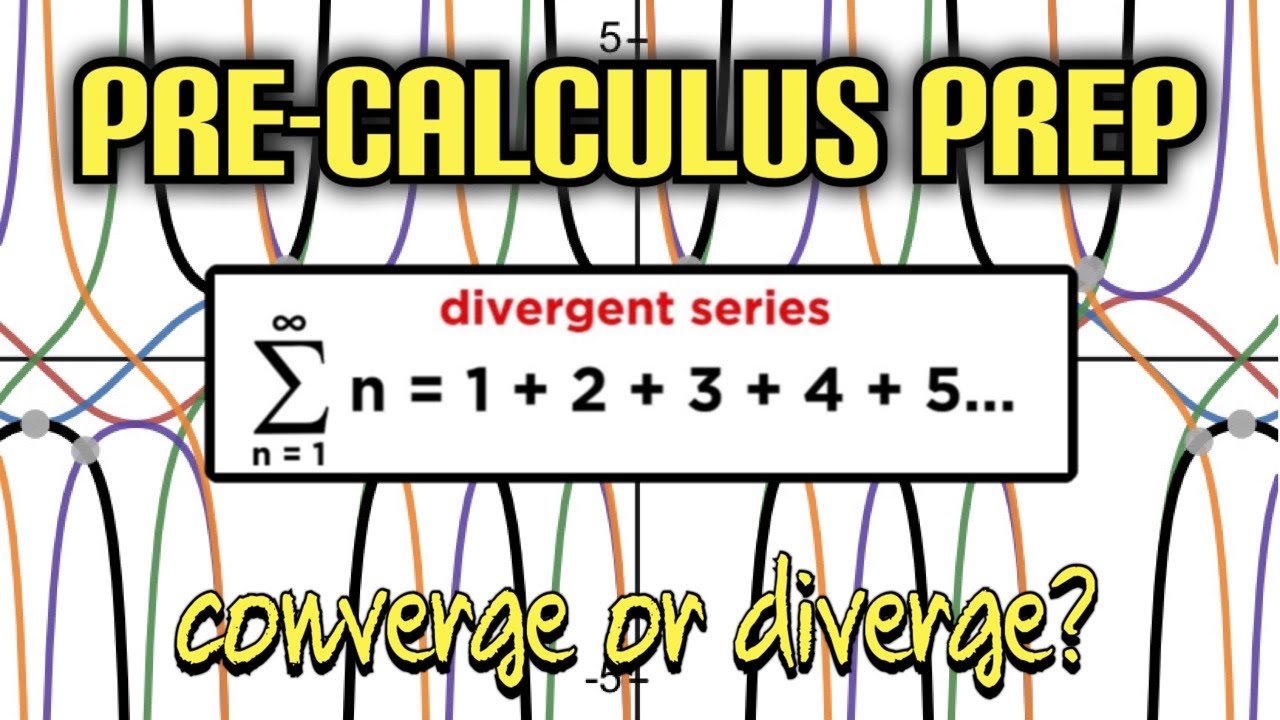
Pre-Calculus Prep: Does the Series Converge or Diverge?

In pre-calculus, determining whether a series converges or diverges is an important concept. A series is said to converge if the sum of its terms approaches a finite value as the number of terms increases. On the other hand, a series is said to diverge if the sum of its terms does not approach a finite value and instead grows infinitely or approaches positive or negative infinity.

To determine whether a series converges or diverges, there are several tests and criteria that can be applied. Here are some common ones:

1. Geometric Series Test: A geometric series of the form ∑(ar^(n-1)) converges if the absolute value of the common ratio, |r|, is less than 1. It diverges if |r| is greater than or equal to 1.

2. Divergence Test: If the terms of a series do not approach zero as n approaches infinity, then the series diverges. However, if the terms approach zero, the test is inconclusive, and other tests must be used.

3. Integral Test: For a series ∑(f(n)), where f(n) is a positive, continuous, and decreasing function, the series converges if the corresponding improper integral ∫(f(x)) converges. If the integral diverges, the series also diverges.

4. Comparison Test: If 0 ≤ a(n) ≤ b(n) for all n and the series ∑(b(n)) converges, then the series ∑(a(n)) also converges. If the series ∑(b(n)) diverges, then the series ∑(a(n)) also diverges.

5. Alternating Series Test: An alternating series of the form ∑((-1)^(n-1)a(n)) converges if the terms are decreasing (|a(n+1)| ≤ |a(n)|) and the limit of the terms approaches zero as n approaches infinity.

These are just a few examples of tests that can be used to determine the convergence or divergence of a series. It's important to note that there are many other tests available depending on the specific characteristics of the series being studied. Applying these tests can help you determine whether a series converges or diverges and provide insights into the behavior of the series as the number of terms increases.

These videos are designed to review and reteach Precalculus and Collegeboard Pre-CALC AP content. My videos cover functions, polynomials, exponential and logarithmic expressions, trigonometry, parametric equations, polar coordinates, vectors, matrices and systems, conic sections, discrete mathematics, sequences and series; and an introduction to calculus.

Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa .
.
.
#math #maths #mathskills #mathsucks #mathstudent #mathsmemes #mathstudents #mathsteacher #mathsisfun #gcsemaths #quickmaths #mathstutor #mathsclass #mathstricks #mathsjokes #brunomathsson #mathstations #mathslover #mathsproblems #mathsfun #alevelmaths #earlymaths #mathsquiz #mathsmeme #mathsmock #mathsnotes #mathsbeauty #ilovemaths #lovemaths #addmaths #mathsforlife #mathsweek #mathsgames #mathsexam #eyfsmaths #mathsrevision #primarymaths #ihatemaths #mathslesson #mathstuition