Why is a differentiable function necessarily continuous? - Week 3 - Lecture 7 - Mooculus

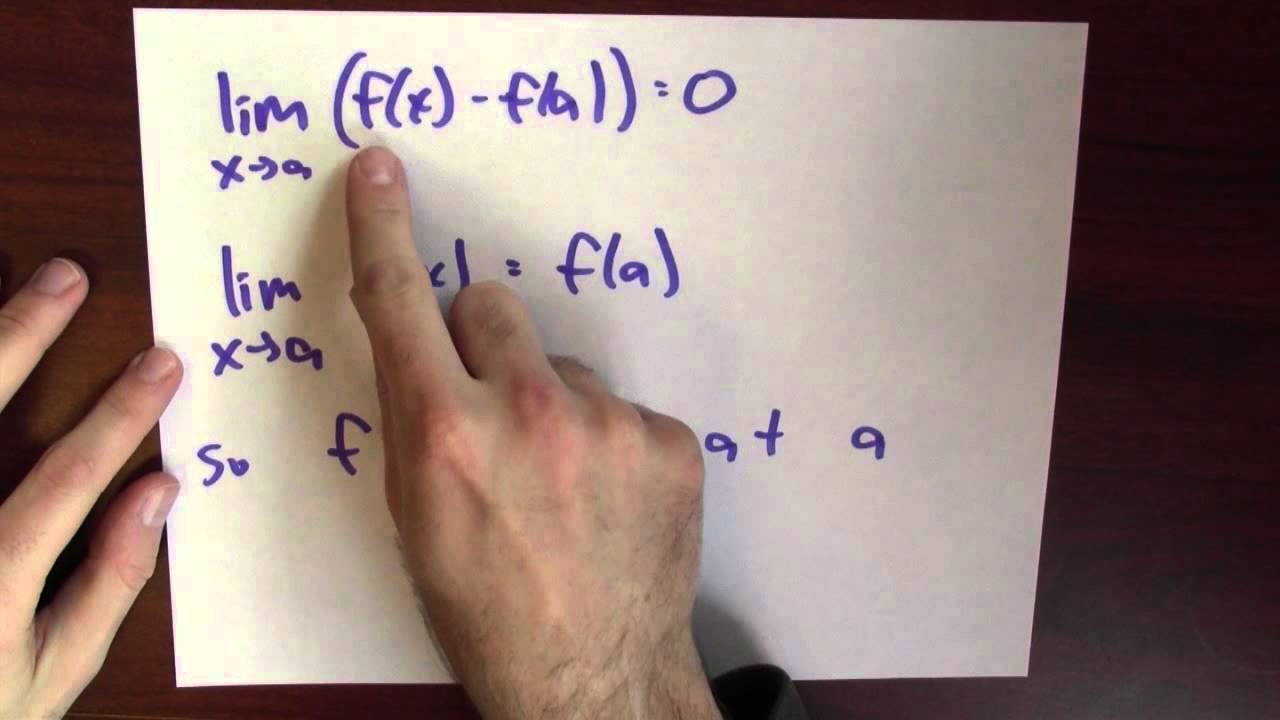
preview_player
Показать описание
  1. Jim Fowler
  2. mathematics
  3. calculus
  4. mooculus
  5. math
  6. maths
Рекомендации по теме
Continuity and Differentiability 0:05:33

Continuity and Differentiability EXPLAINED with Examples

Where a function 0:03:35

Where a function is not differentiable | Taking derivatives | Differential Calculus | Khan Academy

Differentiability (Formal Definition) 0:04:12

Differentiability (Formal Definition)

Why is a 0:06:02

Why is a differentiable function necessarily continuous? - Week 3 - Lecture 7 - Mooculus

What exactly is 0:08:56

What exactly is a differentiable function? Differentiability examples

Continuity and Differentiability 0:32:48

Continuity and Differentiability

Differentiable Functions 0:09:46

Differentiable Functions

How to determine 0:02:21

How to determine if a function is continuous and differentiable

Class 12 maths 0:18:38

Class 12 maths chapter- 5, Continuity and Differentiability, exercise 5.4 #imp questions #by pc sir

Differentiability and continuity 0:09:38

Differentiability and continuity | Derivatives introduction | AP Calculus AB | Khan Academy

Differentiability at a 0:06:21

Differentiability at a point: algebraic (function isn't differentiable) | Khan Academy

A Proof that 0:03:55

A Proof that Differentiable Functions are Continuous

Differentiable Functions 0:05:31

Differentiable Functions

Differentiable Function 0:10:32

Differentiable Function

Differentiable function 0:05:05

Differentiable function

Screencast 1.7.4: Determining 0:04:41

Screencast 1.7.4: Determining differentiability graphically

This Infinitely Differentiable 0:11:54

This Infinitely Differentiable Function Breaks Taylor Series

Differentiability of Piecewise 0:10:13

Differentiability of Piecewise Functions - Calculus

Lecture 18: Weierstrass's 1:15:36

Lecture 18: Weierstrass's Example of a Continuous and Nowhere Differentiable Function

Continuity vs Partial 0:09:11

Continuity vs Partial Derivatives vs Differentiability | My Favorite Multivariable Function

f(x)=sin(x) is a 0:02:57

f(x)=sin(x) is a Differentiable Function

Differentiability of a 0:12:35

Differentiability of a function - an example

Differentiability at a 0:06:38

Differentiability at a point for a piecewise function AP Calculus

Learn how to 0:02:37

Learn how to determine if a piecewise function is continuous and differentiable

Комментарии
Автор

Very clear, instructive and student-friendly video! I have a simple remark, more logical than mathematical. Maybe saying at the end of the video that differentiability and continuity are connected could be misleading. The reason is that " being connected to" is ( in ordinary language) a symmetric relation. So it suggests that continuity is linked to differentiability in the same way as differentiability is linked to continuity. But, as you explained before very clearly, the implication does not "work" both ways: differentiability is actually " connected" to continuity ( continuity is a necessary condition of differentiability) but continuity is not connected to differentiability ( continuity is not a sufficient condition of differentiability).

raylittlerock
Автор

I also know examples of cloudy days without rain: if it is snow, hail, sleet, et.c. Then it isn't really rain faling.

I know a function that is continuous everywhere, but never differentiable:

sum(n=0 to infinity) (a^n * cos (pi(b^n)x))

martinnolin
Автор

I love your energy!! Thanks your video was of great help!!

nourmahmoud