filmov
tv
Differentiability at a point for a piecewise function AP Calculus
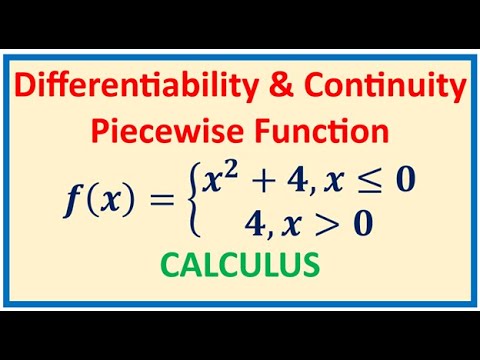
Показать описание
#limits_calculus #calculusanilkumar #mcv4u_limits #IBSLcalculus #tangentsandnormals #limits_substitution #edexcel_limits #edexcel_calculus #limits_indeterminants
Continuity
The function f is continuous at x=c iff f(c) exists
lim┬(x→c)〖f(x)〗 exists
lim┬(x→c)〖f(x)〗=f(c)
Differentiability
The function f is differentiable at x=c if f'(c) has a numeric value
If f is differentiable at every value of x in an interval, then f is differentiable on that interval.
NOTE: The limits of f'(c) should exist for differentiability
Continuity
The function f is continuous at x=c iff f(c) exists
lim┬(x→c)〖f(x)〗 exists
lim┬(x→c)〖f(x)〗=f(c)
Differentiability
The function f is differentiable at x=c if f'(c) has a numeric value
If f is differentiable at every value of x in an interval, then f is differentiable on that interval.
NOTE: The limits of f'(c) should exist for differentiability
Differentiability at a point: graphical | Derivatives introduction | AP Calculus AB | Khan Academy
Differentiability at a point: algebraic (function isn't differentiable) | Khan Academy
Continuity and Differentiability EXPLAINED with Examples
Differentiability at a point for a piecewise function AP Calculus
Differentiability at a Point
Differentiability and continuity | Derivatives introduction | AP Calculus AB | Khan Academy
Continuity and Differentiability
DIFFERENTIABILITY AT A POINT ( DIFFERENTIABILITY OF A FUNCTION ) #3
NCERT CLASS 12 | Maths | Chapter 5 Continuity and Differentiability | part 2 | by Hit8OM
Differentiability at a point
Differentiability at a point: algebraic (function is differentiable) | AP Calculus AB | Khan Academy
Differentiability (Formal Definition)
Differentiability of Functions
Differentiability at a point
Differentiability of a function at a point
Differentiability at a point
How to determine the points of differentiability
Non-differentiability
How to determine if a function is continuous and differentiable
Differentiability of Piecewise Functions - Calculus
Differentiability of a Function
What is differentiability for multivariable functions??
Differentiability
Differentiability at a Point & Differentiability in a Set
Комментарии
 0:05:38
0:05:38
 0:06:21
0:06:21
 0:05:33
0:05:33
 0:04:36
0:04:36
 0:09:38
0:09:38
 0:32:48
0:32:48
 0:05:32
0:05:32
 0:43:42
0:43:42
 0:10:14
0:10:14
 0:05:01
0:05:01
 0:04:12
0:04:12
 0:07:51
0:07:51
 0:24:57
0:24:57
 0:21:35
0:21:35
 0:24:57
0:24:57
 0:04:10
0:04:10
 0:00:54
0:00:54
 0:02:21
0:02:21
 0:10:13
0:10:13
 0:19:34
0:19:34
 0:14:35
0:14:35
 0:06:22
0:06:22
 0:07:04
0:07:04