filmov
tv
Differentiability of Piecewise Functions - Calculus
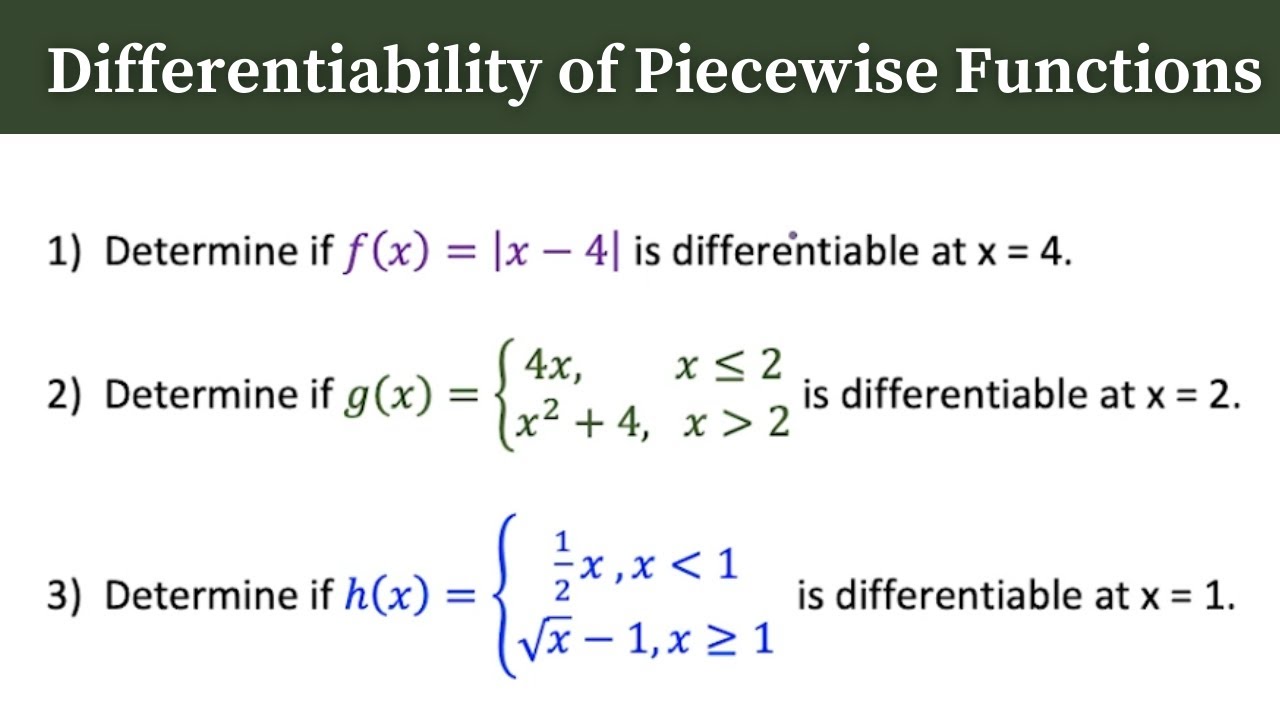
Показать описание
In this video, I go through 3 examples, showing how to verify that a piecewise function is differentiable. I show a few different methods; I show how to check for differentiability graphically, using the limit definition of differentiability and through numerical analysis.
If you found this video to be helpful, please LIKE and SUBSCRIBE. If you have any requests, leave the topics you want me to cover in the COMMENT section below. Thanks for watching!
If you found this video to be helpful, please LIKE and SUBSCRIBE. If you have any requests, leave the topics you want me to cover in the COMMENT section below. Thanks for watching!
Differentiability of Piecewise Functions - Calculus
Learn how to determine if a piecewise function is continuous and differentiable
Differentiability of Piecewise Functions
Differentiability and continuity of a piecewise function
Grade 12 Calculus - Differentiability of piecewise functions (ch 2)
Differentiability at a point for a piecewise function AP Calculus
Continuity and differentiability with piecewise functions
Continuity and Differentiability
Week-9|Doubt session - Maths 1
Determine the differentiability of piecewise functions
Differentiability of Piecewise Functions
Differentiability of Piecewise Function
Continuity and Differentiability EXPLAINED with Examples
How to determine Differentiability of Piecewise Functions Continuous not Differentiable Calculus
Check continuity and differentiability of a piecewise function
Differentiability of Piecewise Functions
Differentiability and Piecewise Functions
HOW TO CHECK DIFFERENTIABILITY OF PIECE - WISE FUNCTIONS
2.4b Finding Differentiability and Continuity of a Piecewise Function
Learn how to determine if a function is continuous and differentiable piecewise
Piecewise Functions - Limits and Continuity | Calculus
Differentiability of Piecewise Functions
Differentiability with piecewise function
Calc Video 2-5 Differentiability of Piecewise Functions
Комментарии
 0:10:13
0:10:13
 0:02:37
0:02:37
 0:04:48
0:04:48
 0:08:43
0:08:43
 0:09:40
0:09:40
 0:06:38
0:06:38
 0:06:36
0:06:36
 0:32:48
0:32:48
 2:43:40
2:43:40
 0:10:25
0:10:25
 0:11:54
0:11:54
 0:05:27
0:05:27
 0:05:33
0:05:33
 0:15:03
0:15:03
 0:00:56
0:00:56
 0:08:37
0:08:37
 0:06:46
0:06:46
 0:00:32
0:00:32
 0:04:52
0:04:52
 0:01:24
0:01:24
 0:10:06
0:10:06
 0:05:00
0:05:00
 0:09:20
0:09:20
 0:18:11
0:18:11