filmov
tv
Find Rank of a Matrix in Seconds! | Linear Algebra Exercises
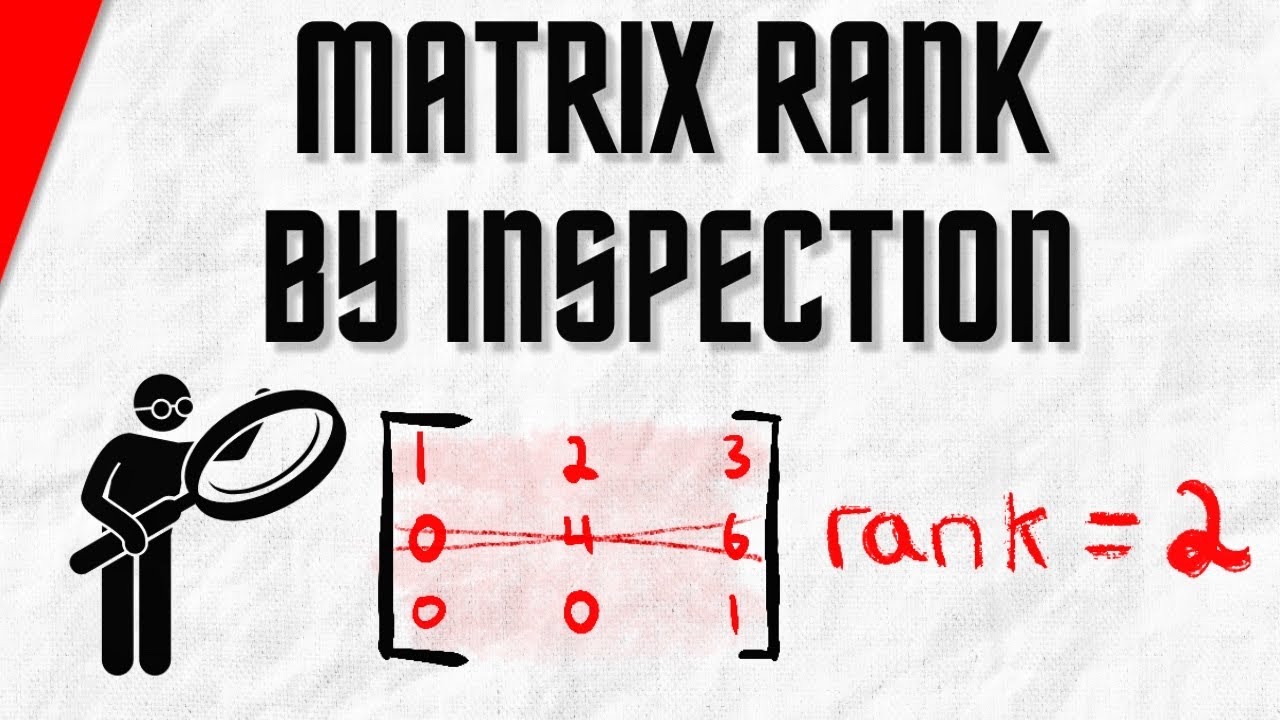
Показать описание
To find the rank of a matrix by inspection, we must try to identify how many linearly independent rows or columns it has. If the matrix or its transpose is in row echelon form, this can be done by counting the number of nonzero rows or columns. Otherwise, we need to consider linear combinations of the rows or columns and how one might be created from the others. We do several practice problems in this video, in each case finding the matrix rank with little more than a pinch of thought. #linearalgebra
◉Textbooks I Like◉
★DONATE★
Thanks to Loke Tan, Matt Venia, Micheline, Doug Walker, Odd Hultberg, Marc, Roslyn Goddard, Shlome Ashkenazi, Barbora Sharrock, Mohamad Nossier, Rolf Waefler, Shadow Master, and James Mead for their generous support on Patreon!
Outro music is mine. You cannot find it anywhere, for now.
Follow Wrath of Math on...
◉Textbooks I Like◉
★DONATE★
Thanks to Loke Tan, Matt Venia, Micheline, Doug Walker, Odd Hultberg, Marc, Roslyn Goddard, Shlome Ashkenazi, Barbora Sharrock, Mohamad Nossier, Rolf Waefler, Shadow Master, and James Mead for their generous support on Patreon!
Outro music is mine. You cannot find it anywhere, for now.
Follow Wrath of Math on...
How to Find the Rank of a Matrix (with echelon form) | Linear Algebra
The rank of a matrix
Find the Rank of a 4x4 Matrix (with echelon form) | Linear Algebra Exercises
Find Rank of a Matrix in Seconds! | Linear Algebra Exercises
Rank of matrix
How to Find Out Rank of Matrix
Linear Algebra - 22 - Rank
Find Rank of a Matrix in Seconds
L-5 RANK OF MATRIX BY ECHELON FORM | RANK AND NULLITY THEOREM | EXAMPLES | ENGINEERING MATHEMATICS
Method to find Rank Of Matrix | By Echelon Form (part4)
Mathematics: Finding Rank of Matrix
Finding the rank of the matrix using determinants
Linear Algebra. Rank of a matrix. Minor method.
Method to find Rank Of Matrix | By Definition of Rank (part5)
Rank Of The Matrix In 5 minutes 🔥. 7 Marks In Your Pocket
Rank of a Matrix : Data Science Basics
Dimension of the column space or rank | Vectors and spaces | Linear Algebra | Khan Academy
How do you Find Rank of a Matrix
Rank of Matrix Explained in Hindi | Engineering Mathematics
Find Rank of Matrix In 20 Seconds🤯| Best Tips & Tricks🥳#byjusgateprep #gate2023 #shorts #mathstr...
Find Rank of a 3x3 Matrix (with row echelon form) | Linear Algebra Exercises
🔷12 - Rank and Nullity of a given Matrix (Row Echelon Form)
Finding the Rank of a Matrix | Solved Example Pt.1 | Linear Algebra
Trick to find RANK of a Matrix /Rank of Matrix in Hindi /How to Find Rank of Matrix | Maths is Easy
Комментарии
 0:03:25
0:03:25
 0:17:17
0:17:17
 0:03:31
0:03:31
 0:07:59
0:07:59
 0:04:20
0:04:20
 0:08:05
0:08:05
 0:05:45
0:05:45
 0:00:52
0:00:52
 0:49:11
0:49:11
 0:05:15
0:05:15
 0:08:08
0:08:08
 0:02:49
0:02:49
 0:09:31
0:09:31
 0:05:58
0:05:58
 0:05:33
0:05:33
 0:14:34
0:14:34
 0:12:48
0:12:48
 0:06:22
0:06:22
 0:05:20
0:05:20
 0:00:56
0:00:56
 0:03:30
0:03:30
 0:22:10
0:22:10
 0:14:54
0:14:54
 0:06:46
0:06:46