filmov
tv
When a complicated proof simplifies everything

Показать описание
Also, a special book cover if you're fast enough. You'll be fast enough.
Huge thanks to my Patreon supporters. I’m a huge exponent of all of
them, minus none.
CORRECTIONS
- None yet, let me know if you spot anything!
Filming by Alex Genn-Bash
Editing by Gus Melton
Graphics by Sam Hartburn and Matt Parker
Written and performed by Matt Parker
Produced by Nicole Jacobus
Music by Howard Carter
Design by Simon Wright and Adam Robinson
MATT PARKER: Stand-up Mathematician
Website:
New book!
parker-signed
Huge thanks to my Patreon supporters. I’m a huge exponent of all of
them, minus none.
CORRECTIONS
- None yet, let me know if you spot anything!
Filming by Alex Genn-Bash
Editing by Gus Melton
Graphics by Sam Hartburn and Matt Parker
Written and performed by Matt Parker
Produced by Nicole Jacobus
Music by Howard Carter
Design by Simon Wright and Adam Robinson
MATT PARKER: Stand-up Mathematician
Website:
New book!
parker-signed
When a complicated proof simplifies everything
The 360-Page Proof That 1+1=2
The longest mathematical proof ever
Fast shortcut for nested square roots (plus the proof)
Euler's Formula Proof
Proof by induction | Sequences, series and induction | Precalculus | Khan Academy
Proof and Problem Solving - Logical Expression Simplification Example 02
Imaginary Numbers Are Real [Part 1: Introduction]
Trigonometric Functions | 1st PUC 11th CBSE | Mathematics | Part 5 of 10
Only U.S. President to prove a theorem
An Example Proof using Identities of Regular Expressions
How to Prove Trigonometric Identities (and how not to)
Necessity of complex numbers
Proof that Chinese is Common Sense
Proof anything will sound great if you are good at rhythm
CAN YOU PROVE | how We solve | simplifying complex numbers | Step-by-Step Explanation | L.HS = R.H.S
GCSE Maths 2023 Revision Algebraic Proof
The Riemann Hypothesis, Explained
Proof and Problem Solving - Logical Expression Simplification Example 01
Proof #2: Does 1 = 2?
x⁰=1 Proof in 10 seconds #challenge #maths #easy #proof #concept
Proof
Proof and Problem Solving - Logical Expression Simplification Example 03
Simplifying Trigonometric Expressions
Комментарии
 0:06:22
0:06:22
 0:06:03
0:06:03
 0:19:30
0:19:30
 0:05:09
0:05:09
 0:00:56
0:00:56
 0:09:23
0:09:23
 0:04:53
0:04:53
 0:05:47
0:05:47
 0:15:46
0:15:46
 0:01:00
0:01:00
 0:05:49
0:05:49
 0:07:37
0:07:37
 0:07:39
0:07:39
 0:00:30
0:00:30
 0:01:31
0:01:31
 0:07:58
0:07:58
 0:04:39
0:04:39
 0:16:24
0:16:24
 0:04:41
0:04:41
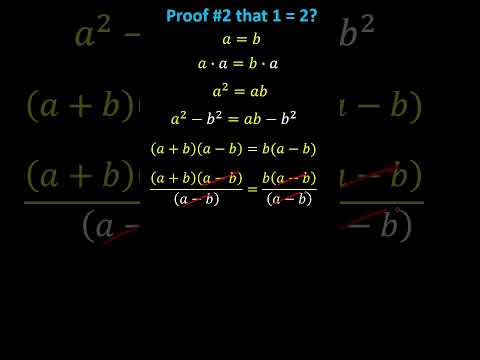 0:00:48
0:00:48
 0:00:20
0:00:20
 0:07:09
0:07:09
 0:04:46
0:04:46
 0:12:15
0:12:15