Prime Knots - Numberphile

They are knot what you think!
More links & stuff in full description below ↓↓↓

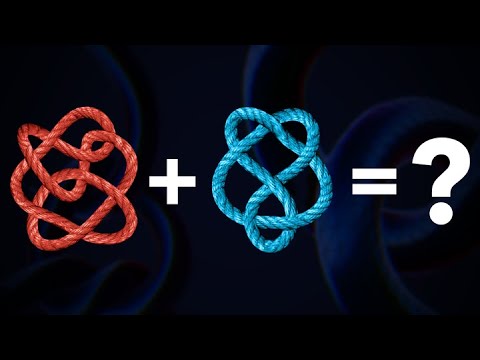
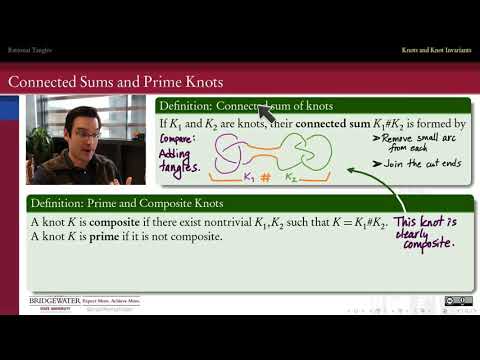
Primes, Composites and the usefulness of knots....
Second in a series of videos about knots. Here we again speak with Carlo H. Séquin from UC Berkeley.
Edit and animation by Pete McPartlan. Film and interview by Brady Haran

NUMBERPHILE

Videos by Brady Haran

[userrL]

Can we all just take a moment to appreciate how difficult it must have been for the animator do recreate these knots digitally.

[juliusdictatorperpetuus]

Professor: What's your favourite part of mathematics?
Student: Not theory.
Professor: Me too.

[Dixavd]

I'm with Brady: having just been explained the minimum number of knots, the idea of compound knots being ignored is revelatory.

[thomaskn]

Professor Carlo H. Séquin works at UC Berkeley.  Does he also teach at the University of Knottingham?

[thulyblu]

To be, or knot to be, that is the question (being addressed in this video)

[Madoc_EU]

With the help of these videos, I feel like knot theory is actually something really exciting, despite the limited usefulness. It seems to be one of the many places where mathematics and art meet.

[thomassomeone]

The animators on this channel are amazing! Where do I send the animator money?

[nagertwi]

someone drew all 165 knots with 10 crossings, only for them to be on the screen for about 2 seconds.

[PrincipalAgents]

Those vectors... :D :D

(w, h, a, t) * (e, v, e, r) ;)

[tsneak]

Fantastic explanations and animations. Thanks.

[yashovardhandubey]

"It's a special field of mathematics where you don't need to know much mathematics"
ME : SO YOU'RE SAYING THERE'S STILL A CHANCE

[SeanRhoadesChristopher]

I am so thrilled that there is a practical use for the abstract closed looped knots, but it was a surprised about compound knots. Moreover, I wonder if there is a fundamental theorem of knots, similar to arithmetic?

[anitejbanerjee]

Very interesting content and lovely animation!
Kudos to you, Brady, and thanks to professor Carlo :)

[electromika]

Knot that I want to repeat myself, I mean, I've tied this joke to the last video as well, but I just love doing this. I almost feel like I needle do this. What a yarn.

[bryanwan]

Hey. can you tie a knot?

No, I cannot.

Ah, so you can knot...

No I cannot knot.

Not knot?








Who's there?
-Rabbit, Piglet and Winnie the Pooh

[TheNefari]

Numberphile If you can combine these knos ...
is it possible to unknot a knot if you combine it with another knot, that has a special property?

[lawrencedoliveiro]

5:33 After he said at 2:38 that such cutting and rejoining was something that “mathematicians would never do” ...

[postbodzapism]

Well you don't need to know calculus for the first chapter, but then you need integration to do Kontsevich integral, matrices to do Seifert matrices and Alexander polynomial etc.
And also Fox calculus for some differentials if you count Fox derivative as differentiation.

[keniangervo]

Glad there's more videos coming about knot theory, somehow it interests me alot and I don't even know why but it does!

[simargl]

were there more "knot"s or "not"s in this video?