filmov
tv
Integral of xsec(x)tan(x) using integration by parts.
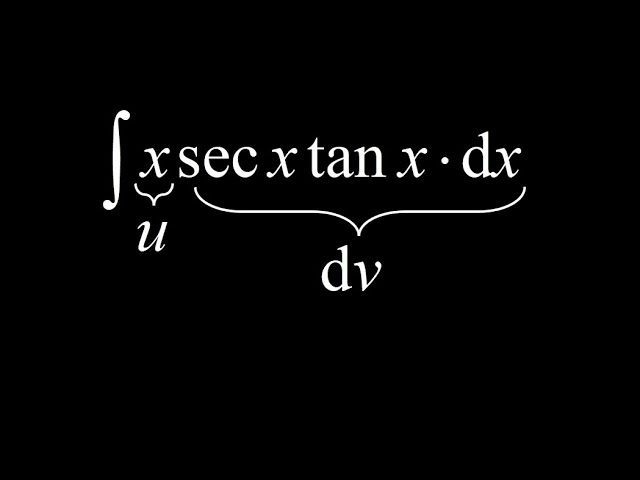
Показать описание
To compute the integral of xsec(x)tan(x), we have to recognize that sec(x)tan(x) is the derivative of the elementary trig function sec(x). In other words, the antiderivative of sec(x)tan(x) is just sec(x).
So, we let dv=sec(x)tan(x) and v=sec(x).
The other part of the integral is just x, so we let u=x and du=dx.
Using integration by parts, we get uv-integral(vdu) or xsecx-integral(sec(x)dx). Now we have to remember the integral of sec(x) which is ln|sec(x)+tan(x)|, and we're ready to write the final answer for the integral of xsec(x)tan(x) using parts: xsec(x)-ln|sec(x)+tan(x)|+C.
So, we let dv=sec(x)tan(x) and v=sec(x).
The other part of the integral is just x, so we let u=x and du=dx.
Using integration by parts, we get uv-integral(vdu) or xsecx-integral(sec(x)dx). Now we have to remember the integral of sec(x) which is ln|sec(x)+tan(x)|, and we're ready to write the final answer for the integral of xsec(x)tan(x) using parts: xsec(x)-ln|sec(x)+tan(x)|+C.
 0:01:57
0:01:57
 0:01:34
0:01:34
 0:03:27
0:03:27
 0:02:14
0:02:14
 0:05:04
0:05:04
 0:12:11
0:12:11
 0:02:46
0:02:46
 0:07:47
0:07:47
 0:08:54
0:08:54
 0:03:59
0:03:59
 0:16:51
0:16:51
 0:01:58
0:01:58
 0:01:20
0:01:20
 0:02:43
0:02:43
 0:04:10
0:04:10
 0:00:35
0:00:35
 0:02:09
0:02:09
 0:00:54
0:00:54
 0:02:48
0:02:48
 0:00:43
0:00:43
 0:05:59
0:05:59
 0:01:09
0:01:09
 0:02:23
0:02:23
 0:04:25
0:04:25