filmov
tv
Functional Limits and Continuity , Real Analysis II

Показать описание
In this video we begin our study of continuous functions between metric spaces. We start by explaining terminology, such as domain, codomain, image, and preimage, and how denote them. Then, we define a functional limit using the epsilon-delta definition, generalizing the concept of limits for functions that you've likely already seen (from R to R) to functions from one metric space to another. Then we look at the formal definition of continuity, emphasizing that a function is continuous at a point c if the limit as x approaches c equals the function’s value f(c) (or the point c is isolated in the domain A).
Then we do a quick example to show how to prove that a vector-valued function of two variables, f(x, y) = (2x, y+1), is continuous on its domain using the epsilon-delta method.
We wrap up with a brief discussion of the topological definition of continuity: the preimage of every open set in the codomain is open relative to the domain. This topological perspective will be revisited when exploring the four characterizations of continuity.
#mathematics #realanalysis #advancedcalculus #ContinuousFunctions #FunctionalLimit #MetricSpaces #EpsilonDelta #Continuity #Topology #MathEducation
Then we do a quick example to show how to prove that a vector-valued function of two variables, f(x, y) = (2x, y+1), is continuous on its domain using the epsilon-delta method.
We wrap up with a brief discussion of the topological definition of continuity: the preimage of every open set in the codomain is open relative to the domain. This topological perspective will be revisited when exploring the four characterizations of continuity.
#mathematics #realanalysis #advancedcalculus #ContinuousFunctions #FunctionalLimit #MetricSpaces #EpsilonDelta #Continuity #Topology #MathEducation
 0:10:10
0:10:10
 0:19:19
0:19:19
 0:20:20
0:20:20
 0:07:18
0:07:18
 0:10:06
0:10:06
 2:14:36
2:14:36
 0:11:32
0:11:32
 0:11:14
0:11:14
 0:47:42
0:47:42
 0:13:31
0:13:31
 0:11:08
0:11:08
 0:09:47
0:09:47
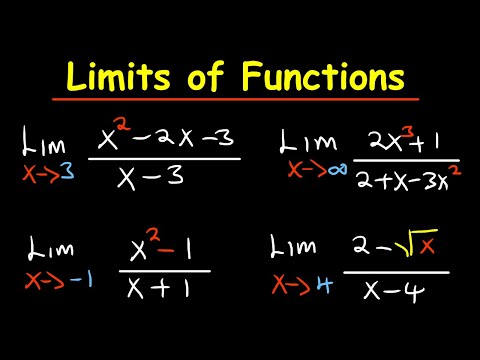 0:15:09
0:15:09
 0:12:22
0:12:22
 0:08:38
0:08:38
 0:03:49
0:03:49
 0:05:33
0:05:33
 0:11:08
0:11:08
 0:05:38
0:05:38
 0:19:04
0:19:04
 0:05:11
0:05:11
 0:21:38
0:21:38
 0:08:14
0:08:14
 0:11:15
0:11:15