Understanding Continuity in Calculus

Welcome to our enlightening YouTube video on continuity in calculus! In this comprehensive tutorial, we delve into the fascinating world of calculus and equip you with the knowledge and tools to confidently comprehend and analyze the concept of continuity.

Whether you're a student grappling with the intricacies of calculus or an enthusiast seeking a deeper understanding of mathematical analysis, this video is your gateway to unlocking the power of continuity. Our expert instructor breaks down complex concepts into digestible explanations, ensuring that you grasp the essence of continuity in calculus.

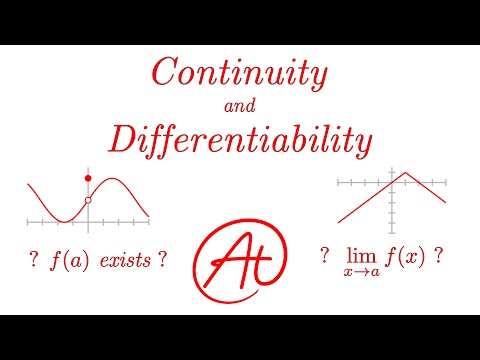
Join us as we explore the fundamentals of continuity, uncovering the essential characteristics and properties of continuous functions. Through clear explanations and illustrative examples, we demonstrate how continuity relates to the absence of abrupt changes, holes, or jumps in a function's graph.

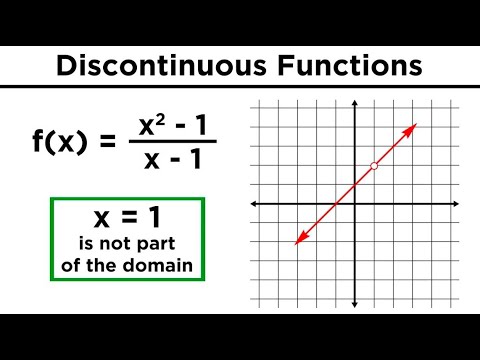
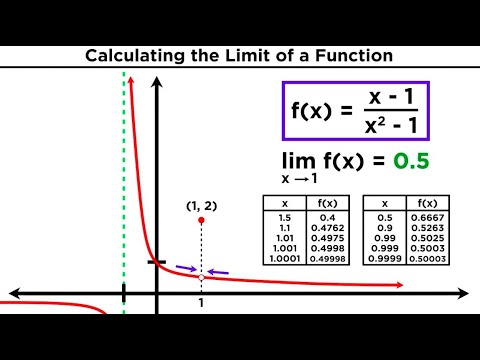
We unravel the secrets behind identifying and analyzing continuity, discussing concepts such as one-sided continuity, removable and non-removable discontinuities, and the intermediate value theorem. We showcase how continuity plays a crucial role in calculus, allowing for the application of powerful mathematical tools and techniques.

Through interactive visuals and step-by-step instructions, we make the learning process engaging and accessible. With our comprehensive explanations and insightful examples, you'll develop a solid foundation in understanding and applying continuity in calculus.

Don't let the complexities of calculus intimidate you any longer. Join us for this transformative tutorial and unlock the power to confidently comprehend and analyze continuity. Hit that play button now and embark on your journey to becoming a master of continuity in calculus!

[rubi]

Do I understand every video? No, but I still watch and thumbs up. This man makes the world a better place with nothing but a dry erase board, a brilliant brain, and some markers.

[_RS-SS]

Nice succinct, straightforward lesson. Thanks!

[johnroberts]

What an excellent lesson. Many thanks.

[Alan_kurde]

I've done it، and you've done it❤

[hemrajue]

A function is said to be continuous, if we can draw it's graph without lifting pen.
Eg. Sine function is continuous function whereas tangent function is discontinuous.

[RickRudy]

Well explained and to the point. Thank you!

[ogrikisamuel]

I'm really grateful Sir❤
Thank you

[apotheosisaurel]

Nice and clear, it helped me review the topic, tysm.

[sneha]

It was actually helpful than my imagination 😊😊😊

[joey]

Please can you do a video on Taylor series as I’m having problems with it….

[melisa]

F over g will not be continuous if g =0 ?