filmov
tv
Solving 2^x=x+(1/x), a Non-Standard Equation
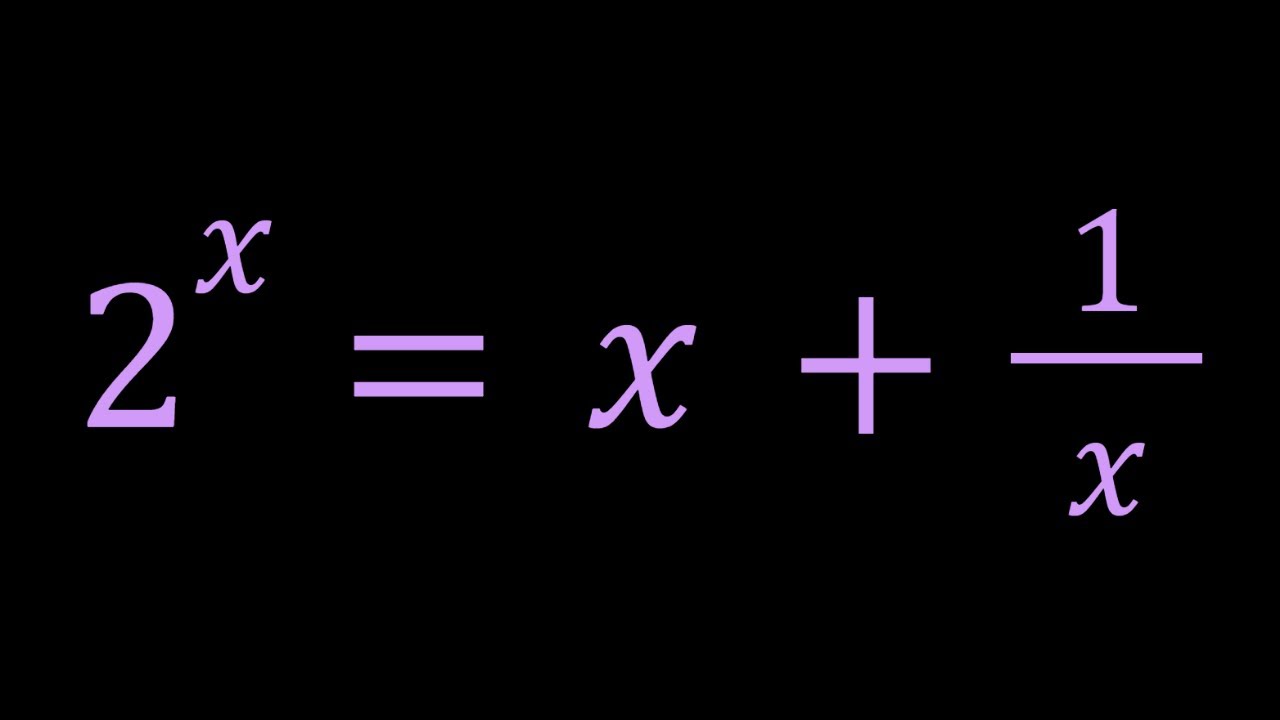
Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #NonStandardEquations
PLAYLISTS 🎵 :
#ChallengingMathProblems #NonStandardEquations
PLAYLISTS 🎵 :
Solving 2^x=x+(1/x), a Non-Standard Equation
Solving a Non-Standard Equation, 2^{x^2}=1-x^8
A Non-Standard Equation | ln(x)=1/2-x
Solving A Non-Standard Equation
Solving a Non-Standard Equation For Reals
A Non-Standard Equation With One Solution
Solving a Non-Standard Equation, 2^{x^2}=1-x^8 #ChallengingMathProblems #ExponentialEquations
A Non-Standard Exponential Equation from Russia
Project152 Week 22 Solutions
Solving An Interesting Non-Standard Equation
Solving a nonic equation using a non-standard method...An algebra challenge...
Solving a Non-standard System of Equations
Non-standard Exponential Equation! / How to solve in two ways?
Solving a Non-standard System of Equations in Two Ways
A Non-Standard Equation with One Solution (k is positive)
Finding f(0) When f(f(x))=x^2-x+1
Solving a non-standard equation
Solving a Non-Standard Equation
Solving an equation that is tricky by using restriction. 2^x+2^1/x=4
BEST Method to Solve this Non-Standard OLYMPIAD Exponential Equation | X=?
Let's Solve A Non-Standard Equation | Problem #112
A non-standard quartic equation
Solving a Non-standard System of Equations | Two Ways
Solving a Non-Standard Exponential Equation | x^x^2 + 6x + 8 = 1
Комментарии
 0:10:18
0:10:18
 0:07:27
0:07:27
 0:05:39
0:05:39
 0:09:05
0:09:05
 0:04:34
0:04:34
 0:09:22
0:09:22
 0:05:12
0:05:12
 0:09:53
0:09:53
 0:27:59
0:27:59
 0:06:31
0:06:31
 0:07:08
0:07:08
 0:10:17
0:10:17
 0:13:36
0:13:36
 0:12:45
0:12:45
 0:08:42
0:08:42
 0:08:35
0:08:35
 0:08:15
0:08:15
 0:00:48
0:00:48
 0:06:30
0:06:30
 0:10:03
0:10:03
 0:09:47
0:09:47
 0:06:09
0:06:09
 0:12:45
0:12:45
 0:08:18
0:08:18