filmov
tv
Conditional Probability For X given Y is less than 1 Provided we have the Joint PDF
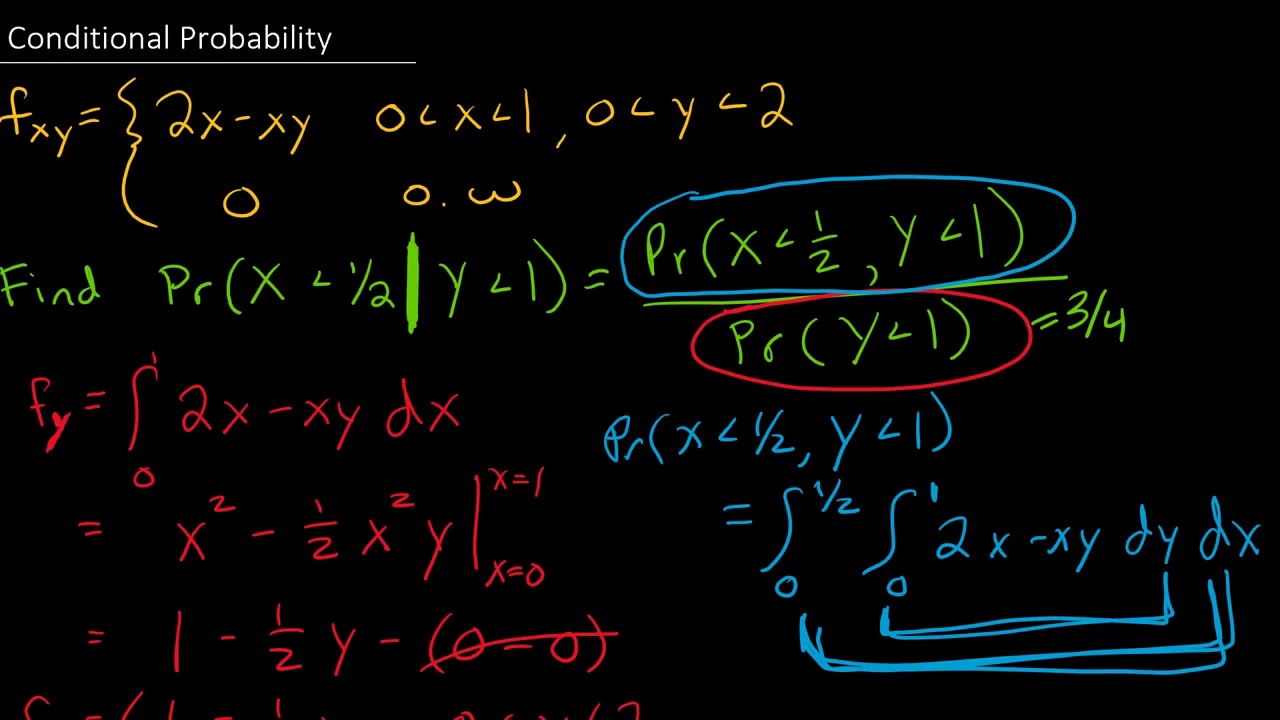
Показать описание
Find the conditional probability that X is less than 0.5 GIVEN that Y is less than 1.
Intro to Conditional Probability
Conditional Probability For X given Y is less than 1 Provided we have the Joint PDF
The Conditional Expectation of X given that Y=y
Conditional Probability given Joint PDF
Conditional expectation -- Example 1
Calculating conditional probability | Probability and Statistics | Khan Academy
L07.2 Conditional PMFs
Understanding Conditional Probabilities of X and Y | Probability Explained
Probability Density Function of Z=X+Y : Example 1
Conditional Probability - Example 1
Probability Formulas, Symbols & Notations - Marginal, Joint, & Conditional Probabilities
Conditional Probability With Venn Diagrams & Contingency Tables
Conditional Probability Distributions
Conditional Expectation of Random Variables: Example 1
Conditional Probability Density Functions
[Chapter 6] #8 Conditional distribution, the discrete case
Marginal distribution and conditional distribution | AP Statistics | Khan Academy
L06.4 Conditional PMFs & Expectations Given an Event
Conditional probability
Conditional probability density function
Conditional Probability | GCSE Maths 2025
[Chapter 7] #5 Conditional expectation
MA 381: Section 8.1: Joint Probability Density Functions
Examples of Conditional Expectation for Discrete and Continuous Case
Комментарии
 0:06:14
0:06:14
 0:07:51
0:07:51
 0:04:11
0:04:11
 0:12:02
0:12:02
 0:03:22
0:03:22
 0:06:43
0:06:43
 0:10:48
0:10:48
 0:10:09
0:10:09
 0:07:58
0:07:58
 0:08:12
0:08:12
 0:30:43
0:30:43
 0:16:26
0:16:26
 0:08:42
0:08:42
 0:08:34
0:08:34
 0:06:37
0:06:37
![[Chapter 6] #8](https://i.ytimg.com/vi/D7NhXkHwnEk/hqdefault.jpg) 0:13:37
0:13:37
 0:06:36
0:06:36
 0:07:31
0:07:31
 0:00:05
0:00:05
 0:05:14
0:05:14
 0:00:57
0:00:57
![[Chapter 7] #5](https://i.ytimg.com/vi/NM9L7sGpriI/hqdefault.jpg) 0:20:34
0:20:34
 0:09:52
0:09:52
 0:23:46
0:23:46