A Tricky Integral Problem with Solution by Substitution | Integral Calculus

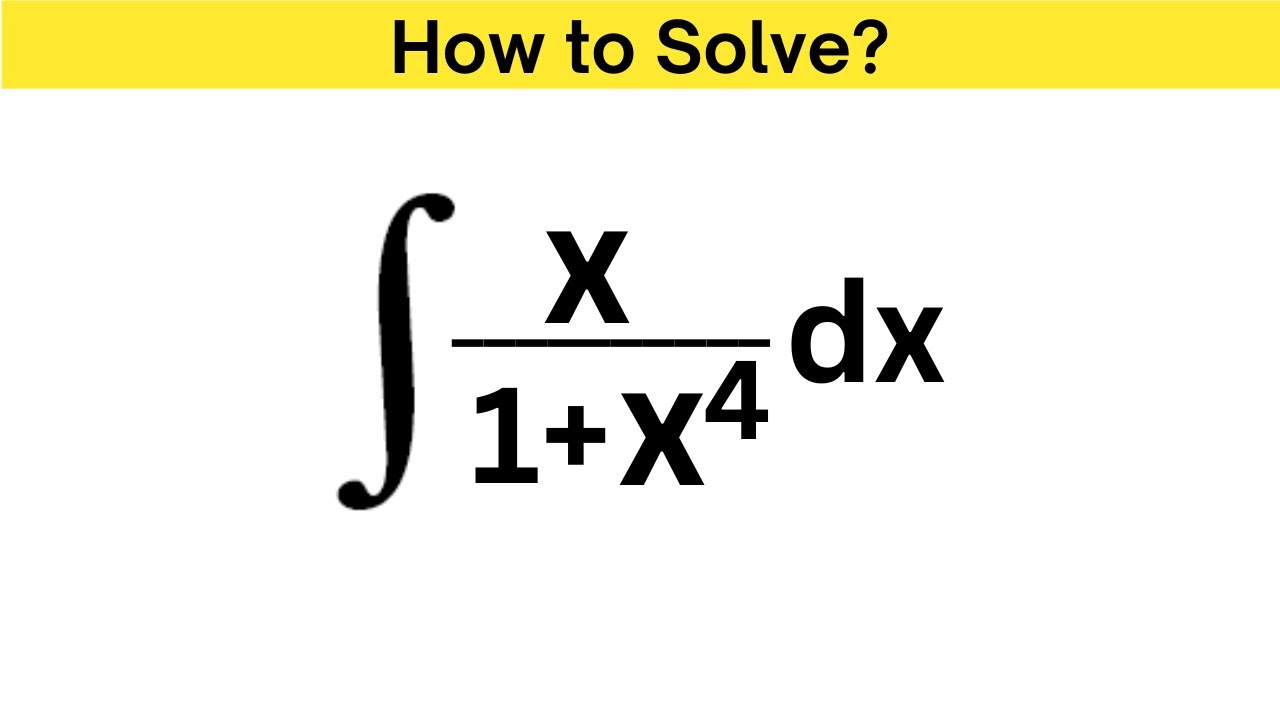
preview_player
Показать описание
Integration by substitution method

Tags
#OlympiadMathematics #OlympiadPreparation #CollegeEntranceExam #CompetitiveExams #jobexam
#數學 #数学 #算数 #Matemáticas #الرياضيات #matematica #matematika #Mathematik #수학#математика #mônToán #คณิตศาสตร์ #သင်္ချာ #अंकशास्त्र #கணிதம் #অংক #ಗಣಿತಶಾಸ್ತ್ರ
  1. Mathsiyan
  2. integration
  3. integral calculus
  4. mathematics
  5. exponential function
  6. integration of exponential functions
Рекомендации по теме
Another Tricky integral 0:03:42

Another Tricky integral | Daily integral problem-87 #calculus #integral

life changing integration 0:05:23

life changing integration by parts trick

a TRICKY integral 0:16:04

a TRICKY integral

Math Integration Timelapse 0:00:09

Math Integration Timelapse | Real-life Application of Calculus #math #maths #justicethetutor

Two Tricky Integration 0:08:35

Two Tricky Integration By Parts Examples

Change the order 0:07:39

Change the order of integration to solve tricky integrals

MIT Integration Bee 0:00:19

MIT Integration Bee Qualifier Solved In 30 Seconds

Integration Tricks (That 0:11:26

Integration Tricks (That Teachers Won't Tell You) for Integral Calculus

The Scenic Route 0:12:41

The Scenic Route To Solving 2+2 PART 5 #hyperspaceccalculus #integrationbysubstitution #maths

I Computed An 0:04:20

I Computed An Integral That Breaks Math

A tricky integral 0:10:08

A tricky integral from MIT

What Integration Technique 0:22:40

What Integration Technique Should I Use? (trig sub, u sub, DI method, partial fractions) calculus 2

Understand u substitution 0:14:41

Understand u substitution for integration (3 slightly trickier examples), calculus 1 tutorial

A Simple looking 0:08:28

A Simple looking but tricky rational integral.

🔥Seems difficult, but 0:00:47

🔥Seems difficult, but actually an EASY integral problem! #calculus #integration #math

One weird integral 0:01:00

One weird integral trick

INTEGRATION Shortcut Method 0:00:33

INTEGRATION Shortcut Method - Calculus Tricks : Trick to calculate Integration

Why I don't 0:14:54

Why I don't teach LIATE (integration by parts trick)

What is Integration? 0:10:55

What is Integration? 3 Ways to Interpret Integrals

A-Level Maths: H5-24 0:07:11

A-Level Maths: H5-24 Further Integration: Tricky Integration by Substitution Problems

Use of Integration 0:00:12

Use of Integration in REAL LIFE | why should we learn Integration? #integration #class12 #calculus

Tricky Trig/Integration Question 0:06:08

Tricky Trig/Integration Question (2 of 3)

Solve This Tricky 0:12:31

Solve This Tricky Integral to get Free Wi-Fi!

How To Master 0:00:59

How To Master Integration For JEE? | Overcome The Fear Of Integration For JEE #maths #integration

Комментарии
Автор

I found this integral relatively simple. I'm struggling with the same integral but with a 1 on top instead of the x.

miguelvieira
Автор

Hey Everyone!I 'm really new to calculus and had a doubt. Say we consider 1+x4=p so differentiating we get dp/dx as 4x^3 now we get dp as 4x^3 dx.
Let us cube the integral and multiply and divide by 4. We get the integral of dp/4p^3 by substitution and then what we do is we drag 1/4 out and integrate. So we get p^-2 / -2 and the integral comes out to be -1/8p^2. So what is the problem here?I did not even get tan in my soln?

sanatankaushik
Автор

After second step u can solve it by tan inverse (x²)*(1/2) +C

ahmednabil