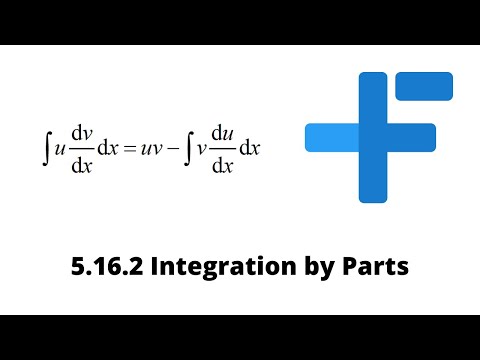
Two Tricky Integration By Parts Examples

We investigate two tricky integration by parts examples. In the first one we have to combine I.B.P with a u-substitution because perhaps the natural first guess doesn't work. In the second, we see what to do with I.B.P over and over again results in a cyclical pattern.

1) x^7 sqrt(1+x^4)
2) e^xsin(x)

****************************************************
YOUR TURN! Learning math requires more than just watching videos, so make sure you reflect, ask questions, and do lots of practice problems!
****************************************************

****************************************************
Other Course Playlists:

***************************************************

► Want to learn math effectively? Check out my "Learning Math" Series:

►Want some cool math? Check out my "Cool Math" Series:

*****************************************************

*****************************************************

*****************************************************

This video was created by Dr. Trefor Bazett, an Assistant Professor, Educator at the University of Cincinnati. #calculus #math

BECOME A MEMBER:

MATH BOOKS & MERCH I LOVE:

[TrinityTwo]

The mathematics department of Rutgers University can learn a thing about how to teach math from you.

[averydrago]

At 2:30 ish, I was able to use u-substitution by splitting x^7 into x^4 * x^3 (int is integral as in indefinite):
u = 1 + x^4
du = 4x^3 dx
x^4 = u - 1
Then you have :
1/4 int(( u - 1 )u^1/2 du)
Then I simply distributed the sqrt(u) to the ( u - 1 )
Found the antiderivatives
Substituted again

Note: I havent watched far into the video yet to know if you did this too

[johnouyang]

Some people ask why Integrating by parts vs. U-substitution gives me two different results,
The answer is, Yes of course, You can get different function as integrals of a single function.

And the magic behind this lies in Constant of Integration,
the results you get differ by some constant value.

Dr. Trefor, Thank you very much for this math channel, help me a LOT.

[sirig]

excellent! It took me a while for the logic to click in my head, but I'm blown away now :) Math can be so beautiful

[a.b]

6:22 you can use BPRP DI method here, maybe? I'll try it out.

[robertj]

Reverse thinking: (e^x cosx)' = e^x(cosx - sinx); (e^x sinx)' = e^x(sinx + cosx); integrate and then add two equations to get the answer directly.

[velocity]

I've read online that U-substitution is mostly used for a "chain-rule" type of problem and integration by parts is mostly used for two functions being multiplied together. For the first example, both are applicable, so how would you know which to use? Is integration really just trial and error or is there a definite way of knowing?

[WeaverLovesChrist]

Im super lost on your u-subs for the first example

[nightbot.]

At the end of problem 1 I got a different answer to u and used a deriverative calculator and got the correct expression

[Ragehunter]

the second problem is not really a matter but at first question i only did U-substitution cuz i realised x^7 is x^4 . x^3
so i did:
u = 1+x^4
du = 4x^3dx
and x^4= u-1
so i re-write it the equation: integral of x^4.x^3 . sqrt(1+x^4)dx by ---> integral of u-1 . sqrt(u). du/4

and after just Found the antiderivatives
Substituted again


i found

you can copy and past it to symbolab or somewhere to see what i write here

time for replacing u with 1+x^4



this is what i found but.. not same answer? i remember we can solve this problem in Calculus 1 with U-substitution but why is not same answer with the given answer in the video? can anyone tell me why is this not working or may working that i couldnt understand

[kennethsalido]

5:06 I'm a little bit confused.. what happen to 4x^3???
Tnxxx

[omkarumadi]

You forgot to integrate 4x^3 part at the end of problem 1

[IagobaApellaniz]

Wouldn't be better to do first the u substitution?

[holdenaronson]

at 7:14 why do your "v" values keep changing? yo have v= sinX) first and then the equation states that it is e^x. then for the next intregral you have v= -cos(x) and then in the equation state that is it -cos(x) AND e^x. why do the v-values keep changing?

[hpp]

At 8:08, where did the integration constant C come from? After all, we didn't evaluate any integral specifically.

[Spacexioms]

6:08 v should be sin(x) and du should be e^xdx?

[tradingchris]

Y all yt has different ways? It makes us more problematic 😵

[visweswararoach]

It's a nice example of integration by parts...the lecture is good and the teacher is familiar with the subject... congratulations to channel directors and the faculty for the good work done.

[peter]

Your answer a for the first question is so weird and it is totally different from my answer and symbolab's. Could have just substitute u=x^4 at the beginning.

[libelldrian]

Can't you just substitute x^4 with u after breaking up x^7 into x^4 and x^3?
du/dx is 4x^3, so dx is du/4x^3. This is enough to cancel all the x terms: $ u * x^3 * sqrt(1+u) du/4x^3 = 1/4 $ u * sqrt(1+u).