filmov
tv
Worked example: arc length | Applications of definite integrals | AP Calculus BC | Khan Academy

Показать описание
A finely tuned example demonstrating how the arc length formula works.
AP Calculus BC on Khan Academy: Learn AP Calculus BC - everything from AP Calculus AB plus a few extra goodies, such as Taylor series, to prepare you for the AP Test
For free. For everyone. Forever. #YouCanLearnAnything
AP Calculus BC on Khan Academy: Learn AP Calculus BC - everything from AP Calculus AB plus a few extra goodies, such as Taylor series, to prepare you for the AP Test
For free. For everyone. Forever. #YouCanLearnAnything
Worked example: arc length | Applications of definite integrals | AP Calculus BC | Khan Academy
Arc Length Calculus Problems,
Worked example: Parametric arc length | AP Calculus BC | Khan Academy
Another arc length integration example
Finding Arc Lengths | GCSE Maths 2025
Arc Length Calculus (Example 1)
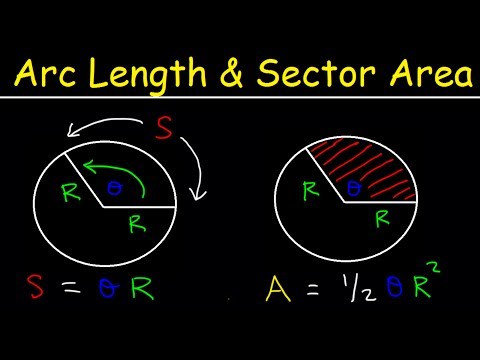
Arc Length of a Circle Formula - Sector Area, Examples, Radians, In Terms of Pi, Trigonometry
Arc Length of Parametric Curves
E&M Reading Group Ch. 9 Part 2, Arc Length in Polar Coordinates, Tangents to y=x^3 Through (4,0)
Arc Length Fast Example #Shorts
Arc Length Calculus (Example 3)
Arc Length (formula explained)
Arc length intro | Applications of definite integrals | AP Calculus BC | Khan Academy
Arc Length
Finding Arc Length | Calculus 2 | Math with Professor V
Arc Length Calculus (Example 2)
Arc Length Formula - Example 2
Arc Length
Arc length of vector functions example
Find The Arc Length | Geometry SAT Math Question Practice
Arc Length of a Parametric Curve, Another Example #2
Arc Length of a Parametric Curve, Another Example #1
Arc Length & Surface Area examples
Arc Length Along Parabola 1: Base Case
Комментарии
 0:07:03
0:07:03
 0:30:47
0:30:47
 0:04:48
0:04:48
 0:09:18
0:09:18
 0:00:54
0:00:54
 0:07:04
0:07:04
 0:15:57
0:15:57
 0:12:34
0:12:34
 5:41:01
5:41:01
 0:00:58
0:00:58
 0:08:19
0:08:19
 0:07:57
0:07:57
 0:06:21
0:06:21
 0:04:02
0:04:02
 0:16:50
0:16:50
 0:11:14
0:11:14
 0:03:19
0:03:19
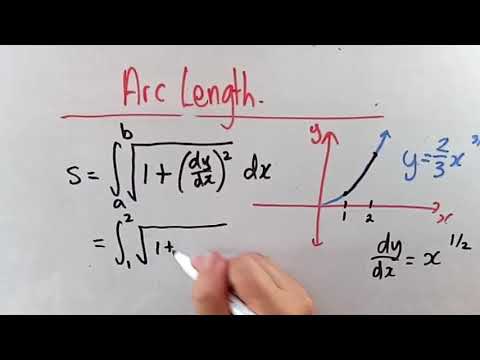 0:03:39
0:03:39
 0:04:27
0:04:27
 0:00:55
0:00:55
 0:05:23
0:05:23
 0:01:50
0:01:50
 0:17:35
0:17:35
 0:10:05
0:10:05