filmov
tv
Gravitational Potential is NOT mgh!!!

Показать описание
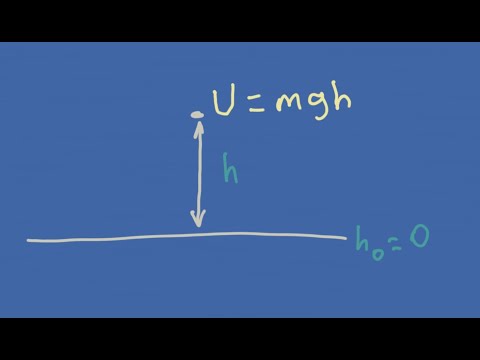
Here we derive and explore the true Gravitational Potential Energy of an object within a gravitational field. Gravitational Potential Energy (Ug , GPE or PEG , depending on who you ask) is only approximated as mgh when it in fact is not. So here we use Newton's Law of Universal Gravitation to along with the definition of Work to integrate to get the potential energy of an object.
It is important to point out that when using this potential energy function the potential is zero only at an infinite radius. All other radii have negative potentials.
Additionally it is important to point out, that for any object at a nonzero radius from another object, the potential energy of the object is finite. So even if you lift something infinitely high, you will only ever do so much work on that object. This leads to escape velocity, which we will explore in another video.
It is important to point out that when using this potential energy function the potential is zero only at an infinite radius. All other radii have negative potentials.
Additionally it is important to point out, that for any object at a nonzero radius from another object, the potential energy of the object is finite. So even if you lift something infinitely high, you will only ever do so much work on that object. This leads to escape velocity, which we will explore in another video.
Gravitational Potential is NOT mgh!!!
7.3.1 Why is gravitational potential energy always negative?
Calculating gravitational potential energy | Modeling energy | High school physics | Khan Academy
Gravitational Potential Energy Part 2 - Calculating Mass
How To Prove That Gravitational Potential Energy = mgh -- CIE A2 Physics (Exam Question Walkthrough)
Great science teacher risks his life explaining potential and kinetic energy
Connection between mgh and General Expression for Gravitational Potential Energy
Gravitational Potential Energy Formula mgh DERIVED
Class 9th EM SCIENCE Work and Energy
Gravitational Potential Energy Space
How to Derive Gravitational Potential Energy Quickly - A Level Physics
The General Gravitational Potential Energy Formula DERIVED
5.2.1 Gravitational Potential Energy: Why is GPE=mgh?
Error Using mgh - Intro to Physics
Gravitational potential energy at large distances | AP Physics 1 | Khan Academy
What is Gravitational Potential Energy #4
Work Done By Gravity and Gravitational Potential Energy - Physics
Is Gravitational Potential Energy 'mgh' - Saral Explanation
Gravitational Potential Energy | Physics
2.511 Proving that potential energy is mgh
Gravitational Potential Φ & Gravitational Potential Energy- Gravitational Fields, A Level Physic...
Gravitational Potential Energy & Work Done + Calculation Example // HSC Physics
√ The Gravitational Potential Energy Explained with Fair Examples
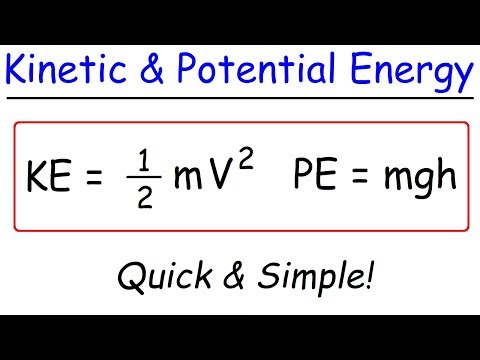
Kinetic Energy and Potential Energy
Комментарии
 0:14:58
0:14:58
 0:01:34
0:01:34
 0:04:33
0:04:33
 0:02:18
0:02:18
 0:04:11
0:04:11
 0:03:19
0:03:19
 0:09:31
0:09:31
 0:08:38
0:08:38
 0:19:58
0:19:58
 0:07:30
0:07:30
 0:01:27
0:01:27
 0:11:34
0:11:34
 0:03:56
0:03:56
 0:01:49
0:01:49
 0:05:04
0:05:04
 0:09:06
0:09:06
 0:12:47
0:12:47
 0:25:08
0:25:08
 0:06:52
0:06:52
 0:04:49
0:04:49
 0:12:30
0:12:30
 0:13:34
0:13:34
 0:21:53
0:21:53
 0:13:18
0:13:18