filmov
tv
Three unsolved problems in geometry

Показать описание
The Toeplitz conjecture and perfect cuboid problem are among easy-to-understand geometry problems that remain unsolved.
My other YouTube channels:
The Science Fiction Rock Experience ( the music show I produce):
My science and music channel::
Science World (with Emrah Polat):
My other YouTube channels:
The Science Fiction Rock Experience ( the music show I produce):
My science and music channel::
Science World (with Emrah Polat):
Three unsolved problems in geometry
The Three Square Geometry Problem - Numberphile
THREE UNSOLVED PROBLEMS OF ANCIENT GREECE I Innovative 3D presentation of these 3 classic problems
Every Unsolved Math Problems that Sounds Easy - Part 2
The hardest problem on the hardest test
George Dantzig, The Accidental Maths Genius (Good Will Hunting in Real Life)
Open Problems in Geometry
10 Unsolved Math Problems!!!
9/3/24 Regular Board Meeting
Can you solve this ALMOST IMPOSSIBLE third grade geometry puzzle?!
Monty Hall Problem - Numberphile
Evil Geometry Problem
Beautiful Geometry Problem Easily Solved
Classical problems of Greek geometry
5 = 3 + 4? The Staircase Paradox. Spot The Mistake 'Disproving' The Pythagorean Theorem
The biggest unsolved problem in Mathematics. Mathematics with Edward Frenkel
Millennium Problems: Math’s Million Dollar Bounties
A slacker was 20 minutes late and received two math problems… His solutions shocked his professor.
Do you know that many Math problems remain unsolved ? | Everything You Need To Know | Plop Info
Section 4- Three Famous Greek Problems
The paradox at the heart of mathematics: Gödel's Incompleteness Theorem - Marcus du Sautoy
Who cares about topology? (Inscribed rectangle problem)
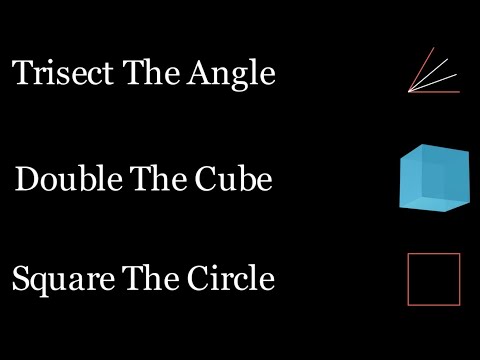
Impossible Geometry Problems: Trisecting Angle, Doubling Cube, Squaring Circle
The Simple Question that Stumped Everyone Except Marilyn vos Savant
Комментарии
 0:08:18
0:08:18
 0:12:21
0:12:21
 0:03:25
0:03:25
 0:12:43
0:12:43
 0:11:15
0:11:15
 0:01:49
0:01:49
 0:14:59
0:14:59
 0:04:23
0:04:23
 1:13:30
1:13:30
 0:15:24
0:15:24
 0:05:30
0:05:30
 0:03:23
0:03:23
 0:06:43
0:06:43
 0:08:54
0:08:54
 0:01:48
0:01:48
 0:02:12
0:02:12
 0:15:05
0:15:05
 0:07:13
0:07:13
 0:03:45
0:03:45
 0:11:53
0:11:53
 0:05:20
0:05:20
 0:18:16
0:18:16
 0:11:16
0:11:16
 0:07:06
0:07:06