Geometric average annual return

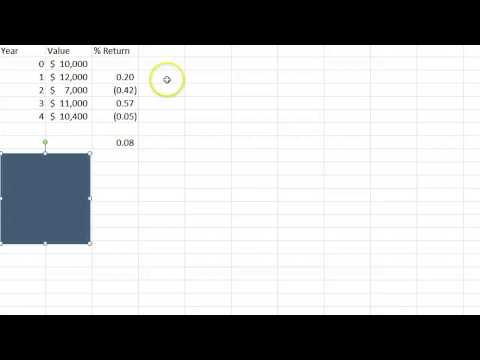
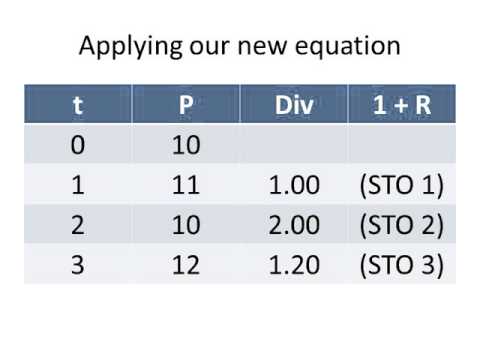
There are two ways one can calculate the Average Annual Return on, say, a stock investment: (1) the arithmetic average, and (2) the geometric average. While the arithmetic average is nothing but the "simple average", the geometric average is calculated very differently, and it also has a slightly different interpretation.

Watch this video to see how the Geometric Average Annual Return can be calculated using a FORMULA, as well as the trick to find it using a FINANCIAL CALCULATOR (I used "Texas Instruments BA ii plus" calculator).

Enjoy! :-)

[CPaulBreezy]

Yay! Had a problem starting with $80 initial investment, & $100 at the end of yr 1, $120 at the end of yr 2, $150 at the end of yr 3, & sold for $100 at end of yr 4. Instead of running through the whole Calc of 1+r.... I just plugged in the -$80 PV, $100 FV, and 4 N to get my I/yr. So much easier! Thank you! 👏🏽👏🏽👏🏽

[cjohnson]

I like the second method because it really shows the intuition behind geometric return and how its compounded

[lasereyes]

I have a question: If I have multiple Geometric Averages in a portfolio (multiple accounts in a portfolio), can you use the same calculation method to find the geometric average of the portfolio?

For example, let's say my portfolio has three accounts:

1) S&P 500 Index Fund, geometric average of 10%
2) Gold Investment, geometric average of 12%
3) Tesla Stock, geometric average of 15%

And, I want to find the geometric average of my portfolio. Would this calculation be correct:

[(1.10)x(1.12)x(1.15)]^1/3 -1.

Thank you.

[cathyjohnson]

Exceptional...thank you for posting this so clearly

[Tj-tcgp]

Thank you for your help I was able to work though a problem on my assignment using your example 👍