Algebra of physical space | Wikipedia audio article

This is an audio version of the Wikipedia Article:


00:01:15 1 Special relativity
00:01:25 1.1 Spacetime position paravector
00:02:37 1.2 Lorentz transformations and rotors
00:03:45 1.3 Four-velocity paravector
00:05:20 1.4 Four-momentum paravector
00:05:56 2 Classical electrodynamics
00:11:37 2.1 The electromagnetic field, potential and current
00:12:25 2.2 Maxwell's equations and the Lorentz force
00:12:35 2.3 Electromagnetic Lagrangian
00:17:41 3 Relativistic quantum mechanics
00:18:48 4 Classical spinor
00:19:37 5 See also
00:20:44 6 References
00:22:22 6.1 Textbooks



Listening is a more natural way of learning, when compared to reading. Written language only began at around 3200 BC, but spoken language has existed long ago.

Learning by listening is a great way to:
- increases imagination and understanding
- improves your listening skills
- improves your own spoken accent
- learn while on the move
- reduce eye strain

Now learn the vast amount of general knowledge available on Wikipedia through audio (audio article). You could even learn subconsciously by playing the audio while you are sleeping! If you are planning to listen a lot, you could try using a bone conduction headphone, or a standard speaker instead of an earphone.

Listen on Google Assistant through Extra Audio:
Other Wikipedia audio articles at:
Upload your own Wikipedia articles through:
Speaking Rate: 0.8089983275479954
Voice name: en-US-Wavenet-C


"I cannot teach anybody anything, I can only make them think."
- Socrates


SUMMARY
=======
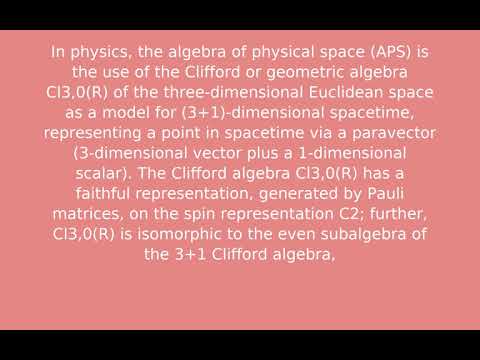
In physics, the algebra of physical space (APS) is the use of the Clifford or geometric algebra Cl3,0(R) of the three-dimensional Euclidean space as a model for (3+1)-dimensional spacetime, representing a point in spacetime via a paravector (3-dimensional vector plus a 1-dimensional scalar).
The Clifford algebra Cl3,0(R) has a faithful representation, generated by Pauli matrices, on the spin representation C2; further, Cl3,0(R) is isomorphic to the even subalgebra of the 3+1 Clifford algebra, Cl[0]3,1(R).
APS can be used to construct a compact, unified and geometrical formalism for both classical and quantum mechanics.
APS should not be confused with spacetime algebra (STA), which concerns the Clifford algebra Cl1,3(R) of the four-dimensional Minkowski spacetime.