filmov
tv
Edmentum Algebra1 Unit5 Systems of Linear Equations
Показать описание
00:00 Tutorial
27:24 Practice
59:46 Mastery Test
Which system of equations is satisfied by the solution shown in the graph?
x + 2y = 6 and x − y = 10
x + y = 6 and x − 2y = 10
x + 2y = 10 and x − y = 6
x + y = 6 and x − y = -10
Which set of coordinates satisfies the equations 3x − 2y = 15 and 4x − y = 20?
The graphed system represents the rate at which two trucks are being filled, where x represents the time in minutes and y represents the percentage filled.
Which statement is true?
The solution is nonviable because the percentage filled cannot be negative.
The solution is nonviable because the time cannot be negative.
The solution is nonviable because the time cannot be partial minutes.
The solution is viable.
This system of equations is shown on the graph:
2y − 4x = 6
y = 2x + 3
The system has no solution.
The system has a unique solution at (0,3).
The system has a unique solution at (1,5).
The system has infinitely many solutions.
A company sells two versions of an antivirus software. The home edition costs $23.50, and the business edition costs $58.75. Last week, the company earned $29,668.75 from selling 745 copies of the software.
If x represents the number of copies of the home edition sold and y represents the number of copies of the business edition sold, which system of equations represents this situation?
Determine which situation could be represented by the system of linear equations given below.
An audience contains 210 people. Student tickets cost $3 each and adult tickets cost $5 each. At one performance, there are 60 more adults than students.
A guitar requires 5 strings and a banjo requires 3 strings. An orchestra has a total of 210 strings. One guitar player and one banjo player have 60 strings.
A candy store sells boxes of chocolates for $5 each and boxes of caramels for $3 each. In one afternoon, the store sold 210 boxes of candy and made a profit of $60.
An art teacher bought paintbrushes in packs of 5 and packs of 3. She bought a total of 60 packs and now has 210 paintbrushes.
If the graphs of the linear equations in a system are parallel, what does that mean about the possible solution(s) of the system?
The lines in a system cannot be parallel.
There is no solution.
There is exactly one solution.
There are infinitely many solutions.
The concession stand at the high school football stadium sells hot dogs and hamburgers to raise money for the high school athletic programs. Each hot dog sold earns the programs $0.50, and each hamburger sold earns $0.75. This week, the concession stand sold a combination of 230 hot dogs and hamburgers and earned $138.50 for the athletic programs. If x represents the number of hot dogs sold and y represents the number of hamburgers sold, which system of equations represents this situation?
Amanda is placing an order for running shoes and leather boots for her footwear boutique. She needs a total of 48 pairs of shoes and twice as many pairs of running shoes as leather boots.
Set up the two equations that can be used to find the number of each type of shoe that Amanda needs to order.
Let the equation that represents the total number of pairs of shoes be referred to as constraint 1.
Let constraint 2 refer to the equation that describes the ratio of the number of running shoes to leather boots.
Ms. Rodriguez purchased markers to use on her class whiteboard. She bought a total of 16 markers and three times as many blue markers as red markers.
Set up the two equations that can be used to find the number of markers of each color that Ms. Rodriguez purchased.
Let constraint 1 refer to the equation that represents the total number of markers.
Let constraint 2 refer to the equation that describes the ratio of the number of blue markers to red markers.
A local museum charges $25 per adult and $12 per child for admission fees. At the end of a day, the museum made $9,014 in total admission revenue, not including sales tax, and had a total of 450 guests.
The system of equations below can be used to model the number of guests that were children, x, and the number of guests that were adults, y.
Then, determine the approximate number of guests that were children and the approximate number of guests that were adults for that day.
Suppose you have a pocketful of change. You have some pennies (p) and some quarters (q). One expression could be used to describe the total number of coins in your pocket:
p + q.
A second expression could be used to describe the amount of money (in dollars) in your pocket:
0.01p + 0.25q.
Evaluate each expression for the situation where you have 6 quarters and 7 pennies in your pocket.
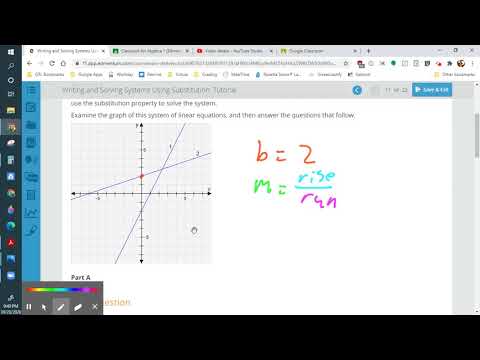
The lines in the graph of part A don’t intersect at two whole numbers on the coordinate grid. Estimate the values of the solution to the nearest one-fourth of a unit. Then substitute the x- and y-values of the approximate solution into the two equations. How close do you get to the constant numbers in each equation?
27:24 Practice
59:46 Mastery Test
Which system of equations is satisfied by the solution shown in the graph?
x + 2y = 6 and x − y = 10
x + y = 6 and x − 2y = 10
x + 2y = 10 and x − y = 6
x + y = 6 and x − y = -10
Which set of coordinates satisfies the equations 3x − 2y = 15 and 4x − y = 20?
The graphed system represents the rate at which two trucks are being filled, where x represents the time in minutes and y represents the percentage filled.
Which statement is true?
The solution is nonviable because the percentage filled cannot be negative.
The solution is nonviable because the time cannot be negative.
The solution is nonviable because the time cannot be partial minutes.
The solution is viable.
This system of equations is shown on the graph:
2y − 4x = 6
y = 2x + 3
The system has no solution.
The system has a unique solution at (0,3).
The system has a unique solution at (1,5).
The system has infinitely many solutions.
A company sells two versions of an antivirus software. The home edition costs $23.50, and the business edition costs $58.75. Last week, the company earned $29,668.75 from selling 745 copies of the software.
If x represents the number of copies of the home edition sold and y represents the number of copies of the business edition sold, which system of equations represents this situation?
Determine which situation could be represented by the system of linear equations given below.
An audience contains 210 people. Student tickets cost $3 each and adult tickets cost $5 each. At one performance, there are 60 more adults than students.
A guitar requires 5 strings and a banjo requires 3 strings. An orchestra has a total of 210 strings. One guitar player and one banjo player have 60 strings.
A candy store sells boxes of chocolates for $5 each and boxes of caramels for $3 each. In one afternoon, the store sold 210 boxes of candy and made a profit of $60.
An art teacher bought paintbrushes in packs of 5 and packs of 3. She bought a total of 60 packs and now has 210 paintbrushes.
If the graphs of the linear equations in a system are parallel, what does that mean about the possible solution(s) of the system?
The lines in a system cannot be parallel.
There is no solution.
There is exactly one solution.
There are infinitely many solutions.
The concession stand at the high school football stadium sells hot dogs and hamburgers to raise money for the high school athletic programs. Each hot dog sold earns the programs $0.50, and each hamburger sold earns $0.75. This week, the concession stand sold a combination of 230 hot dogs and hamburgers and earned $138.50 for the athletic programs. If x represents the number of hot dogs sold and y represents the number of hamburgers sold, which system of equations represents this situation?
Amanda is placing an order for running shoes and leather boots for her footwear boutique. She needs a total of 48 pairs of shoes and twice as many pairs of running shoes as leather boots.
Set up the two equations that can be used to find the number of each type of shoe that Amanda needs to order.
Let the equation that represents the total number of pairs of shoes be referred to as constraint 1.
Let constraint 2 refer to the equation that describes the ratio of the number of running shoes to leather boots.
Ms. Rodriguez purchased markers to use on her class whiteboard. She bought a total of 16 markers and three times as many blue markers as red markers.
Set up the two equations that can be used to find the number of markers of each color that Ms. Rodriguez purchased.
Let constraint 1 refer to the equation that represents the total number of markers.
Let constraint 2 refer to the equation that describes the ratio of the number of blue markers to red markers.
A local museum charges $25 per adult and $12 per child for admission fees. At the end of a day, the museum made $9,014 in total admission revenue, not including sales tax, and had a total of 450 guests.
The system of equations below can be used to model the number of guests that were children, x, and the number of guests that were adults, y.
Then, determine the approximate number of guests that were children and the approximate number of guests that were adults for that day.
Suppose you have a pocketful of change. You have some pennies (p) and some quarters (q). One expression could be used to describe the total number of coins in your pocket:
p + q.
A second expression could be used to describe the amount of money (in dollars) in your pocket:
0.01p + 0.25q.
Evaluate each expression for the situation where you have 6 quarters and 7 pennies in your pocket.
The lines in the graph of part A don’t intersect at two whole numbers on the coordinate grid. Estimate the values of the solution to the nearest one-fourth of a unit. Then substitute the x- and y-values of the approximate solution into the two equations. How close do you get to the constant numbers in each equation?
 1:26:41
1:26:41
 1:45:07
1:45:07
 0:19:10
0:19:10
 1:47:28
1:47:28
 0:36:16
0:36:16
 0:45:10
0:45:10
 0:32:29
0:32:29
 0:32:29
0:32:29
 0:12:12
0:12:12
 0:05:00
0:05:00
 1:27:23
1:27:23
 0:24:25
0:24:25
 1:24:16
1:24:16
 0:42:40
0:42:40
 1:45:07
1:45:07
 0:46:32
0:46:32
 0:21:20
0:21:20
 0:48:11
0:48:11
 0:22:28
0:22:28
 0:00:58
0:00:58
 0:07:27
0:07:27
 0:21:53
0:21:53
 0:00:27
0:00:27