filmov
tv
Pauli Matrices -- Properties

Показать описание
Some Useful Properties of the Pauli Matrices
- anticommutation relations
- commutation relations
- determinant and trace
- inverse
- hermiticity
[ review of summation, Kroneker delta, Levi-Civita symbol, etc. ]
--
Prof. Dr. Ricardo Gomes (IF - UFG)
- anticommutation relations
- commutation relations
- determinant and trace
- inverse
- hermiticity
[ review of summation, Kroneker delta, Levi-Civita symbol, etc. ]
--
Prof. Dr. Ricardo Gomes (IF - UFG)
Pauli Matrices -- Properties
The Pauli matrices
Algebra of the Pauli matrices
Pauli Spin Matrices and their properties | Commutation relations | NNN | QM
Spinors for Beginners 6: Pauli Vectors and Pauli Matrices
Pauli Matrices in Quantum Mechanics, Orthonormal Basis for 2D Hilbert Space, Trace and Determinant
PAULI SPIN MATRICES || PROPERTIES OF PAULI SPIN MATRICES || Commutation relations || CSIR NET||HINDI
All you need to know about Pauli matrices
D2B Pauli matrices.
Pauli matrices are involutory matrices (describe spin particles) #maths #physics
You've Heard of SPIN - But How Is it Encoded in the Math of Quantum Physics? Parth G
L4-4 Pauli Matrices Part I
Spinors for Beginners 8: Are the Pauli Matrices also Vectors? (Intro to Spinor Spaces)
L5-1 Pauli Matrices and Inner Product of Matrices
Pauli Matrices in brief||#Physics#Shorts
QM 4.11 Pauli spin matrices and their properties
PROPERTIES of PAULI MATRICES - Tutorial series on Spin [Part 8]
Spinning Around The Pauli Matrices! [Analyzing Tensor Products]
#QuantumMechanics #PauliMatrices #QuantumSpin #SigmaX #SigmaY #SigmaZ #QuantumComputing
Pauli-Matrizen und Spin-Operatoren
PAULI SPIN MATRICES AND PROPERTIES
Commutation RelationOf Pauli's Spin Matrices
Quantum Spin (2) - Pauli Matrices
Properties of Pauli matrices #maths #physics #quantumphysics #shorts
Комментарии
 0:16:18
0:16:18
 0:16:58
0:16:58
 0:01:22
0:01:22
 0:31:22
0:31:22
 0:26:35
0:26:35
 0:29:55
0:29:55
 0:17:32
0:17:32
 0:09:39
0:09:39
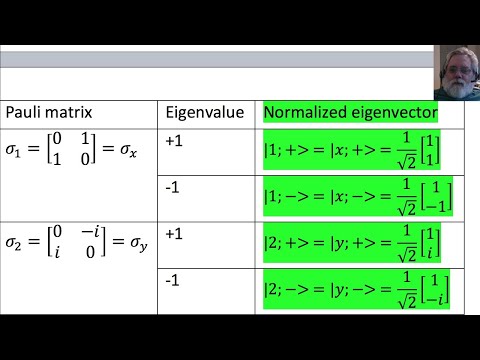 0:21:48
0:21:48
 0:00:11
0:00:11
 0:11:34
0:11:34
 0:08:28
0:08:28
 0:24:08
0:24:08
 0:43:24
0:43:24
 0:00:54
0:00:54
 0:14:01
0:14:01
 0:11:19
0:11:19
 0:06:08
0:06:08
 0:00:51
0:00:51
 0:18:31
0:18:31
 0:00:20
0:00:20
 0:00:11
0:00:11
 1:08:49
1:08:49
 0:00:11
0:00:11