filmov
tv
FAST eigenvalues
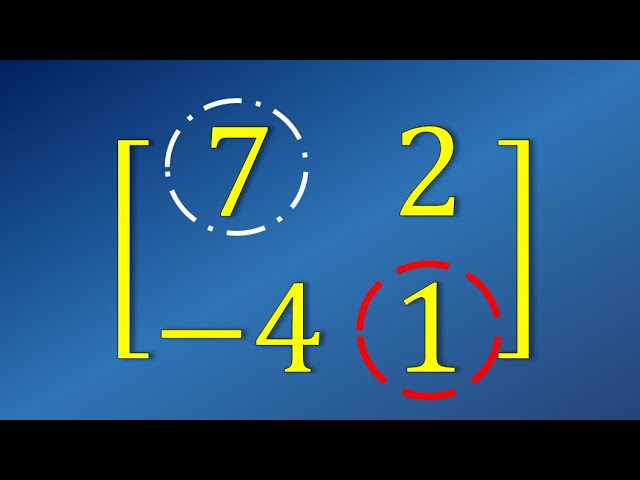
Показать описание
Are you tired of finding eigenvalues? Then check out this video for a 2-minute way of finding eigenvalues of any 2x2 matrix! This is based on a beautiful quadratic trick introduced by Po-Shen Loh at Carnegie Mellon University, but known since Babylonian times.
A quick trick for computing eigenvalues | Chapter 15, Essence of linear algebra
FAST eigenvalues
Eigenvalues (3x3) | fast and easy
Eigenvalues (2x2) | fast and easy
Linear Algebra 17e: Easy Eigenvalues - The Trace
Shortcut Trick to Find EigenValues of 3x3 Matrix
Find eigenvalues of 2x2 matrix - FAST and EASY!
How to find the eigenvector of a 3x3 matrix | Math with Janine
The applications of eigenvectors and eigenvalues | That thing you heard in Endgame has other uses
Find Eigenvalues of a 2x2 Matrix Fast!
Shortcut for Eigen Vectors of Matrix | Eigen Vector Engineering Math | Eigen Vector for GATE 2024
Fastest Way to Find Eigenvalues #maths #linearalgebra
Stability and Eigenvalues: What does it mean to be a 'stable' eigenvalue?
(Lecture 1) Quick review of eigenvalues, eigenvectors, and diagonalization
Real life example of Eigen values and Eigen vectors
Math Subject GRE: Eigenvalues Quickly!
Ch8 Pr5: Finding eigenvalues and eigenvectors
How to find determinant of 3×3 matrix ? Using calculator #engineeringmathematics #m4 #inhindi
18 Computing Eigenvectors and Eigenvalues
Eigenvalues & The Fourier Transform - Crossing the Bridge
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
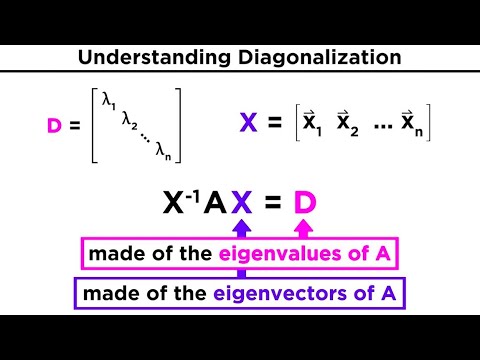
Diagonalization
12. Computing Eigenvalues and Singular Values
Dear linear algebra students, This is what matrices (and matrix manipulation) really look like
Комментарии
 0:13:13
0:13:13
 0:02:08
0:02:08
 0:17:36
0:17:36
 0:14:56
0:14:56
 0:04:23
0:04:23
 0:06:29
0:06:29
 0:09:07
0:09:07
 0:03:56
0:03:56
 0:23:45
0:23:45
 0:05:59
0:05:59
 0:05:37
0:05:37
 0:00:53
0:00:53
 0:14:53
0:14:53
 0:51:37
0:51:37
 0:04:44
0:04:44
 0:02:42
0:02:42
 0:05:45
0:05:45
 0:01:00
0:01:00
 0:16:58
0:16:58
 0:12:44
0:12:44
 0:00:16
0:00:16
 0:08:43
0:08:43
 0:49:28
0:49:28
 0:16:26
0:16:26