filmov
tv
Find All Possible Values - a Problem of Divisors | Number Theory | Viewer Suggested Problem

Показать описание
This is a nice number theory problem sent in by a viewer.
b, c and d are the three smallest positive divisors of n, not equal to 1.
Also, n = b⁴ + c³ + d² + 9.
Can you find all possible values of n?
Did you solve it?
Let me know in the comments how you solved this problem. I’d love to hear about it!
Test your friends and family to see if they're as quick as you.
Challenge yourself to fun and interesting maths problems and start your journey as an olympiad.
Maths videos by Giuliano Grasso - mathematics graduate from the University of East Anglia.
I post weekly maths olympiad videos for you to try and solve.
If you want to see more problems or send me your own, then subscribe here:
b, c and d are the three smallest positive divisors of n, not equal to 1.
Also, n = b⁴ + c³ + d² + 9.
Can you find all possible values of n?
Did you solve it?
Let me know in the comments how you solved this problem. I’d love to hear about it!
Test your friends and family to see if they're as quick as you.
Challenge yourself to fun and interesting maths problems and start your journey as an olympiad.
Maths videos by Giuliano Grasso - mathematics graduate from the University of East Anglia.
I post weekly maths olympiad videos for you to try and solve.
If you want to see more problems or send me your own, then subscribe here:
Find the sum of all possible values of x² + y²
Find All Possible Values - a Problem of Divisors | Number Theory | Viewer Suggested Problem
Find all possible values of X | Triangle Inequality Theorem | Important math skills explained
Find all possible values of X | Solving Inequalities | Important math skills explained
15 Possible values for expression
Find All Possible Values of M | Azerbaijani Olympiad | Number Theory | Math Olympiad Training
16 Possible values for t
Find all possible values of x in this nice exponential function question
Day 2 - Bi-Annual Planning and Work Session- State Board of Education - Dec. 4, 2024
Identify the set of possible values for each random variable.
Find the values a and b that make the piecewise function continuous
13 Possible values for x
Find all possible values of coefficient for Factorable Trinomial
Find all possible values of θ, where 0^∘ ≤θ≤360^∘. cosθ=-…
Russian l Very Nice Olympiad Math Problem l Find all possible values of k?
Find The Values of n | Number Theory | Math Problem Solving
Find all possible values of θ, where 0^∘ ≤θ≤360^∘. cosθ=-1
Finding All Values of a Such That There Are No x- and y-Intercepts for f(x) = 2| x + 1| + a
Find all possible values of x for which the number 7x3 is divisible by 3 Also find each such number
Find all the possible values of x for similar triangles
Finding all possible values of the inverse sine of a given value
For each of the following, find all possible values of the scalar αthat make the matrix defec…
Find Possible Values of X & Y | A Nice Algebraic Problem
Find all possible values of (a) L,(b) L_2, and (c) θfor a hydrog…
Комментарии
 0:13:47
0:13:47
 0:03:31
0:03:31
 0:08:28
0:08:28
 0:03:41
0:03:41
 0:05:40
0:05:40
 0:03:31
0:03:31
 0:04:33
0:04:33
 0:01:19
0:01:19
 5:46:00
5:46:00
 0:05:20
0:05:20
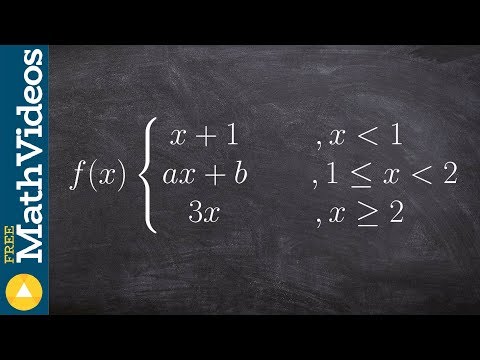 0:03:44
0:03:44
 0:02:32
0:02:32
 0:04:52
0:04:52
 0:00:33
0:00:33
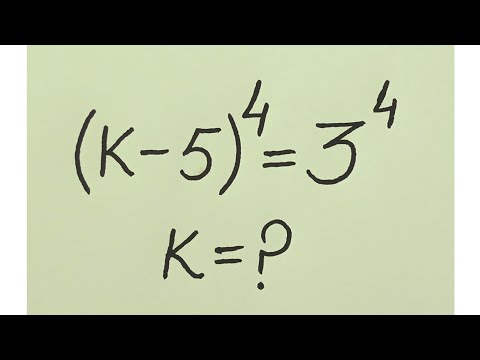 0:09:08
0:09:08
 0:04:54
0:04:54
 0:00:33
0:00:33
 0:10:20
0:10:20
 0:05:03
0:05:03
 0:06:58
0:06:58
 0:03:50
0:03:50
 0:00:33
0:00:33
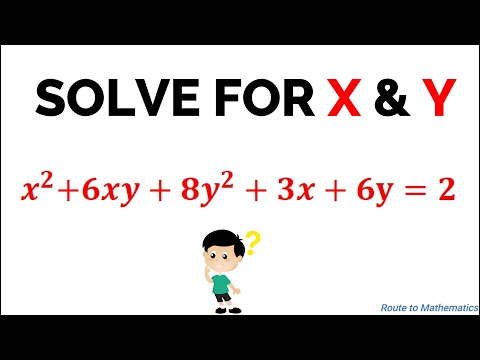 0:07:55
0:07:55
 0:00:33
0:00:33