filmov
tv
Ax=b Vs Ax=0, consistent & inconsistent, trivial & nontrivial
Показать описание
❖ The difference between Ax=b Vs Ax=0 and knowing about consistent & inconsistent systems and trivial & nontrivial solutions.
Ax=b is called a Nonhomogeneous system and has a consistent or inconsistent system.
Ax=0 is called a Homogeneous system and has a trivial or nontrivial solution.
❖ A linear system is called Non-homogeneous (Ax=b) if the right-hand side is a non-zero vector.
Ax=b has three possible solutions:
(1) the system has a unique (only one) solution.
(2) the system has more than one solution.
(3) the system has no solution at all.
Note:
(*) A linear system is called Consistent if there is at least one solution.
(**) A linear system is called Inconsistent if there is no solution.
❖ A linear system is called Homogeneous (Ax=0) if the right-hand side is a zero vector.
Ax=0 has two possible solutions:
(1) The system has A unique solution (only one solution) called a Trivial solution.
(2) The system has infinitely many solutions (more than one solution) called Nontrivial solutions.
The link to this playlist (Linear Algebra):
My Website:
Subscribe to My Channel to check out more videos:
Ax=b is called a Nonhomogeneous system and has a consistent or inconsistent system.
Ax=0 is called a Homogeneous system and has a trivial or nontrivial solution.
❖ A linear system is called Non-homogeneous (Ax=b) if the right-hand side is a non-zero vector.
Ax=b has three possible solutions:
(1) the system has a unique (only one) solution.
(2) the system has more than one solution.
(3) the system has no solution at all.
Note:
(*) A linear system is called Consistent if there is at least one solution.
(**) A linear system is called Inconsistent if there is no solution.
❖ A linear system is called Homogeneous (Ax=0) if the right-hand side is a zero vector.
Ax=0 has two possible solutions:
(1) The system has A unique solution (only one solution) called a Trivial solution.
(2) The system has infinitely many solutions (more than one solution) called Nontrivial solutions.
The link to this playlist (Linear Algebra):
My Website:
Subscribe to My Channel to check out more videos:
0:03:43
Ax=b Vs Ax=0, consistent & inconsistent, trivial & nontrivial
0:06:21
Geometric View on Solutions to Ax=b and Ax=0.
0:03:03
Ax = 0, the Homogenous Solution
0:17:05
Linear Algebra 1.5.1 Homogeneous System Solutions
0:06:05
Necessary and sufficient condition for a non-homogeneous system AX=B has a solution or consistent.
0:05:10
Homogeneous Systems: Given a Coefficient Matrix, Solve Ax=0
0:08:13
CONSISTENT AND INCONSISTENT SYSTEM (AX = b)
0:09:04
Solving Ax=b
0:10:04
Solving Ax=0
0:05:15
Linear equations - Importance of Ax = 0
0:11:11
Linear Algebra 1.4.1 The Matrix Equation Ax=b
0:13:08
A unique solution, No solution, or Infinitely many solutions | Ax=b
0:07:06
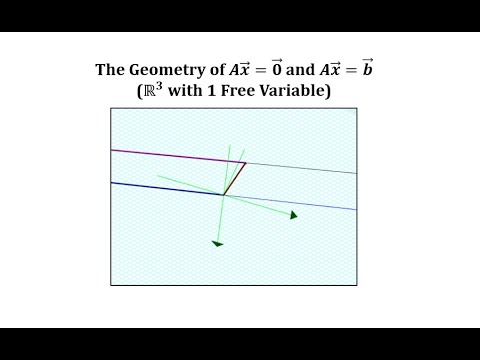
The Geometry of the Solutions to Ax=0 and Ax=b in R3 with 1 Free Variables
0:00:22
Linear Algebra- True or False? Ax = 0 has only the trivial solution iff
0:09:47
Linear Algebra | S01E08 System of Equations, Ax=0 & Ax=b | B.A/M.A Economics Entrance ISI/DSE
0:08:28
Examples with Ax=b Matrix Equations
0:30:14
Homogeneous and Nonhomogeneous Solutions: Ax=0 and Ax=b
0:06:57
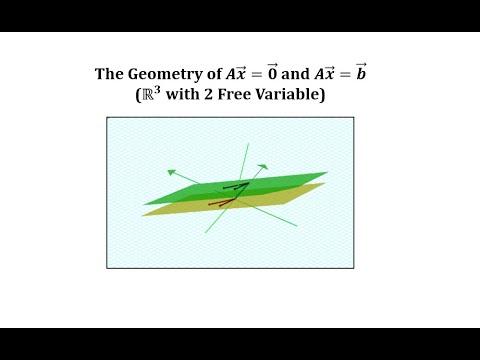
The Geometry of the Solutions to Ax=0 and Ax=b in R3 with 2 Free Variables
0:05:59
Solving a System Using the Matrix Equation, AX=B, Example
0:04:48
Writing solutions to Ax=b in vector form
0:05:20
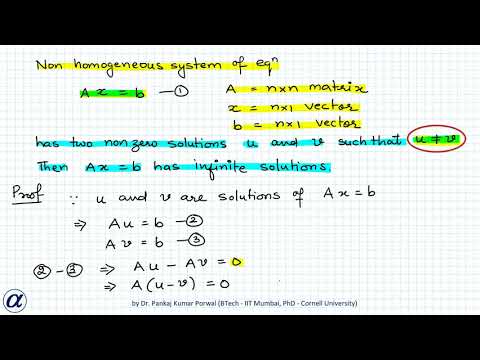
Ax = b has two distinct nonzero solutions
0:02:36
A quick video on solving Ax=b.
0:20:55
Consistency Theorem: the system of equations AX=B is consistent if the coefficient matrix A and ...
0:01:40
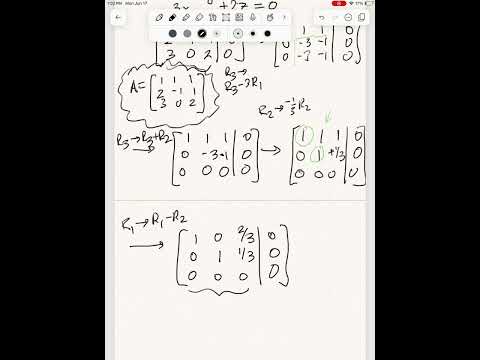
Find solution to Ax = 0 when given solution to Ax = b
Комментарии