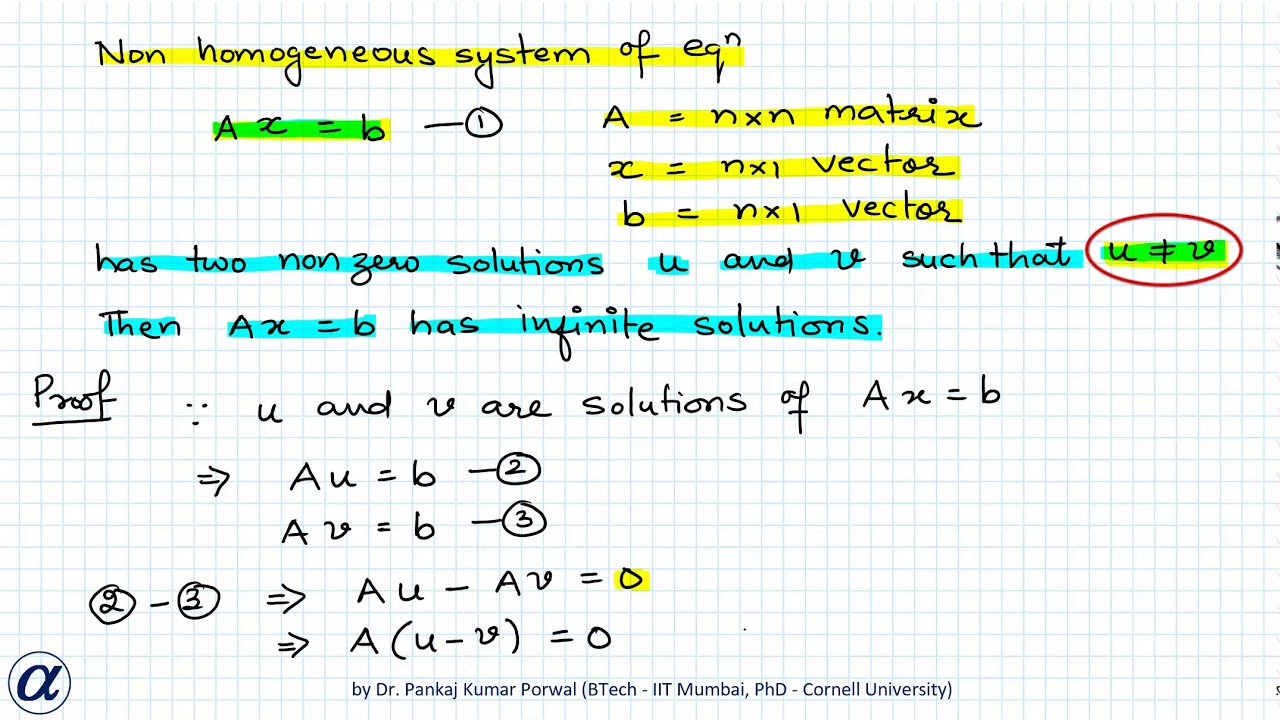
Ax = b has two distinct nonzero solutions

System of Linear Equations ( Number of unknowns is equal to number of equations )

A x = b is matrix form of system of linear equations. A is a matrix ( n x n ), x and b are vectors ( n x 1 ).
The system is called non-homogeneous if b is not equal to zero.
The system is called homogeneous if b is equal to zero ( b = 0 ).

Consistent system of equations : A system of equation is said to be consistent if there exist a solution satisfying the system of equations. For a consistent system of equations, we can either have a unique solution or infinite number of solutions. We can not have more than one but finite number of solutions. If we can somehow show (or we are given) that more than one distinct solution exists for a system of equations then there has to be infinite solutions. In particular if we can show that there are two distinct solutions to a system of linear equations then there will be infinitely many solutions.

Inconsistent system of equations : A system of equation is said to be inconsistent if no solution exist.

Non homogeneous system of equations

(i) Consistent and has a unique solution if : |A| not equal to zero ( determinant of A not equal to zero )
(ii) Consistent and has infinite solutions if : |A| equal to zero ( determinant of A equal to zero ) and adj(A)b equal to zero ( adjoint of A into b is zero )
(iii) Inconsistent : |A| equal to zero ( determinant of A equal to zero ) and adj(A)b is not equal to zero ( adjoint of A into b is not equal to zero )

Homogeneous system of equations

A homogeneous system of equation is always consistent
(i) Consistent and has a unique solution if |A| not equal to zero ( determinant of A is not equal to zero ). The solution is zero vector. This solution is also called trivial solution.
(ii) Consistent and has infinite number of solution if |A| is equal to zero ( determinant of A is equal to zero ). These solutions are also called non trivial solutions.

What is nonhomogeneous system of equations ?
What is homogeneous system of equations ?
What is the condition of existence of unique solution for nonhomogeneous system of equations ?
When does a nonhomogeneous system of equations have unique solution ?
When does a nonhomogeneous system of equations have infinite solution ?
When does a nonhomogeneous system of equations does not have a solution ?
When does a nonhomogeneous system of equations admit infinite solutions ?
What is trivial solution ?
When does a homogeneous system of equation has trivial solution ?
What is non trivial solution ?
When does a homogenous system of equation has nontrivial solution ?
When does a homogeneous system of equations have unique solution ?
When does a homogeneous system of equations have infinite solution ?

This video is uploaded by
Alpha Academy, Udaipur
Minakshi Porwal (9460189461)