filmov
tv
Ax = 0, the Homogenous Solution

Показать описание
In this video I talk about the linear algebra topic Ax = 0, also known as the Homogenous Solution. This is a concept of solving systems of linear equations. The idea of a homogeneous solution is important in linear algebra because it allows us to classify systems of linear equations based on the number and type of solutions they have. For example, a system of linear equations that has no solutions is called inconsistent, while a system of linear equations that has a unique solution is called consistent and independent. A system of linear equations that has infinitely many solutions is called consistent and dependent. In summary, the concept of a homogeneous solution is important in linear algebra because it allows us to classify systems of linear equations based on the number and type of solutions they have. It is also closely related to other important concepts in linear algebra, such as the null space of a matrix and the rank-nullity theorem.
#Ax=0 #homogenoussolution #solveamatrix
#Ax=0 #homogenoussolution #solveamatrix
Ax = 0, the Homogenous Solution
Homogeneous Systems: Given a Coefficient Matrix, Solve Ax=0
Solving Ax=0
Linear Algebra 1.5.1 Homogeneous System Solutions
Homogenous Linear Systems, Trivial and Nontrivial Solutions | Linear Algebra
Geometric View on Solutions to Ax=b and Ax=0.
Sec 2. 2 Proof that the solution of a homogeneous linear system os a subspace
Linear Algebra - How to Write Parametric Vector Form of a Homogeneous System of Equations, Ax = 0
solution of system of linear equations !! Homogeneous equations AX=0 , (Class - 1)
Linear Algebra: Homogeneous Systems
Linear Algebra - Lecture 10 - Homogeneous Linear Systems
Solution of Linear Homogeneous Equations AX = 0 || UNIQUE SOL & INFINITE SOLUTIONS
LA 1.5 Homogeneous System Ax=0
Three nice properties of homogeneous systems of linear equations
Linear Algebra - Lecture 11 - Non-Homogeneous Linear Systems
What is a homogeneous system in the matrix form ax 0?||Is the homogeneous system ax 0 consistent?
Homogeneous system of linear equations | Homogeneous system trivial solution | ax=0 unique solution
Homogeneous and Nonhomogeneous Solutions: Ax=0 and Ax=b
The set of all solutions to the homogeneous equation Ax = 0 where A is an 'm x n'matrix is...
Linear Algebra - Homogeneous Linear Systems
System of Homogenous and Non Homogenous equation (Part 1)
Dimension of Solution space of a homogeneous system AX=0 is n-rank of A- Proof and examples
Homogeneous and Particular Solution
Check if the homogeneous system Ax = 0 has a nontrivial solution. Is the matrix A nonsingular?
Комментарии
 0:03:03
0:03:03
 0:05:10
0:05:10
 0:10:04
0:10:04
 0:17:05
0:17:05
 0:09:57
0:09:57
 0:06:21
0:06:21
 0:08:01
0:08:01
 0:05:34
0:05:34
 0:16:14
0:16:14
 0:10:07
0:10:07
 0:08:54
0:08:54
 0:10:15
0:10:15
 0:09:33
0:09:33
 0:07:53
0:07:53
 0:10:58
0:10:58
 0:01:01
0:01:01
 0:05:59
0:05:59
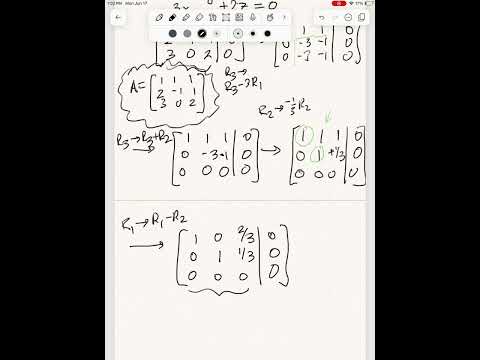 0:30:14
0:30:14
 0:00:54
0:00:54
 0:13:06
0:13:06
 0:35:18
0:35:18
 0:15:11
0:15:11
 0:15:37
0:15:37
 0:03:48
0:03:48