filmov
tv
Mechanics of Materials: Lesson 12 - Strain Energy; Example Problems From Stress Strain Diagram
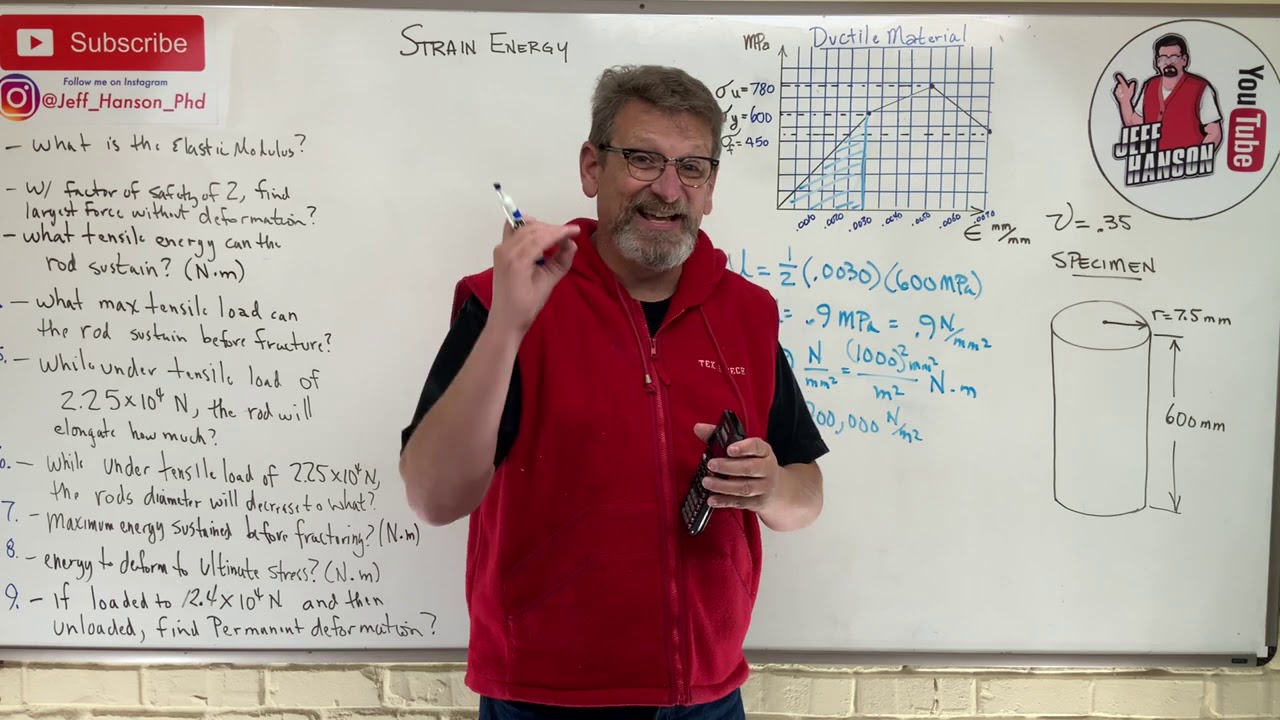
Показать описание
Top 15 Items Every Engineering Student Should Have!
This video is NOT sponsored. Some product links are affiliate links which mean if you buy something we'll receive a small commission.
Please consider supporting the channel by subscribing, commenting, and liking each video. This moves these videos up the search list and helps me greatly. Thank you for watching.
This video is copyrighted by the Jeff Hanson for the private use of our audience. Any other use of this video or any pictures, descriptions, or accounts of the video without written consent from Jeff Hanson is strictly prohibited.
Mechanics of Materials: Lesson 12 - Strain Energy; Example Problems From Stress Strain Diagram
Mechanics of Materials: Lesson 12 - Shear Stress-Strain Diagram
Mechanic of Materials: Lesson 24 -- Power Transmission Torque Example
Mechanics of Materials: Lesson 23 - Shear Stress Due to Torsion, Polar Moment of Inertia
Mechanics of Materials: Lesson 13 - Stress Strain Diagram Example Problem
Mechanics of Materials: Lesson 7 - Intro to Strain and Poisson’s Ratio
Mechanics of Materials: Lesson 25 - Angle of Twist Due to Torque, Torsion
Tensile Stress & Strain, Compressive Stress & Shear Stress - Basic Introduction
BEGINNER COURSE IN AUTOCAD MECHANICAL - EPISODE 12
Mechanics of Materials: Lesson 38 - Maximum Transverse Shear Stress in a Beam
Mechanics of Materials: Lesson 21 - Thermal Coefficient of Expansion, Axial Elongation
Mechanics of Materials: Lesson 18 - Axial Elongation Example Problem, Displacement
Mechanics of Materials: Lesson 17 - Axial Elongation Due to Axial Load Example
Mechanics of Materials: Lesson 11 - Modulus of Elasticity Example Problem
Elasticity & Hooke's Law - Intro to Young's Modulus, Stress & Strain, Elastic &...
Mechanics of Materials: Lesson 66 - Intro to Column Buckling
Mechanics of Materials: Lesson 37 - What the Heck is Q? Example Problem
Mechanics of Materials: Lesson 15 - Shear Stress Strain Diagram
Mechanics of Materials: Lesson 31 - The Flexure Formula, Beam Bending Example
Mechanics of Materials: Lesson 8 - Shear Strain Explained, Sign Convention
Mechanics of Materials: Lesson 62 - Slope and Deflection Beam Bending Introduction
Mechanics of Materials: Lesson 39 - Shear Flow Introduction, Example Problem
Understanding and Analysing Trusses
Mechanics of Materials: Lesson 9 - Stress Strain Diagram, Guaranteed for Exam 1!
Комментарии
 0:43:02
0:43:02
 0:08:06
0:08:06
 0:16:14
0:16:14
 0:17:01
0:17:01
 0:19:03
0:19:03
 0:16:02
0:16:02
 0:17:47
0:17:47
 0:13:05
0:13:05
 0:04:09
0:04:09
 0:17:06
0:17:06
 0:20:18
0:20:18
 0:15:41
0:15:41
 0:11:48
0:11:48
 0:18:48
0:18:48
 0:19:01
0:19:01
 0:20:06
0:20:06
 0:18:03
0:18:03
 0:08:30
0:08:30
 0:15:25
0:15:25
 0:15:38
0:15:38
 0:17:57
0:17:57
 0:12:13
0:12:13
 0:17:41
0:17:41
 0:22:41
0:22:41