Example of Determinant Using Row Echelon Form

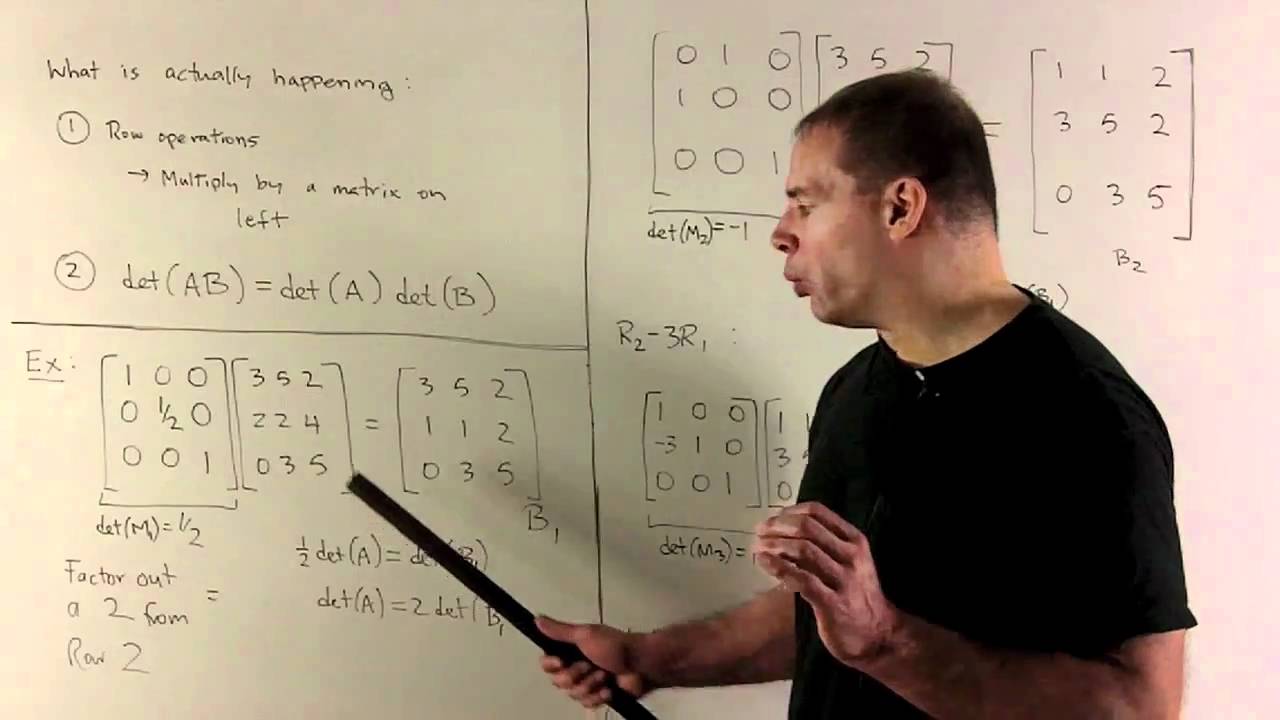
Linear Algebra: Find the determinant of the 3 x 3 matrix A = [ 3 5 2 \ 2 2 4 \ 0 3 5] by using row operations to put A in row echelon form. We review the effect of row operations on determinants.

[downscreen]

Wow, thanks Dr. Bob. I love your no BS approach to math and you explain it very clearly.

[green_pear_eater]

why are your explanations so easy to understand. ur, a huge help!!!

[bicboi]

Dude this is so good. Straight to the point of "how" using clear steps, then you give the conceptual basis, and it's all very organized and easy to understand.


So many professors and other youtubers make learning difficult material harder, just because of the poor communication. Either by trying to teaching it so dumbed down like (we have 3 bunnies here, how many carrots do we need) that you don't learn the concepts, you just learn by repetition, and therefore makes learning tedious and time consuming. Or making it seem so difficult, when in reality most things are simple ideas, that take many unintuitive steps to reach the goal.


P.S. I skipped from the middle to the end because you already found the determinant and was confused for a sec... Then I looked at the whiteboard and abra kadabra, plain as day on the whiteboard "what is actually happening". Simple things like the teacher being organized makes the learning process so much smoother!

[SentryTurretSpanky]

Your videos relieve so much stress when studying for exams! They're so direct and neatly presented.

[themightyquinn]

Awesome! Such a great explanation and proof! I wish my Linear Algebra professor would go in-depth like this.

[juliankanaskieking]

You make it so much simpler than my professor, thanks!

[korganrivera]

I've been using minors to calculate determinants and I hadn't thought to unravel those calculations; I didn't know the diagonals trick that you describe in the video. You've just helped me make my code SO much simpler. Thanks!

[lolno]

Dr. Bob you're amazing!!! This was clear and simple!!! Thank you sir!!!

[brent]

I'm not sure why there are comments saying people are scared haha.Your videos are awesome, good mix of theory and examples. Thanks heaps!

[MathDoctorBob]

@w00x91 You're welcome! Glad to be of help. - Bob

[cshalaby]

Thanks so much, I really appreciate the video. Your other videos have also been extremely helpful as well. You're such an amazing teacher!!

[MathDoctorBob]

@cshalaby87 You're welcome again! Definitely let me know if you have any requests.

[theennovators]

I felt like I am taking that class in a jail 😅 clear explaination

[MathDoctorBob]

Not necessarily. Just consider [1 2\ 1 3]. That goes to REF using one type 3 operation, but det=1.

[nonesta]

Let's say I have [0 0 -1] on R3 do I factor out the -1 to the determinant?

[劉信亨-ux]

in 1:55 if possible we can use "R1*3-R2 to-R2" ; why we use in "R2-3R1to-R2", please explain thanks

[MathDoctorBob]

You're welcome! If it dispels some stereotypes (in either direction), I'll take it. At the end of the day, it's about getting the math across.

[MathDoctorBob]

Getting your ass kicked hurts for a little while. Kicking your grade's ass hurts for life. :)

[davionsterling]

Doctor Bob at the 3:16 part of the video, how do you know that the two which is factor out is actually negative i understand everything else in the video but that section just confuses me a bit and i have an exam in the next two days where this is defineitely coming on it thank you very much

[aaliquegrahame]

If I reduce my matrix to row echelon form only using type 3 operations, do I conclude that my determinant is zero?