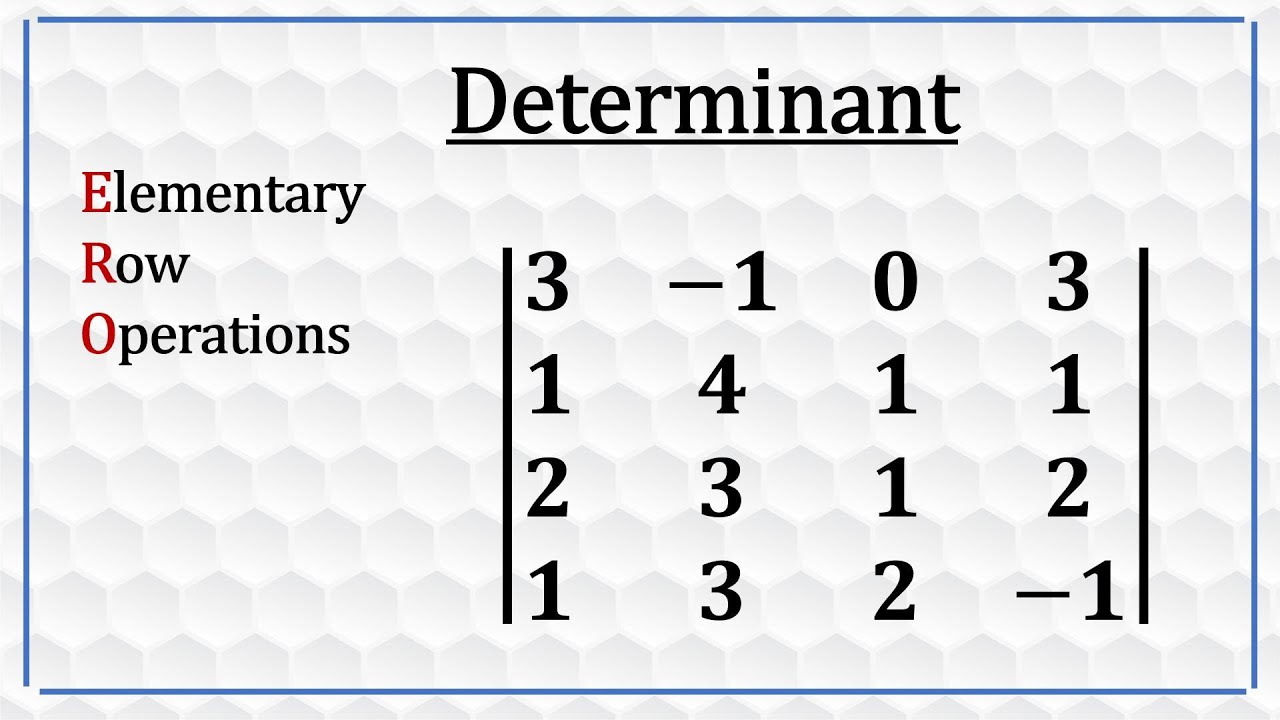
Determinant and Elementary Row Operation

In this video, I explained the benefit of having multiple zeros along a column or row in a matrix in order to compute the determinant. I also explained the effect of each of the elementary row operations on the value of the determinant

[hassanzaytoon]

I love your way of simplifying complex maths

[sammylee]

The Love ur writing, everything is so clear in this video

[ddgyt]

For me using the triangle rule on 3x3s is easier than using the rule of Sarrus because it doesn't involve writing any thing extra and usually can be easily computed in the head unless you have unusually large numbers. For all your videos they are very helpful.

[kpariwuurgilbertb]

Thanks for the wonderful job you have doing. So far so fantastic. Pls there's this prove of rank nullity theorem that I want to seek from you though I have seen several but I am not getting it. Thanks very much

[vibhavkumar]

Great Video. Quick question, when doing ERO #2, if you were to have a row (call it row A), and make row A = (K*row A) + (C*row B) or row A = (K*row A) - (C*row B), doesn't that multiply the determinant by K (C should have no effect)?

[Patricialupande-ybet]

Job well done, thanks for the wonderful explanation

[holyshit]

If we want to combine elementary row operations with cofactor expansion third column in this example is good for elimination because we need two row operations

[birb]

really great series don't putting out such informative content

[punditgi]

Nothing elementary about Prime Newtons! That is easily determined! 😊🎉

[kalumbualfredmandona]

the method for droping down cant it word for all matrices
from1x1 to nxn where any is any interger