filmov
tv
INCORRECT PROOF of Fermat's Last Theorem

Показать описание
before you bring a trampoline to this bowling tournament you should know that your cousin Archy Dyfunkle is the referee and dating your mother-in-law. There's a strict and I mean STRICT "NO TRAMPOLINES" allowed sign outside the combination Taco Bell-KFC-Bowling Alley. Okay folks sorry we're going to have to call off the butter chugging contest. if you watch this video till the end, a magic math moth may make mother moose milk made mischievously matching maroon Mariners merrily marching madly mooning magazine merchants.
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
INCORRECT PROOF of Fermat's Last Theorem
Proving Fermat' s Last Theorem (almost) in just 2 minutes !
Fermat's Last Theorem - Numberphile
Pingu reacting to proof of Fermat's Last Theorem
Proving weird Fermat's Last Theorem in just 2 minutes !
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
360 Years To Prove Fermat's Last Theorem | A Historic Breakthrough | #Shorts | Infinity Learn J...
The Man Who Solved the World’s Most Famous Math Problem
Fermat's Theorem (Part 4, Burton)
The Bridges to Fermat's Last Theorem - Numberphile
Mathematicians explains Fermat's Last Theorem | Edward Frenkel and Lex Fridman
Handsome Math Professor Pietro Boselli Explains Fermat's Last Theorem | GQ
Proof Converse of Fermat's Theorem Is False by producing a counterexample; Euler Totient functi...
Fermat's Last Theorem: A Very, Very Basic Proof
The paradox at the heart of mathematics: Gödel's Incompleteness Theorem - Marcus du Sautoy
Thirty years of proof: an interview with Andrew Wiles on the anniversary of Fermat's Last Theor...
Fermat's Last Theorem | Jordan Ellenberg and Lex Fridman
Andrew Wiles: Fermat's Last theorem: abelian and non-abelian approaches
How Was Fermat's Last Theorem Proved for Regular Primes?
Homer Simpson vs Pierre de Fermat - Numberphile
Fermat's Last Theorem; A Simple Proof 2017
Fermat's Last Theorem with Simon Singh
Fermat's Proof - Why You can never have a right triangle with a square area
Euler's and Fermat's last theorems, the Simpsons and CDC6600
Комментарии
 0:10:20
0:10:20
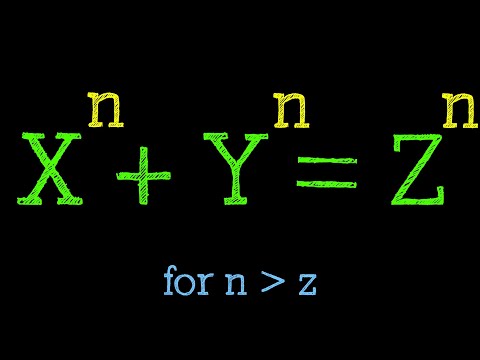 0:02:00
0:02:00
 0:09:31
0:09:31
 0:00:10
0:00:10
 0:02:00
0:02:00
 0:00:38
0:00:38
 0:00:47
0:00:47
 0:11:14
0:11:14
 0:23:51
0:23:51
 0:27:53
0:27:53
 0:15:14
0:15:14
 0:01:39
0:01:39
 0:08:06
0:08:06
 0:05:00
0:05:00
 0:05:20
0:05:20
 0:02:02
0:02:02
 0:17:24
0:17:24
 0:53:12
0:53:12
 0:17:05
0:17:05
 0:07:53
0:07:53
 0:01:02
0:01:02
 0:03:57
0:03:57
 0:01:14
0:01:14
 0:31:35
0:31:35