filmov
tv
Prove Algebraically That The Sum Of The Squares Of Any 2 Positive Even Integers Is A Multiple Of 4

Показать описание
To do this call the first even integer 2n and the second even integer 2n+2. If you square 2n you get 4n^2. And if you square 2n+2 you get 4n^2+8n+4. Now since sum means add then adding these up gives 8n^2+8n+4. Now to prove this is multiple of 4 factorise the expression putting 4 at the start of the bracket. This will give 4(n^2+2n+1).
 0:01:39
0:01:39
 0:03:50
0:03:50
 0:03:05
0:03:05
 0:02:16
0:02:16
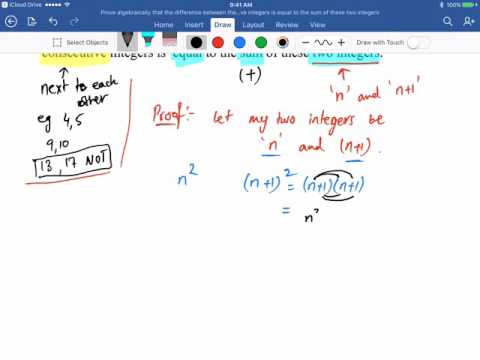 0:07:04
0:07:04
 0:02:17
0:02:17
 0:06:12
0:06:12
 0:23:21
0:23:21
 0:05:19
0:05:19
 0:14:48
0:14:48
 0:02:14
0:02:14
 0:00:48
0:00:48
 0:00:37
0:00:37
 0:00:50
0:00:50
 0:06:28
0:06:28
 0:00:23
0:00:23
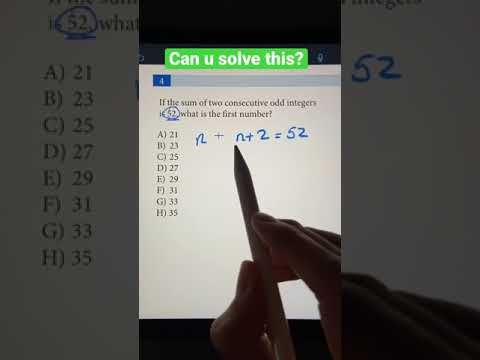 0:00:37
0:00:37
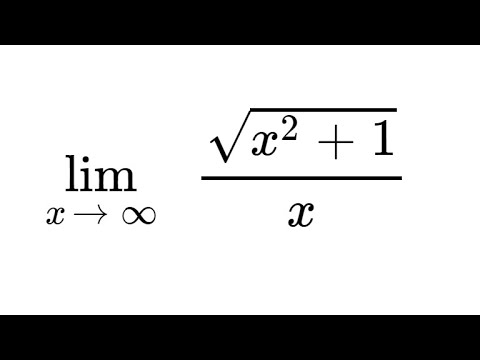 0:00:14
0:00:14
 0:02:32
0:02:32
 0:00:16
0:00:16
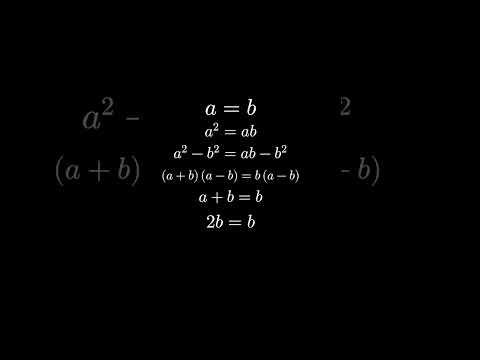 0:00:58
0:00:58
 0:05:28
0:05:28
 0:00:48
0:00:48
 0:02:37
0:02:37