filmov
tv
Computing Arc Length - Analytic Geometry and Calculus II | Lecture 12
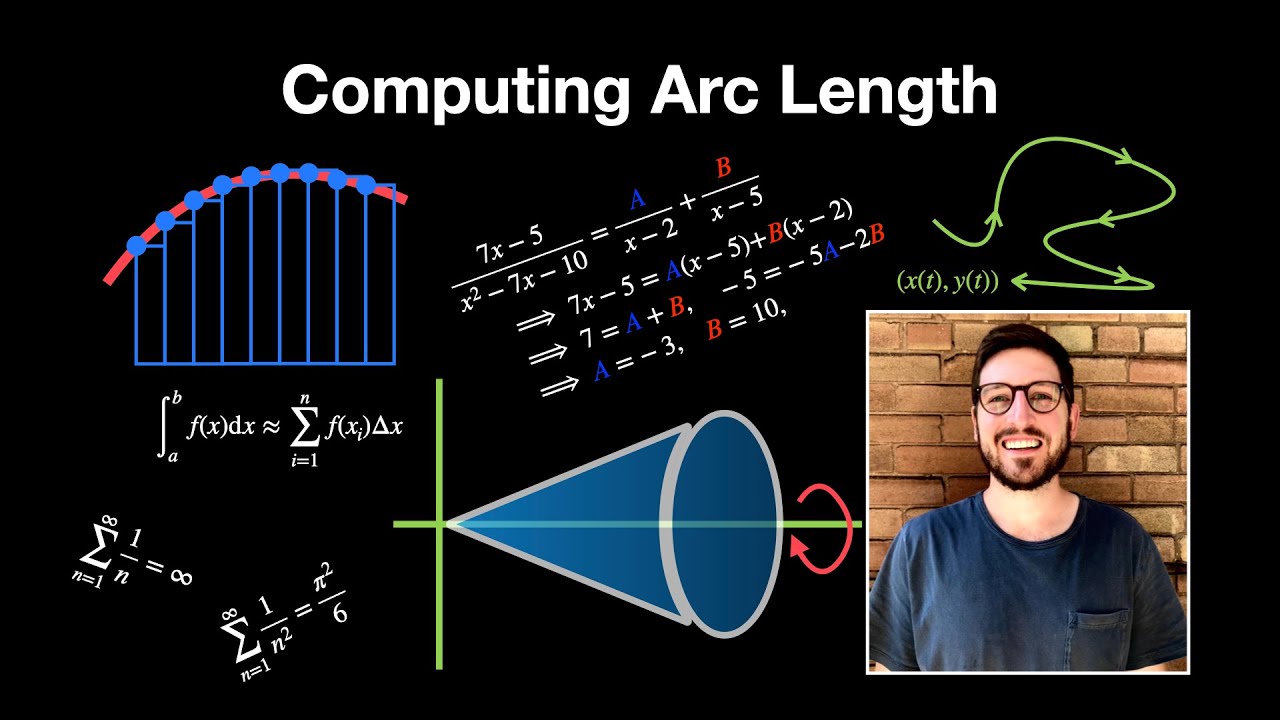
Показать описание
In this lecture we derive the formula for the length of a curve given by a smooth function. As in the previous lectures, we build up from discrete approximations of the curve to arrive at a Riemann sum. In an appropriate limit these Riemann sums converge to a definite integral whose value is exactly the length of the curve. Three examples are presented to elucidate the method.
This course is taught by Jason Bramburger for George Mason University.
Course Topics and Goals: At the end of the semester, students should be able to solve various geometry and physics problems that are modelled with definite integrals, use techniques to evaluate integrals, understand infinite series and power series, and be able to identify and graph conic sections and basic parameter and polar curves.
This course is taught by Jason Bramburger for George Mason University.
Course Topics and Goals: At the end of the semester, students should be able to solve various geometry and physics problems that are modelled with definite integrals, use techniques to evaluate integrals, understand infinite series and power series, and be able to identify and graph conic sections and basic parameter and polar curves.
 0:30:22
0:30:22
 0:07:57
0:07:57
 0:30:47
0:30:47
 0:12:09
0:12:09
 0:00:45
0:00:45
 0:06:33
0:06:33
 0:07:10
0:07:10
 0:03:24
0:03:24
 0:13:02
0:13:02
 0:15:57
0:15:57
 0:06:21
0:06:21
 0:22:42
0:22:42
 0:04:38
0:04:38
 0:07:44
0:07:44
 0:03:37
0:03:37
 0:25:40
0:25:40
 0:00:53
0:00:53
 0:00:51
0:00:51
 0:07:43
0:07:43
 0:31:09
0:31:09
 0:06:00
0:06:00
 0:08:01
0:08:01
 0:00:14
0:00:14
 1:03:26
1:03:26