filmov
tv
Implicit Differentiation with e^x
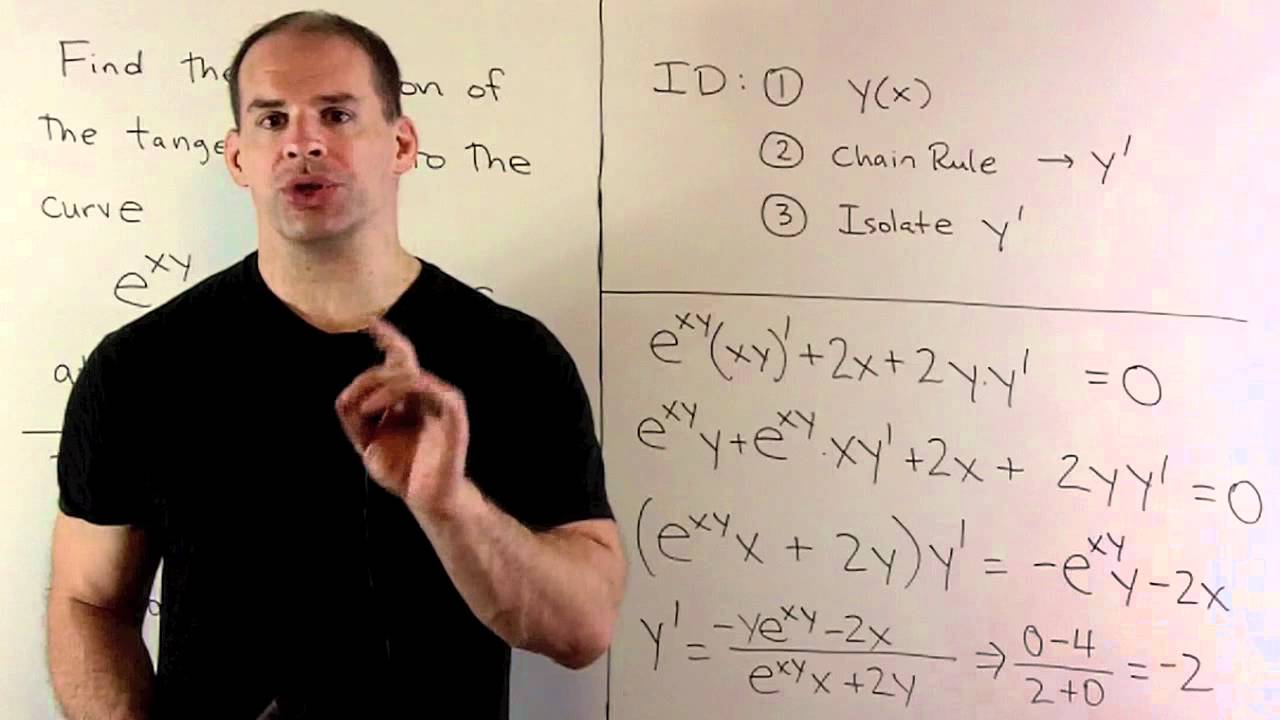
Показать описание
Calculus: Consider the curve defined by exp(xy) + x^2 + y^2 = 5. Use implicit differentiation to find the tangent line to the curve at (2, 0). Then use the tangent line to approximate the point near (2, 0) with x = 2.01.
Derivative of e^xy (Implicit Differentiation) | Calculus 1 Exercises
Implicit Differentiation with e^y ❖ Calculus 1
Implicit Differentiation e ^ x+y = 1 + x^2 y^2
Implicit Differentiation with e^x
Implicit Differentiation with Exponential Functions | Calculus 1 Exercises
Implicit Differentiation
Ex 1: Implicit Differentiation
Implicit differentiation with the chain rule and in
Derivatives of Exponential Functions
Why is the derivative of e^x equal to e^x?
FIND dy/dx BY IMPLICIT DIFFERENTIATION | e^(x^2y) = x + y
using implicit differentiation to find dy/dx for e^(x/y)=x-y
Implicit Derivative of Exponential Function xe^y - y^3e^x = 0 with Chain Rule IBSL AP Calculus
e^(x^2*y) = x + y, Find dy/dx by implicit differentiation
derivative for e^(x/y) = x - y, calculus 1 tutorial
Derivatives of Logarithmic and Exponential Functions
Derivative of e^x Proof (Using Implicit Differentiation)
implicit differentiation ex
Proof: Derivative of e^x is e^x
Ex 3: Implicit Differentiation Using the Product Rule and Factoring
Implicit Differentiation - with e
Ex 2: Implicit Differentiation Using the Product Rule
Derivative of e^2x (Chain Rule) | Calculus 1 Exercises
Implicit derivative of e^(xy^2) = x - y
Комментарии
 0:03:37
0:03:37
 0:03:26
0:03:26
 0:04:54
0:04:54
 0:07:01
0:07:01
 0:13:01
0:13:01
 0:14:34
0:14:34
 0:03:25
0:03:25
 0:05:25
0:05:25
 0:12:03
0:12:03
 0:11:59
0:11:59
 0:09:04
0:09:04
 0:03:33
0:03:33
 0:05:23
0:05:23
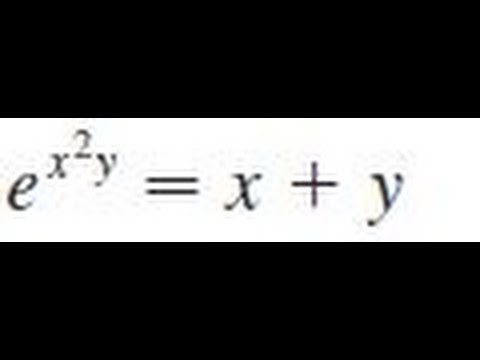 0:02:42
0:02:42
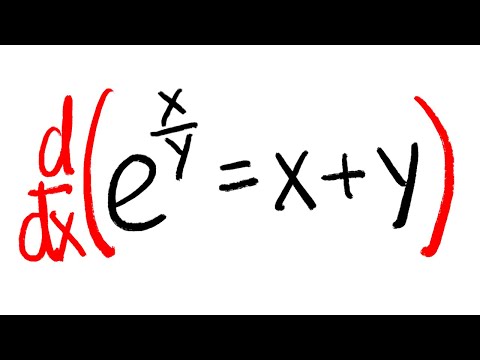 0:05:24
0:05:24
 0:08:41
0:08:41
 0:03:02
0:03:02
 0:06:18
0:06:18
 0:10:24
0:10:24
 0:05:40
0:05:40
 0:09:34
0:09:34
 0:04:02
0:04:02
 0:00:50
0:00:50
 0:05:58
0:05:58