filmov
tv
Solving absolute value inequalities when there are infinite many solutions
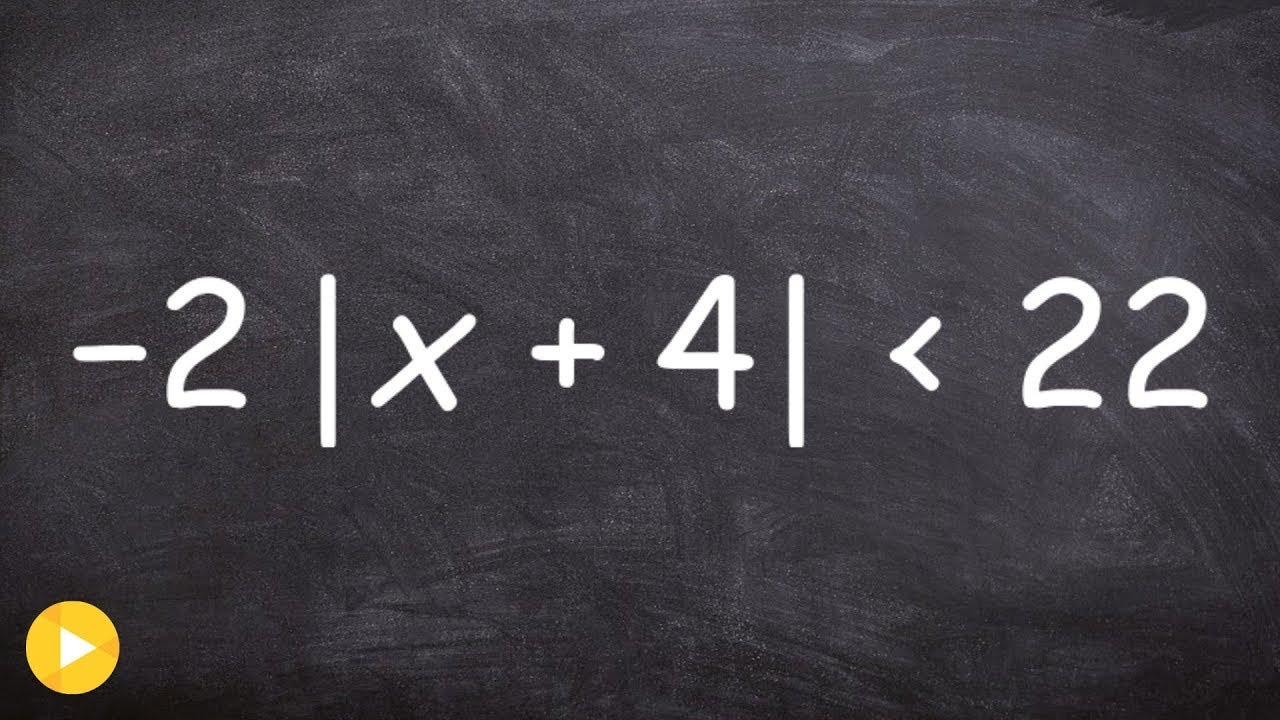
Показать описание
👉 Learn how to solve multi-step absolute value inequalities. The absolute value of a number is the positive value of the number. For instance, the absolute value of 2 is 2 and the absolute value of -2 is also 2. To solve an absolute value inequality where there are more terms apart from the absolute value term in the same side of the inequality sign as the absolute value term, we first isolate the absolute value term. i.e. make the absolute value term the subject of the formula.
After isolating the absolute value term, we then create the two cases of absolute value problems: the positive case and the positive case. For the negative case, we flip the inequality sign. This results in a compound inequality which we then solve accordingly and graph our solution on a number line.
Organized Videos:
✅Solve Absolute Value Inequalities
✅Solve Absolute Value Inequalities | Easy
✅Solve Absolute Value Inequalities | Medium
✅Solve Absolute Value Inequalities | Hard
Connect with me:
#AbsoluteValue #Brianmclogan
After isolating the absolute value term, we then create the two cases of absolute value problems: the positive case and the positive case. For the negative case, we flip the inequality sign. This results in a compound inequality which we then solve accordingly and graph our solution on a number line.
Organized Videos:
✅Solve Absolute Value Inequalities
✅Solve Absolute Value Inequalities | Easy
✅Solve Absolute Value Inequalities | Medium
✅Solve Absolute Value Inequalities | Hard
Connect with me:
#AbsoluteValue #Brianmclogan
Absolute Value Inequalities - How To Solve It
How To Solve Absolute Value Inequalities, Basic Introduction, Algebra
Absolute value inequalities | Linear equations | Algebra I | Khan Academy
17 - Solving & Graphing Absolute Value Inequalities in Algebra, Part 1
Absolute Value Inequalities - How to Solve
Learn how to solve absolute value inequalites by creating compound inequalities
ABSOLUTE VALUE INEQUALITIES with ZERO/NEGATIVES on one side
Solving Equations w/ Absolute Values Algebraically ('By Cases')
J.E.D.I. Training MATH for DIGITAL SAT - Day 6 of 7 (37 Digital SAT Math Problems EXPLAINED)
How to solve a one variable absolute value inequality or statement
Solve absolute values inequalities practice
How To Solve Absolute Value Equations, Basic Introduction, Algebra
Solving absolute value inequalities when there are infinite many solutions
Absolute Value Inequality Example: When both sides have modulus
Solving an absolute value inequality by switching the signs
Solving Inequalities with Absolute Values: Ex |x-2| less than 3
Solve absolute rational inequalities
Solving an Absolute Value Equation
Solving Advanced Absolute Value Inequalities Practice Test Full Solutions
Ex 6: Solve and Graph Absolute Value inequalities (Requires Isolating Abs. Value)
Solving a Basic Absolute Value Inequality
Solving Inequalities with Two Absolute Values by Testing Points
Solving Absolute Value Inequalities (And vs Or)
Absolute Value Equations & Inequalities (1 of 4: Visualising an equation)
Комментарии
 0:12:40
0:12:40
 0:03:56
0:03:56
 0:13:13
0:13:13
 0:23:30
0:23:30
 0:05:14
0:05:14
 0:10:50
0:10:50
 0:13:42
0:13:42
 0:09:42
0:09:42
 1:04:45
1:04:45
 0:04:48
0:04:48
 0:14:17
0:14:17
 0:04:21
0:04:21
 0:04:28
0:04:28
 0:05:30
0:05:30
 0:02:30
0:02:30
 0:04:34
0:04:34
 0:08:14
0:08:14
 0:05:00
0:05:00
 1:26:24
1:26:24
 0:03:37
0:03:37
 0:08:51
0:08:51
 0:11:17
0:11:17
 0:10:32
0:10:32
 0:10:07
0:10:07