filmov
tv
Mastering Complex Numbers: Solving Trigonometric Equations with Complex Arithmetic - AIME 2008

Показать описание
Welcome to another exciting installment of our 'Mastering Complex Numbers' series. In this episode, we dive into a captivating problem from the 2008 AIME I - a challenge that blends trigonometry and complex numbers in a fascinating way.
We tackle the problem of finding a positive integer 'n' such that the sum of arctan(1/3), arctan(1/4), arctan(1/5), and arctan(1/n) equals pi/4. This problem provides a wonderful opportunity to explore the intersection of complex numbers and trigonometric identities.
Rather than going down the usual trigonometric route, we explore an ingenious approach involving complex numbers - specifically, the multiplication of complex numbers (3+i), (4+i), (5+i), and (n+i) and the angles these numbers form with the real axis.
Follow along as we unveil the beauty of complex number arithmetic and apply it to solve this intriguing AIME problem. Whether you're preparing for math competitions or just a math enthusiast, this exploration into complex numbers and trigonometric equations promises to be a captivating and educational adventure. Let's master complex numbers together!
We tackle the problem of finding a positive integer 'n' such that the sum of arctan(1/3), arctan(1/4), arctan(1/5), and arctan(1/n) equals pi/4. This problem provides a wonderful opportunity to explore the intersection of complex numbers and trigonometric identities.
Rather than going down the usual trigonometric route, we explore an ingenious approach involving complex numbers - specifically, the multiplication of complex numbers (3+i), (4+i), (5+i), and (n+i) and the angles these numbers form with the real axis.
Follow along as we unveil the beauty of complex number arithmetic and apply it to solve this intriguing AIME problem. Whether you're preparing for math competitions or just a math enthusiast, this exploration into complex numbers and trigonometric equations promises to be a captivating and educational adventure. Let's master complex numbers together!
 0:05:41
0:05:41
 0:27:51
0:27:51
 0:03:22
0:03:22
 0:00:58
0:00:58
 0:10:28
0:10:28
 0:05:17
0:05:17
 0:00:59
0:00:59
 0:27:57
0:27:57
 0:05:02
0:05:02
 0:07:37
0:07:37
 0:05:47
0:05:47
 0:00:25
0:00:25
 0:00:48
0:00:48
 0:02:44
0:02:44
 0:10:17
0:10:17
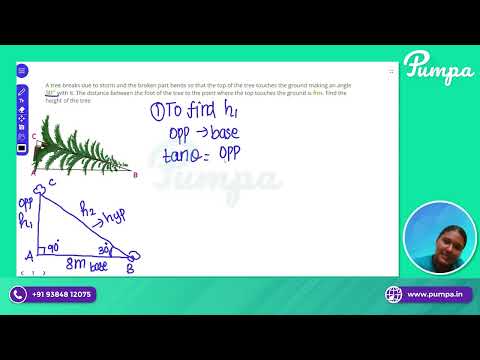 0:15:17
0:15:17
 0:32:33
0:32:33
 0:00:33
0:00:33
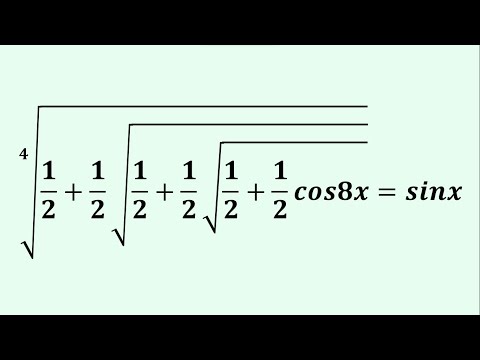 0:11:14
0:11:14
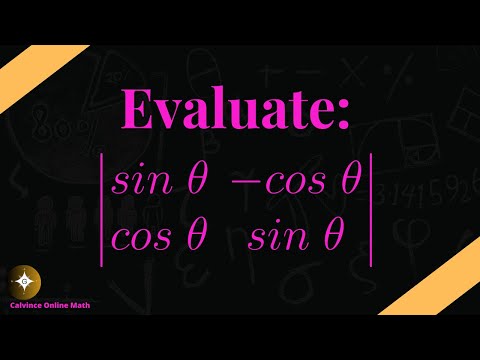 0:01:24
0:01:24
 0:00:29
0:00:29
 0:12:49
0:12:49
 0:05:48
0:05:48
 1:03:43
1:03:43